Comparing and ordering numbers are fundamental mathematical skills. COMPARE.EDU.VN clarifies the distinction between these two concepts. This guide simplifies number comparison and arrangement for students, consumers, and professionals, ensuring a clear understanding and application of numerical relationships and sequencing. Explore the nuances of numerical evaluation and sequencing to enhance decision-making and analytical capabilities.
1. Understanding the Basics: Comparing Numbers
Comparing numbers involves evaluating two or more numbers to determine their relative size or value. This process helps identify whether one number is greater than, less than, or equal to another. The essence of comparing numbers lies in establishing a quantitative relationship between them.
1.1. Defining Comparison
Comparison, in mathematical terms, refers to the act of examining the characteristics of numbers to establish their relationship. This relationship is typically categorized into three possibilities:
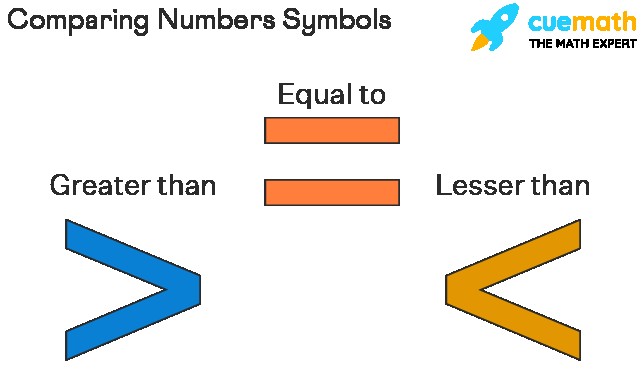
- Greater Than: One number holds a higher value than the other.
- Less Than: One number holds a lower value than the other.
- Equal To: Both numbers possess the same value.
Understanding these relationships is crucial for making informed decisions in various real-life scenarios, from managing finances to analyzing data.
1.2. Methods for Comparing Numbers
Several methods can be employed to effectively compare numbers, each catering to different types of numbers and levels of complexity.
- Number Line: A visual tool where numbers are positioned according to their value, facilitating easy comparison. Numbers to the right are greater, and those to the left are smaller.
- Counting: For natural numbers, counting can be a straightforward method. The number reached later in the counting sequence is the larger one.
- Digit Counting: When comparing whole numbers, the number with more digits is generally larger.
- Place Value: Compare the digits in each place value, starting from the leftmost digit. This method is particularly useful for comparing larger numbers.
1.3. Practical Applications of Number Comparison
The ability to compare numbers is integral to numerous aspects of daily life.
- Financial Management: Comparing prices to find the best deals.
- Cooking: Adjusting recipe quantities based on the number of servings.
- Travel: Assessing distances and travel times for route planning.
- Education: Evaluating test scores to gauge performance.
1.4. Common Pitfalls in Comparing Numbers
While comparing numbers seems straightforward, certain pitfalls can lead to errors.
- Ignoring Negative Signs: Negative numbers are smaller than positive numbers, and larger negative numbers are smaller than smaller negative numbers (e.g., -5 < -2).
- Misinterpreting Decimals: Ensure accurate alignment of decimal points when comparing decimal numbers.
- Overlooking Units: Always consider the units of measurement to avoid comparing dissimilar quantities.
2. Ordering Numbers: Arranging in Sequence
Ordering numbers goes beyond simple comparison, involving the arrangement of a set of numbers in a specific sequence based on their values. This sequence can be either ascending (from smallest to largest) or descending (from largest to smallest).
2.1. Defining Ordering
Ordering, in the context of numbers, is the process of arranging a collection of numbers in a meaningful sequence. This sequence adheres to a specific criterion, primarily ascending or descending order. The act of ordering provides a structured view of the numerical data, making it easier to interpret and analyze.
2.2. Ascending vs. Descending Order
The two primary methods of ordering numbers each serve different purposes.
- Ascending Order: Arranges numbers from the smallest value to the largest value. This is commonly used to organize data in a progressive manner. For example: 1, 2, 3, 4, 5.
- Descending Order: Arranges numbers from the largest value to the smallest value. This is often used to prioritize data or highlight the most significant values. For example: 5, 4, 3, 2, 1.
2.3. Steps for Ordering Numbers
To accurately order numbers, follow these steps:
- Compare: Begin by comparing each pair of numbers in the set to determine their relative values.
- Identify Extremes: Locate the smallest and largest numbers in the set.
- Arrange: Place the numbers in the desired order, either ascending or descending, based on the comparison results.
- Verify: Review the ordered sequence to ensure accuracy and consistency.
2.4. Practical Applications of Number Ordering
Ordering numbers is indispensable in numerous real-world applications.
- Data Analysis: Arranging data sets to identify trends and patterns.
- Inventory Management: Ordering products based on sales performance.
- Project Management: Sequencing tasks based on priority and deadlines.
- Academic Grading: Ranking students based on their scores.
2.5. Common Challenges in Ordering Numbers
Several challenges can arise when ordering numbers, especially with more complex data sets.
- Large Datasets: Ordering a large number of values can be time-consuming and prone to error.
- Negative Numbers: Correctly placing negative numbers requires careful attention to their values relative to zero.
- Decimals and Fractions: Converting decimals and fractions to a common format can simplify the ordering process.
- Mixed Data Types: Handling datasets with a mix of integers, decimals, and fractions requires consistent conversion and comparison methods.
3. Key Differences Between Comparing and Ordering
While both comparing and ordering numbers deal with numerical values, their purposes and processes differ significantly.
3.1. Purpose and Objective
- Comparing: The primary goal is to determine the relationship between two or more numbers—whether they are equal, one is greater than the other, or one is less than the other.
- Ordering: The main objective is to arrange a set of numbers in a specific sequence, either ascending or descending, based on their values.
3.2. Process and Methodology
- Comparing: This involves a pairwise evaluation of numbers, focusing on identifying their relative magnitude.
- Ordering: This requires a systematic arrangement of a collection of numbers, building upon individual comparisons to establish a complete sequence.
3.3. Outcome and Application
- Comparing: The result is a statement of relationship (e.g., A > B, A = B, A < B), which is used to make decisions or draw conclusions based on the relative values.
- Ordering: The outcome is a sequenced list of numbers that facilitates analysis, prioritization, or organization of data.
3.4. Complexity
- Comparing: Generally simpler, as it involves direct pairwise comparisons.
- Ordering: Can be more complex, especially with large datasets or mixed data types, requiring careful attention to detail and systematic application of comparison principles.
4. Symbols Used in Comparing Numbers
Symbols play a crucial role in the comparison of numbers, providing a shorthand notation to express the relationships between numerical values.
4.1. Greater Than (>)
The “greater than” symbol (>) indicates that the number on the left side of the symbol has a higher value than the number on the right side.
- Example: 5 > 3 (5 is greater than 3)
- Usage: This symbol is used to express that one quantity is larger or more significant than another.
4.2. Less Than (<)
The “less than” symbol (<) signifies that the number on the left side of the symbol has a lower value than the number on the right side.
- Example: 2 < 9 (2 is less than 9)
- Usage: This symbol is used to express that one quantity is smaller or less significant than another.
4.3. Equal To (=)
The “equal to” symbol (=) indicates that the numbers on both sides of the symbol have the same value.
- Example: 4 = 4 (4 is equal to 4)
- Usage: This symbol is used to express that two quantities are identical in value.
4.4. Not Equal To (≠)
The “not equal to” symbol (≠) indicates that the numbers on both sides of the symbol do not have the same value.
- Example: 7 ≠ 10 (7 is not equal to 10)
- Usage: This symbol is used to express that two quantities are different in value.
4.5. Greater Than or Equal To (≥)
The “greater than or equal to” symbol (≥) indicates that the number on the left side of the symbol has a value that is either higher than or equal to the number on the right side.
- Example: 6 ≥ 6 (6 is greater than or equal to 6)
- Usage: This symbol is used to express that one quantity is at least as large as another.
4.6. Less Than or Equal To (≤)
The “less than or equal to” symbol (≤) signifies that the number on the left side of the symbol has a value that is either lower than or equal to the number on the right side.
- Example: 1 ≤ 8 (1 is less than or equal to 8)
- Usage: This symbol is used to express that one quantity is no larger than another.
5. Comparing Rational Numbers
Rational numbers, which can be expressed as a fraction p/q where p and q are integers and q ≠ 0, require a systematic approach for comparison.
5.1. Converting to Like Fractions
To compare rational numbers, convert them to like fractions, ensuring they have a common denominator. This is typically achieved by finding the least common multiple (LCM) of the denominators.
- Example: Compare 2/3 and 3/4. The LCM of 3 and 4 is 12.
- 2/3 = (2 × 4) / (3 × 4) = 8/12
- 3/4 = (3 × 3) / (4 × 3) = 9/12
5.2. Comparing Numerators
Once the rational numbers have a common denominator, compare their numerators. The rational number with the larger numerator is the greater number.
- Example: Comparing 8/12 and 9/12.
- Since 9 > 8, then 9/12 > 8/12.
- Therefore, 3/4 > 2/3.
5.3. Rules for Comparing Rational Numbers
- Positive vs. Negative: All positive rational numbers are greater than all negative rational numbers.
- Zero: All positive rational numbers are greater than 0, and all negative rational numbers are less than 0.
- Negative Rational Numbers: When comparing negative rational numbers, the one with the smaller absolute value is greater. For example, -1/4 > -1/2 because |-1/4| < |-1/2|.
5.4. Decimal Representation
Convert rational numbers to their decimal representation for easier comparison, especially when dealing with complex fractions.
- Example: Compare 5/8 and 7/10.
- 5/8 = 0.625
- 7/10 = 0.7
- Since 0.7 > 0.625, then 7/10 > 5/8.
6. Advanced Techniques for Number Manipulation
Mastering number comparison and ordering involves understanding advanced techniques applicable in complex scenarios.
6.1. Logarithmic Comparison
Logarithms can simplify the comparison of very large or very small numbers by compressing their scale.
- Application: Useful in scientific notation and exponential functions.
- Technique: Compare the logarithms of the numbers. If log(A) > log(B), then A > B.
6.2. Percentage Difference
Quantifying the difference between numbers as a percentage provides a relative measure that is useful for understanding proportional changes.
- Formula: Percentage Difference = |(A – B) / ((A + B) / 2)| × 100
- Interpretation: A higher percentage difference indicates a more significant disparity between the numbers.
6.3. Indexing and Normalization
Indexing involves scaling numbers relative to a base value, while normalization scales them to fit within a specific range, such as 0 to 1.
- Application: Useful for comparing data points from different datasets with varying scales.
- Technique: Normalize the data to a common scale and then compare the normalized values.
6.4. Statistical Comparison
Statistical measures, such as mean, median, and standard deviation, can be used to compare datasets rather than individual numbers.
- Application: Useful in data analysis and research.
- Technique: Compare the statistical measures of the datasets to draw inferences about their overall characteristics.
7. Common Mistakes and How to Avoid Them
Even with a solid understanding of comparing and ordering numbers, mistakes can occur. Recognizing common errors and implementing strategies to avoid them is essential for accuracy.
7.1. Misunderstanding Negative Numbers
- Mistake: Assuming that larger negative numbers are greater than smaller negative numbers.
- Solution: Remember that negative numbers decrease in value as their absolute value increases (e.g., -5 < -2).
7.2. Incorrect Decimal Alignment
- Mistake: Failing to align decimal points when comparing decimal numbers.
- Solution: Always align decimal points to ensure accurate comparison of place values.
7.3. Ignoring Units of Measurement
- Mistake: Comparing numbers without considering their units of measurement.
- Solution: Ensure that all numbers are in the same units before comparing them.
7.4. Overlooking Order of Operations
- Mistake: Neglecting the correct order of operations when comparing expressions.
- Solution: Follow the order of operations (PEMDAS/BODMAS) to evaluate expressions accurately before comparing.
7.5. Confusing Ascending and Descending Order
- Mistake: Arranging numbers in the wrong order.
- Solution: Double-check whether you need to arrange numbers from smallest to largest (ascending) or largest to smallest (descending).
8. Practical Examples of Comparing and Ordering Numbers
To solidify understanding, let’s explore practical examples of how comparing and ordering numbers are applied in different scenarios.
8.1. Example 1: Financial Planning
- Scenario: Comparing interest rates on savings accounts to maximize returns.
- Data:
- Account A: 2.5% interest
- Account B: 2.75% interest
- Account C: 2.6% interest
- Solution:
- Compare: 2.75% > 2.6% > 2.5%
- Order (Descending): 2.75%, 2.6%, 2.5%
- Conclusion: Account B offers the highest interest rate.
8.2. Example 2: Inventory Management
- Scenario: Ordering products based on sales performance to optimize stocking levels.
- Data:
- Product X: 150 units sold
- Product Y: 120 units sold
- Product Z: 180 units sold
- Solution:
- Compare: 180 > 150 > 120
- Order (Descending): 180, 150, 120
- Conclusion: Product Z should be stocked in the highest quantity.
8.3. Example 3: Project Management
- Scenario: Sequencing tasks based on priority and deadlines.
- Data:
- Task A: Deadline in 10 days, Priority 3
- Task B: Deadline in 5 days, Priority 1
- Task C: Deadline in 7 days, Priority 2
- Solution:
- Order (Priority – Ascending): 1, 2, 3
- Order (Deadline – Ascending): 5, 7, 10
- Conclusion: Task B should be addressed first due to its high priority and near deadline.
8.4. Example 4: Academic Grading
- Scenario: Ranking students based on their scores in an exam.
- Data:
- Student 1: 85 points
- Student 2: 92 points
- Student 3: 78 points
- Solution:
- Compare: 92 > 85 > 78
- Order (Descending): 92, 85, 78
- Conclusion: Student 2 is ranked first, followed by Student 1, and then Student 3.
9. The Role of COMPARE.EDU.VN in Mastering Number Skills
COMPARE.EDU.VN provides an invaluable resource for mastering number comparison and ordering skills. By offering comprehensive guides, practical examples, and advanced techniques, the website empowers individuals to make informed decisions and enhance their analytical capabilities.
9.1. Comprehensive Guides
COMPARE.EDU.VN offers detailed guides that cover the fundamentals of comparing and ordering numbers, including step-by-step instructions and clear explanations.
9.2. Practical Examples
The website provides numerous real-world examples that illustrate how these skills are applied in various contexts, from financial planning to project management.
9.3. Advanced Techniques
COMPARE.EDU.VN explores advanced techniques such as logarithmic comparison, percentage difference, and statistical comparison, catering to users seeking to deepen their understanding.
9.4. Error Prevention
The platform identifies common mistakes and offers practical solutions to avoid them, ensuring accuracy and efficiency in number manipulation.
9.5. Interactive Tools
COMPARE.EDU.VN provides interactive tools and resources that allow users to practice and reinforce their skills in a dynamic and engaging manner.
10. Conclusion: Empowering Decision-Making Through Numerical Proficiency
Understanding the difference between comparing and ordering numbers is crucial for effective decision-making and analytical capabilities. Comparing numbers involves evaluating their relative size or value, while ordering numbers entails arranging a set of numbers in a specific sequence based on their values. By mastering these skills, individuals can enhance their ability to interpret data, manage resources, and make informed choices in various aspects of life.
COMPARE.EDU.VN stands as a valuable resource for anyone seeking to improve their numerical proficiency. Through comprehensive guides, practical examples, and advanced techniques, the website empowers users to confidently navigate complex numerical scenarios and make data-driven decisions.
Are you ready to elevate your numerical skills and make more informed decisions? Visit COMPARE.EDU.VN today to explore our comprehensive resources and unlock your full potential.
Address: 333 Comparison Plaza, Choice City, CA 90210, United States
WhatsApp: +1 (626) 555-9090
Website: COMPARE.EDU.VN
11. FAQs on Comparing and Ordering Numbers
11.1. What is the difference between comparing and ordering numbers?
Comparing numbers involves evaluating their relative size or value, while ordering numbers entails arranging a set of numbers in a specific sequence based on their values.
11.2. Why is comparing numbers important in real life?
Comparing numbers is essential for making informed decisions in various real-life scenarios, such as financial management, cooking, travel, and education.
11.3. What are the symbols used for comparing numbers?
The symbols used for comparing numbers include greater than (>), less than (<), equal to (=), not equal to (≠), greater than or equal to (≥), and less than or equal to (≤).
11.4. How do you compare rational numbers?
To compare rational numbers, convert them to like fractions with a common denominator, compare their numerators, and apply the rules for comparing positive, negative, and zero values.
11.5. What are some common mistakes to avoid when comparing numbers?
Common mistakes include misunderstanding negative numbers, incorrect decimal alignment, ignoring units of measurement, overlooking the order of operations, and confusing ascending and descending order.
11.6. How can logarithms simplify the comparison of numbers?
Logarithms can simplify the comparison of very large or very small numbers by compressing their scale, making it easier to evaluate their relative magnitudes.
11.7. What is percentage difference, and how is it calculated?
Percentage difference quantifies the difference between numbers as a percentage, providing a relative measure of their disparity. It is calculated using the formula: |(A – B) / ((A + B) / 2)| × 100.
11.8. What is indexing and normalization, and how are they used in number comparison?
Indexing involves scaling numbers relative to a base value, while normalization scales them to fit within a specific range, such as 0 to 1. These techniques are useful for comparing data points from different datasets with varying scales.
11.9. How can statistical measures be used to compare datasets?
Statistical measures, such as mean, median, and standard deviation, can be used to compare datasets by providing insights into their overall characteristics and distributions.
11.10. How does COMPARE.EDU.VN help in mastering number skills?
compare.edu.vn provides comprehensive guides, practical examples, advanced techniques, and interactive tools to empower users to master number comparison and ordering skills and make informed decisions.