**What Does Compare Fractions Mean? A Comprehensive Guide**

What Does Compare Fractions Mean? Discover effective strategies for fraction comparison, including same denominators, unlike denominators, and visualization techniques. At COMPARE.EDU.VN, we provide a detailed exploration to simplify this mathematical concept. Master comparing fractions with ease and enhance your numerical skills.

1. Introduction to Comparing Fractions

Comparing fractions is a fundamental concept in mathematics that involves determining the relative size or value of two or more fractions. This process helps us understand which fraction represents a larger or smaller portion of a whole. In our daily lives, comparing fractions is essential for various tasks, such as following recipes, managing finances, or understanding data. By mastering the techniques of comparing fractions, we can make informed decisions and solve practical problems more effectively.

Fractions, composed of a numerator and a denominator, require specific methods for accurate comparison. Whether dealing with fractions with the same denominator or different denominators, understanding the principles outlined here will allow you to confidently compare and interpret fractional values. Let’s delve deeper into the methods and strategies for comparing fractions effectively.

2. Understanding the Basics: What is a Fraction?

Before diving into the methods of comparing fractions, it’s important to have a clear understanding of what a fraction is. A fraction represents a part of a whole and is expressed as a ratio of two numbers: the numerator and the denominator. The numerator indicates the number of parts we have, while the denominator indicates the total number of equal parts the whole is divided into.

For example, in the fraction 3/4, the numerator is 3 and the denominator is 4. This means we have 3 parts out of a total of 4 equal parts. Understanding this basic concept is essential for accurately comparing fractions and interpreting their values.

2.1 Numerator and Denominator

• Numerator: The number above the fraction bar, indicating the number of parts we have.
• Denominator: The number below the fraction bar, indicating the total number of equal parts the whole is divided into.

Understanding these components is crucial for comparing fractions effectively. The relationship between the numerator and denominator determines the value of the fraction and how it relates to other fractions.

2.2 Types of Fractions

There are several types of fractions, each with its unique characteristics. Understanding these types can aid in comparing fractions more effectively:

• Proper Fractions: The numerator is less than the denominator (e.g., 2/5).
• Improper Fractions: The numerator is greater than or equal to the denominator (e.g., 7/3).
• Mixed Numbers: A whole number combined with a proper fraction (e.g., 2 1/4).

Knowing the type of fraction can guide the comparison process, as improper fractions and mixed numbers may need to be converted to proper fractions for easier comparison.

3. Comparing Fractions with the Same Denominators

Comparing fractions with the same denominators is the simplest scenario. When fractions share a common denominator, the fraction with the larger numerator is the larger fraction. This is because the denominator indicates the size of each part, and if the denominators are the same, the fraction with more parts (larger numerator) represents a greater portion of the whole.

For example, if we want to compare 3/7 and 5/7, since both fractions have the same denominator (7), we can simply compare their numerators. Since 5 is greater than 3, we can conclude that 5/7 is greater than 3/7.

3.1 Step-by-Step Guide

1. Check the Denominators: Ensure that the fractions you are comparing have the same denominator.
2. Compare the Numerators: Identify the numerator of each fraction.
3. Determine the Larger Fraction: The fraction with the larger numerator is the larger fraction.

3.2 Examples and Illustrations

Let’s illustrate this with a few examples:

• Example 1: Compare 2/9 and 7/9.

  • Both fractions have the same denominator (9).
  • Compare the numerators: 7 > 2.
  • Therefore, 7/9 > 2/9.

• Example 2: Compare 4/11 and 1/11.

  • Both fractions have the same denominator (11).
  • Compare the numerators: 4 > 1.
  • Therefore, 4/11 > 1/11.

4. Comparing Fractions with Unlike Denominators

Comparing fractions with different denominators requires an additional step: finding a common denominator. This involves converting the fractions to equivalent fractions with the same denominator, making it possible to directly compare their numerators. The most common method for finding a common denominator is to use the Least Common Multiple (LCM) of the denominators.

Once the fractions have the same denominator, you can then compare their numerators as described in the previous section. This process ensures that you are comparing equal-sized parts of the whole, allowing for an accurate assessment of which fraction is larger or smaller.

4.1 Finding the Least Common Multiple (LCM)

The Least Common Multiple (LCM) is the smallest multiple that is common to two or more numbers. To find the LCM of two denominators, you can list the multiples of each number and identify the smallest multiple they have in common.

For example, to find the LCM of 4 and 6:

• Multiples of 4: 4, 8, 12, 16, 20, 24, …
• Multiples of 6: 6, 12, 18, 24, 30, …

The LCM of 4 and 6 is 12.

4.2 Converting Fractions to Equivalent Fractions

Once you have found the LCM, you need to convert each fraction to an equivalent fraction with the LCM as the denominator. This involves multiplying both the numerator and the denominator of each fraction by a factor that makes the denominator equal to the LCM.

For example, if you want to compare 1/4 and 2/6, and you have determined that the LCM of 4 and 6 is 12:

• To convert 1/4 to an equivalent fraction with a denominator of 12, multiply both the numerator and denominator by 3: (1 3) / (4 3) = 3/12.
• To convert 2/6 to an equivalent fraction with a denominator of 12, multiply both the numerator and denominator by 2: (2 2) / (6 2) = 4/12.

Now that both fractions have the same denominator (12), you can compare their numerators.

4.3 Step-by-Step Guide

1. Find the LCM: Determine the Least Common Multiple (LCM) of the denominators.
2. Convert to Equivalent Fractions: Convert each fraction to an equivalent fraction with the LCM as the denominator.
3. Compare the Numerators: Compare the numerators of the equivalent fractions.
4. Determine the Larger Fraction: The fraction with the larger numerator is the larger fraction.

4.4 Examples and Illustrations

Let’s illustrate this with a few examples:

• Example 1: Compare 2/5 and 3/7.

  • Find the LCM of 5 and 7: LCM(5, 7) = 35.
  • Convert 2/5 to an equivalent fraction: (2 7) / (5 7) = 14/35.
  • Convert 3/7 to an equivalent fraction: (3 5) / (7 5) = 15/35.
  • Compare the numerators: 15 > 14.
  • Therefore, 3/7 > 2/5.

• Example 2: Compare 1/3 and 2/9.

  • Find the LCM of 3 and 9: LCM(3, 9) = 9.
  • Convert 1/3 to an equivalent fraction: (1 3) / (3 3) = 3/9.
  • Convert 2/9 to an equivalent fraction: 2/9 (already has the desired denominator).
  • Compare the numerators: 3 > 2.
  • Therefore, 1/3 > 2/9.

5. Decimal Method of Comparing Fractions

Another effective method for comparing fractions is to convert them to decimal numbers. This involves dividing the numerator by the denominator to obtain the decimal equivalent of each fraction. Once the fractions are in decimal form, you can easily compare their values to determine which fraction is larger or smaller.

This method is particularly useful when dealing with fractions that have denominators that are not easily converted to a common multiple. By converting to decimals, you can directly compare the numerical values and avoid the complexities of finding a common denominator.

5.1 Converting Fractions to Decimals

To convert a fraction to a decimal, simply divide the numerator by the denominator. For example, to convert 3/4 to a decimal, divide 3 by 4:

3 ÷ 4 = 0.75

Therefore, the decimal equivalent of 3/4 is 0.75.

5.2 Step-by-Step Guide

1. Convert to Decimals: Convert each fraction to its decimal equivalent by dividing the numerator by the denominator.
2. Compare the Decimals: Compare the decimal values.
3. Determine the Larger Fraction: The fraction with the larger decimal value is the larger fraction.

5.3 Examples and Illustrations

Let’s illustrate this with a few examples:

• Example 1: Compare 3/5 and 5/8.

  • Convert 3/5 to a decimal: 3 ÷ 5 = 0.6.
  • Convert 5/8 to a decimal: 5 ÷ 8 = 0.625.
  • Compare the decimal values: 0.625 > 0.6.
  • Therefore, 5/8 > 3/5.

• Example 2: Compare 1/4 and 2/10.

  • Convert 1/4 to a decimal: 1 ÷ 4 = 0.25.
  • Convert 2/10 to a decimal: 2 ÷ 10 = 0.2.
  • Compare the decimal values: 0.25 > 0.2.
  • Therefore, 1/4 > 2/10.

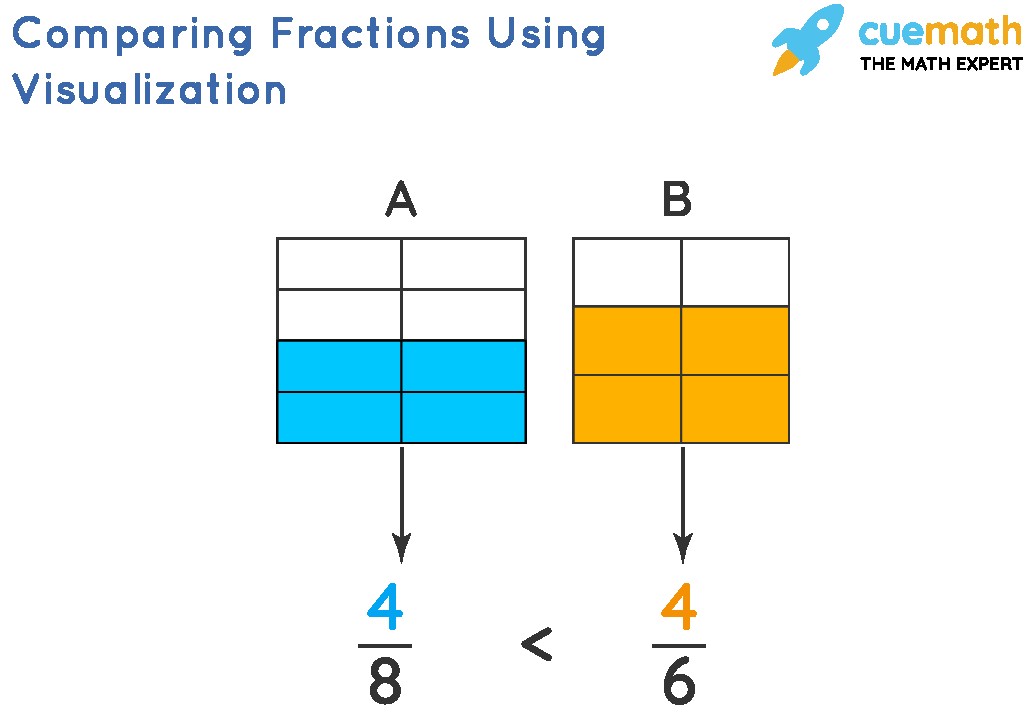
6. Comparing Fractions Using Visualization

Visualizing fractions can provide an intuitive understanding of their relative sizes. This method involves representing fractions using diagrams or models, such as circles or rectangles, to visually compare their values. By dividing the shapes into equal parts and shading the appropriate number of parts, you can easily see which fraction represents a larger or smaller portion of the whole.

This method is particularly useful for learners who benefit from visual aids and can help to reinforce the concept of fractions as parts of a whole. Visual comparison can make abstract concepts more concrete and accessible.

6.1 Using Diagrams and Models

To compare fractions using visualization, follow these steps:

1. Draw Identical Shapes: Draw two or more identical shapes (e.g., circles or rectangles) to represent the whole.
2. Divide into Equal Parts: Divide each shape into the number of equal parts indicated by the denominator of each fraction.
3. Shade the Parts: Shade the number of parts indicated by the numerator of each fraction.
4. Compare the Shaded Areas: Visually compare the shaded areas to determine which fraction represents a larger portion.

6.2 Step-by-Step Guide

1. Draw Shapes: Draw identical shapes for each fraction.
2. Divide and Shade: Divide each shape into equal parts according to the denominator and shade the parts according to the numerator.
3. Compare Visually: Compare the shaded areas to determine the larger fraction.

6.3 Examples and Illustrations

Let’s illustrate this with a few examples:

• Example 1: Compare 2/4 and 3/6.

  • Draw two identical rectangles.
  • Divide the first rectangle into 4 equal parts and shade 2 parts (representing 2/4).
  • Divide the second rectangle into 6 equal parts and shade 3 parts (representing 3/6).
  • Visually compare the shaded areas. In this case, the shaded areas are equal, so 2/4 = 3/6.

• Example 2: Compare 1/3 and 2/5.

  • Draw two identical circles.
  • Divide the first circle into 3 equal parts and shade 1 part (representing 1/3).
  • Divide the second circle into 5 equal parts and shade 2 parts (representing 2/5).
  • Visually compare the shaded areas. You will see that 2/5 covers a larger area than 1/3, so 2/5 > 1/3.

7. Comparing Fractions Using Cross Multiplication

Cross multiplication is a quick and efficient method for comparing two fractions without finding a common denominator. This technique involves multiplying the numerator of one fraction by the denominator of the other fraction and comparing the resulting products. The fraction with the larger product is the larger fraction.

This method is particularly useful when you need to compare fractions quickly or when finding the LCM is cumbersome. Cross multiplication provides a direct comparison of the fractional values.

7.1 The Cross Multiplication Technique

To compare two fractions using cross multiplication, follow these steps:

1. Multiply Diagonally: Multiply the numerator of the first fraction by the denominator of the second fraction.
2. Multiply Diagonally: Multiply the numerator of the second fraction by the denominator of the first fraction.
3. Compare the Products: Compare the two products. The fraction corresponding to the larger product is the larger fraction.

7.2 Step-by-Step Guide

1. Cross Multiply: Multiply the numerator of the first fraction by the denominator of the second fraction, and vice versa.
2. Compare Products: Compare the resulting products.
3. Determine the Larger Fraction: The fraction with the larger product is the larger fraction.

7.3 Examples and Illustrations

Let’s illustrate this with a few examples:

• Example 1: Compare 2/3 and 3/4.

  • Cross multiply: 2 4 = 8 and 3 3 = 9.
  • Compare the products: 9 > 8.
  • Therefore, 3/4 > 2/3.

• Example 2: Compare 1/5 and 2/9.

  • Cross multiply: 1 9 = 9 and 2 5 = 10.
  • Compare the products: 10 > 9.
  • Therefore, 2/9 > 1/5.

8. Practical Examples of Comparing Fractions in Real Life

Comparing fractions is not just an abstract mathematical exercise; it has numerous practical applications in our daily lives. Understanding how to compare fractions can help us make informed decisions in various situations, from cooking and shopping to managing finances and understanding data.

By recognizing the importance of comparing fractions in real-life scenarios, we can appreciate the value of mastering these skills and apply them effectively in our everyday activities.

8.1 Cooking and Baking

In cooking and baking, recipes often call for fractional amounts of ingredients. Comparing fractions can help you adjust recipes, scale ingredients, and ensure accurate measurements. For example, if a recipe calls for 1/2 cup of flour and you want to double the recipe, you need to know that 1/2 + 1/2 = 1 cup.

8.2 Shopping and Budgeting

When shopping, you often need to compare prices that are expressed as fractions. For example, if one store offers a 1/4 discount on an item and another offers a 1/3 discount, knowing how to compare these fractions can help you determine which offer is better.

8.3 Time Management

Comparing fractions can also be useful in time management. For example, if you spend 1/3 of your day working and 1/4 of your day sleeping, you can compare these fractions to understand how much time you spend on each activity relative to the whole day.

8.4 Understanding Data

Fractions are often used to represent data in reports and statistics. Comparing fractions can help you interpret this data and draw meaningful conclusions. For example, if a pie chart shows that 3/8 of the population prefers one product and 2/8 prefers another, you can easily compare these fractions to see which product is more popular.

9. Tips and Tricks for Mastering Fraction Comparison

Mastering fraction comparison requires practice and a solid understanding of the underlying concepts. Here are some tips and tricks to help you improve your skills and become more proficient in comparing fractions:

• Practice Regularly: The more you practice comparing fractions, the more comfortable and confident you will become.
• Use Visual Aids: Visual aids such as diagrams and models can help you understand the relative sizes of fractions and make comparisons more intuitive.
• Memorize Common Equivalents: Memorizing common fraction-to-decimal equivalents can save time when using the decimal method.
• Check Your Work: Always double-check your work to ensure that you have correctly applied the comparison methods and arrived at the right answer.
• Understand the Concepts: Focus on understanding the underlying concepts rather than just memorizing rules. This will help you apply the methods more effectively in different situations.

9.1 Common Mistakes to Avoid

• Ignoring the Denominator: Always pay attention to the denominator when comparing fractions. Comparing numerators without considering the denominators can lead to incorrect conclusions.
• Incorrect LCM: Make sure to find the Least Common Multiple (LCM) correctly. An incorrect LCM will result in inaccurate equivalent fractions and incorrect comparisons.
• Misapplying Cross Multiplication: Ensure that you are multiplying the correct numerators and denominators when using cross multiplication.
• Forgetting to Simplify: Always simplify fractions before comparing them, if possible. This can make the comparison process easier and more accurate.

10. Frequently Asked Questions (FAQs) about Comparing Fractions

10.1 What Does Comparing Fractions Mean?

Comparing fractions means determining the relative size or value of two or more fractions. This involves identifying which fraction is larger, smaller, or equal to another fraction.

10.2 How Do You Compare Fractions with Different Denominators?

To compare fractions with different denominators, you need to find a common denominator. This involves converting the fractions to equivalent fractions with the same denominator, making it possible to directly compare their numerators. The most common method for finding a common denominator is to use the Least Common Multiple (LCM) of the denominators.

10.3 What is the Easiest Way to Compare Fractions?

The easiest way to compare fractions is often to convert them to decimal numbers. This involves dividing the numerator by the denominator to obtain the decimal equivalent of each fraction. Once the fractions are in decimal form, you can easily compare their values.

10.4 Can You Compare Fractions with Negative Signs?

Yes, you can compare fractions with negative signs. Remember that negative fractions are always smaller than positive fractions. When comparing two negative fractions, the fraction with the smaller absolute value is the larger fraction.

10.5 How Do You Compare Mixed Numbers?

To compare mixed numbers, you can either convert them to improper fractions or compare the whole number parts first. If the whole number parts are different, the mixed number with the larger whole number is the larger mixed number. If the whole number parts are the same, compare the fractional parts as you would with regular fractions.

10.6 What is Cross Multiplication and How Does It Work?

Cross multiplication is a method for comparing two fractions without finding a common denominator. It involves multiplying the numerator of one fraction by the denominator of the other fraction and comparing the resulting products. The fraction with the larger product is the larger fraction.

10.7 Why is it Important to Know How to Compare Fractions?

Knowing how to compare fractions is important because it has numerous practical applications in our daily lives, such as cooking, shopping, budgeting, time management, and understanding data.

10.8 How Can Visual Aids Help in Comparing Fractions?

Visual aids such as diagrams and models can help you understand the relative sizes of fractions and make comparisons more intuitive. By representing fractions visually, you can easily see which fraction represents a larger or smaller portion of the whole.

10.9 What Should I Do If the Fractions Are Too Complex to Compare Easily?

If the fractions are too complex to compare easily, try simplifying them first. You can also convert them to decimal numbers or use cross multiplication to compare them more efficiently.

10.10 Are There Any Online Tools That Can Help Me Compare Fractions?

Yes, there are many online tools and calculators that can help you compare fractions. These tools can be useful for checking your work or for quickly comparing complex fractions.

11. Conclusion: Mastering the Art of Fraction Comparison

In conclusion, mastering the art of fraction comparison is a valuable skill that can benefit you in various aspects of life. By understanding the different methods and techniques for comparing fractions, you can confidently solve mathematical problems, make informed decisions, and enhance your overall numerical literacy.

Whether you prefer using common denominators, converting to decimals, visualizing fractions, or applying cross multiplication, the key is to practice regularly and develop a solid understanding of the underlying concepts. With dedication and effort, you can become proficient in comparing fractions and unlock the many practical applications this skill offers.

For more in-depth comparisons and resources, visit COMPARE.EDU.VN. We offer comprehensive guides and tools to help you make informed decisions and compare a wide range of topics, from education to everyday products.

Are you struggling to compare different options and make the right choice? Visit COMPARE.EDU.VN today. We provide detailed, objective comparisons that help you weigh the pros and cons, compare features and prices, and read user reviews. Let us help you make smarter decisions! Visit us at 333 Comparison Plaza, Choice City, CA 90210, United States. For inquiries, contact us via Whatsapp at +1 (626) 555-9090 or visit our website at compare.edu.vn.