How To Explain Comparing Fractions: A Comprehensive Guide

Comparing fractions can seem daunting, but it’s a fundamental skill in mathematics with real-world applications. At COMPARE.EDU.VN, we aim to simplify complex concepts, providing accessible explanations and practical methods for comparing fractions effectively. Discover how to compare fractions using various techniques and understand the underlying principles.

Table of Contents

Understanding the Basics: What Are Fractions?
Why Is Comparing Fractions Important?
Method 1: Comparing Fractions with the Same Denominator
Method 2: Comparing Fractions with the Same Numerator
Method 3: Comparing Fractions with Different Denominators (Finding a Common Denominator)
Step-by-Step Guide to Finding the Least Common Denominator (LCD)
Method 4: Cross-Multiplication Method
Method 5: Converting Fractions to Decimals
Method 6: Using Benchmarks to Compare Fractions
Method 7: Visual Models for Comparing Fractions
Comparing Mixed Numbers
Comparing Improper Fractions
Common Mistakes to Avoid When Comparing Fractions
Real-World Applications of Comparing Fractions
Advanced Techniques for Comparing Fractions
Using COMPARE.EDU.VN for Fraction Comparisons
Practical Examples and Exercises
Tips for Teaching Comparing Fractions
The Role of Fraction Comparison in Higher Mathematics
Tools and Resources for Fraction Comparison
Frequently Asked Questions (FAQs) about Comparing Fractions
Conclusion: Mastering Fraction Comparison

1. Understanding the Basics: What Are Fractions?

Before diving into How To Explain Comparing Fractions, it’s crucial to understand what a fraction is. A fraction represents a part of a whole. It consists of two primary components: the numerator and the denominator. The numerator (the top number) indicates how many parts we have, while the denominator (the bottom number) indicates the total number of equal parts that make up the whole. For instance, in the fraction 3/4, 3 is the numerator, and 4 is the denominator, meaning we have 3 parts out of a total of 4. This foundational knowledge is essential for grasping the concept of fraction comparison.

2. Why Is Comparing Fractions Important?

Comparing fractions is not just an academic exercise; it’s a practical skill with numerous real-world applications. Whether you’re adjusting recipe ingredients, managing finances, or understanding data in charts and graphs, knowing how to compare fractions allows you to make informed decisions. This skill helps in understanding proportions, ratios, and relative quantities, making it an indispensable tool in everyday life and various professional fields. Mastering equivalent fractions and comparing fractions is beneficial in various real-world scenarios.

3. Method 1: Comparing Fractions with the Same Denominator

The simplest scenario in fraction comparison occurs when the fractions share the same denominator. In this case, the fraction with the larger numerator is the larger fraction. For example, when comparing 2/5 and 4/5, since both fractions have the same denominator (5), we only need to compare the numerators. Because 4 is greater than 2, we can conclude that 4/5 is greater than 2/5. This method provides a straightforward way to determine which fraction represents a larger portion of the whole.

4. Method 2: Comparing Fractions with the Same Numerator

When comparing fractions with the same numerator but different denominators, the rule is reversed. The fraction with the smaller denominator is the larger fraction. This is because if you divide a whole into fewer parts, each part will be larger. For instance, when comparing 1/4 and 1/8, both fractions have the same numerator (1). Since 4 is smaller than 8, 1/4 is greater than 1/8. This might seem counterintuitive at first, but visualizing it helps to reinforce the concept.

5. Method 3: Comparing Fractions with Different Denominators (Finding a Common Denominator)

Comparing fractions with different denominators requires a bit more work. The most common approach is to find a common denominator, allowing you to compare the fractions directly. This involves finding a number that is a multiple of both denominators and then converting each fraction to an equivalent fraction with this common denominator. Once the denominators are the same, you can compare the numerators as described in Method 1. This is a crucial skill for anyone learning how to explain comparing fractions.

6. Step-by-Step Guide to Finding the Least Common Denominator (LCD)

The Least Common Denominator (LCD) is the smallest common multiple of the denominators of two or more fractions. Here’s a step-by-step guide to finding it:

List the Multiples: List the multiples of each denominator.
Identify Common Multiples: Find the multiples that the denominators have in common.
Choose the Smallest: Select the smallest of these common multiples. This is your LCD.
Convert the Fractions: Convert each fraction to an equivalent fraction with the LCD as the new denominator.
- For example, compare 1/3 and 1/4:
  - Multiples of 3: 3, 6, 9, 12, 15…
  - Multiples of 4: 4, 8, 12, 16…
  - The LCD is 12.
  - Convert 1/3 to 4/12 (multiply numerator and denominator by 4).
  - Convert 1/4 to 3/12 (multiply numerator and denominator by 3).
  - Now, compare 4/12 and 3/12.

7. Method 4: Cross-Multiplication Method

Cross-multiplication provides a quick way to compare two fractions without explicitly finding a common denominator. To use this method, multiply the numerator of the first fraction by the denominator of the second fraction, and then multiply the numerator of the second fraction by the denominator of the first fraction. Compare the two products. If the first product is larger, then the first fraction is larger; if the second product is larger, then the second fraction is larger. For example, to compare 3/4 and 5/7:

Multiply 3 (numerator of the first fraction) by 7 (denominator of the second fraction): 3 * 7 = 21.
Multiply 5 (numerator of the second fraction) by 4 (denominator of the first fraction): 5 * 4 = 20.
Since 21 is greater than 20, 3/4 is greater than 5/7.

8. Method 5: Converting Fractions to Decimals

Another effective method for comparing fractions is to convert them to decimals. To do this, simply divide the numerator by the denominator. Once both fractions are in decimal form, they can be easily compared. For instance, to compare 1/4 and 3/8:

Convert 1/4 to a decimal: 1 ÷ 4 = 0.25.
Convert 3/8 to a decimal: 3 ÷ 8 = 0.375.
Comparing the decimals, 0.375 is greater than 0.25, so 3/8 is greater than 1/4.

9. Method 6: Using Benchmarks to Compare Fractions

Using benchmarks involves comparing fractions to common reference points, such as 0, 1/2, and 1. This method is particularly useful for quickly estimating and comparing fractions without needing precise calculations. For example, to compare 3/5 and 5/8:

Recognize that 3/5 is slightly more than 1/2 (since 2.5/5 is 1/2).
Recognize that 5/8 is also slightly more than 1/2 (since 4/8 is 1/2).
To compare them further, notice that 3/5 is closer to 1/2 than 5/8, meaning 3/5 is slightly smaller than 5/8.

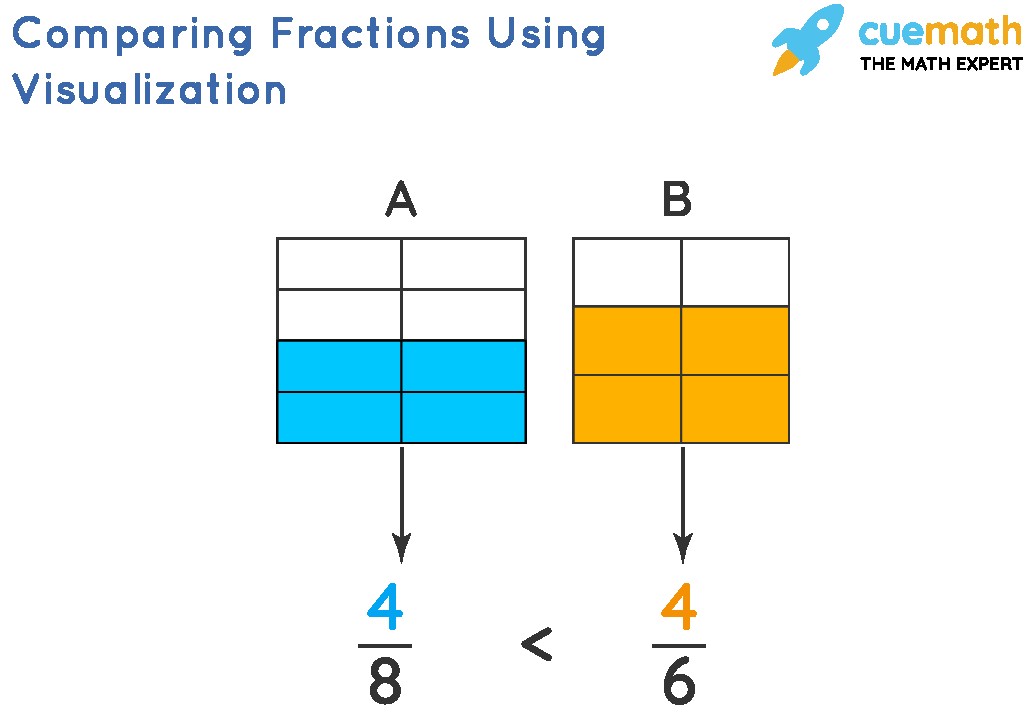
10. Method 7: Visual Models for Comparing Fractions

Visual models, such as pie charts, bar models, or number lines, can provide a concrete representation of fractions, making them easier to compare. For example, draw two equal-sized rectangles. Divide one into 4 equal parts and shade 1 part to represent 1/4. Divide the other into 8 equal parts and shade 3 parts to represent 3/8. By visually comparing the shaded areas, you can see that 3/8 is larger than 1/4.

11. Comparing Mixed Numbers

Mixed numbers consist of a whole number and a fraction. To compare mixed numbers, first compare the whole numbers. If the whole numbers are different, the mixed number with the larger whole number is greater. If the whole numbers are the same, compare the fractional parts using any of the methods discussed earlier. For example, to compare 3 1/4 and 3 2/5:

The whole numbers are the same (both are 3), so compare the fractions 1/4 and 2/5.
Convert them to a common denominator: 1/4 = 5/20 and 2/5 = 8/20.
Since 8/20 is greater than 5/20, 3 2/5 is greater than 3 1/4.

12. Comparing Improper Fractions

Improper fractions have a numerator that is greater than or equal to the denominator. To compare improper fractions, you can use similar methods as with proper fractions, such as finding a common denominator or cross-multiplication. Alternatively, you can convert the improper fractions to mixed numbers and then compare as described above. For example, to compare 7/3 and 9/4:

Convert to mixed numbers: 7/3 = 2 1/3 and 9/4 = 2 1/4.
The whole numbers are the same (both are 2), so compare the fractions 1/3 and 1/4.
Since 1/3 is greater than 1/4, 7/3 is greater than 9/4.

13. Common Mistakes to Avoid When Comparing Fractions

Assuming Larger Denominator Means Larger Fraction: Remember that when numerators are the same, a larger denominator means the fraction represents smaller parts of the whole.
Not Finding a Common Denominator: When denominators are different, you must find a common denominator before comparing numerators.
Incorrectly Applying Cross-Multiplication: Ensure you multiply the correct numerators and denominators and compare the products accurately.
Ignoring the Whole Number in Mixed Numbers: Always compare the whole numbers first before comparing the fractional parts of mixed numbers.
Rushing the Process: Take your time to ensure accuracy, especially when converting fractions or finding common denominators.

14. Real-World Applications of Comparing Fractions

Understanding how to compare fractions is valuable in many real-world scenarios:

Cooking: Adjusting recipe quantities (e.g., doubling or halving ingredients).
Construction: Measuring and cutting materials (e.g., lumber or fabric).
Finance: Calculating proportions of investments or expenses.
Data Analysis: Interpreting statistics and survey results presented as fractions or percentages.
Time Management: Allocating time to different tasks based on fractional parts of an hour.

15. Advanced Techniques for Comparing Fractions

For more complex fraction comparison problems, consider these advanced techniques:

Using Prime Factorization: Simplify fractions by finding the prime factors of the numerators and denominators.
Converting to Percentages: Convert fractions to percentages for easier comparison.
Applying Properties of Inequality: Use properties of inequality to solve complex comparison problems involving variables.

16. Using COMPARE.EDU.VN for Fraction Comparisons

COMPARE.EDU.VN offers a variety of tools and resources to help you compare fractions effectively. Our website provides:

Fraction Comparison Calculators: Input your fractions, and our tool will instantly compare them and show the results.
Educational Articles: In-depth guides and tutorials on fraction comparison techniques.
Practice Exercises: Quizzes and worksheets to test your understanding and skills.
Visual Aids: Interactive models and diagrams to help you visualize fractions and their relative sizes.

Whether you’re a student, teacher, or professional, COMPARE.EDU.VN is your go-to resource for mastering fraction comparison.

17. Practical Examples and Exercises

Here are a few practical examples and exercises to help you practice comparing fractions:

Example 1:
Compare 2/3 and 3/5.

Solution:
- Using cross-multiplication: 2 5 = 10 and 3 3 = 9.
- Since 10 > 9, 2/3 > 3/5.

Example 2:
Compare 1 1/2 and 1 3/4.

Solution:
- Convert to improper fractions: 1 1/2 = 3/2 and 1 3/4 = 7/4.
- Find a common denominator: 3/2 = 6/4 and 7/4.
- Since 7/4 > 6/4, 1 3/4 > 1 1/2.

Exercise 1:
Compare 4/7 and 5/9.

Exercise 2:
Compare 2 2/3 and 2 5/8.

18. Tips for Teaching Comparing Fractions

Start with Concrete Examples: Use real-world objects and visual aids to introduce the concept of fractions and their relative sizes.
Emphasize the Importance of Equal Parts: Ensure students understand that fractions represent equal parts of a whole.
Use Different Methods: Teach multiple methods for comparing fractions, allowing students to choose the one that works best for them.
Encourage Estimation: Help students develop estimation skills by comparing fractions to benchmarks like 0, 1/2, and 1.
Provide Plenty of Practice: Offer a variety of exercises and problems to reinforce understanding and build confidence.

19. The Role of Fraction Comparison in Higher Mathematics

Fraction comparison is a foundational skill that is essential for success in higher mathematics. It forms the basis for understanding ratios, proportions, and percentages, which are used extensively in algebra, geometry, calculus, and statistics. A strong understanding of fraction comparison also helps in developing critical thinking and problem-solving skills, which are valuable in all areas of mathematics.

20. Tools and Resources for Fraction Comparison

There are many tools and resources available to help you learn and practice comparing fractions:

Online Calculators: Websites like COMPARE.EDU.VN offer free fraction comparison calculators.
Educational Websites: Khan Academy, Math Playground, and other educational websites provide tutorials, exercises, and games on fractions.
Textbooks and Workbooks: Many math textbooks and workbooks include sections on fraction comparison.
Mobile Apps: Several mobile apps offer interactive fraction games and exercises.

21. Frequently Asked Questions (FAQs) about Comparing Fractions

Q: What does it mean to compare fractions?
A: Comparing fractions involves determining which fraction is larger or smaller, or if they are equal.

Q: Why is it important to find a common denominator when comparing fractions?
A: Finding a common denominator allows you to compare the numerators directly, as the fractions then represent equal parts of the same whole.

Q: Can you always use cross-multiplication to compare fractions?
A: Yes, cross-multiplication is a reliable method for comparing any two fractions.

Q: How do you compare mixed numbers?
A: First, compare the whole numbers. If they are the same, compare the fractional parts using any of the methods for comparing fractions.

Q: What is the easiest way to compare fractions?
A: Converting fractions to decimals is often the easiest way to compare them, as you can directly compare the decimal values.

Q: Is comparing fractions the same as simplifying fractions?
A: No, comparing fractions is about determining which fraction is larger or smaller, while simplifying fractions is about reducing a fraction to its lowest terms.

Q: What if I’m comparing more than two fractions?
A: Find a common denominator for all fractions, then compare the numerators.

Q: Can I use a calculator to compare fractions?
A: Yes, most calculators can convert fractions to decimals, making comparison easy.

Q: How does understanding fractions help in real life?
A: Understanding fractions is essential for cooking, measuring, finance, and many other everyday tasks.

Q: Where can I find more resources to practice comparing fractions?
A: Websites like COMPARE.EDU.VN, Khan Academy, and Math Playground offer many resources for practicing fraction comparison.

22. Conclusion: Mastering Fraction Comparison

Mastering how to explain comparing fractions is a valuable skill that can be applied in many areas of life. By understanding the basic concepts and practicing the various methods discussed in this guide, you can confidently compare fractions and make informed decisions. Visit COMPARE.EDU.VN for more tools, resources, and support to help you on your journey to mastering fraction comparison. Remember, practice makes perfect, so keep exploring and experimenting with fractions until you feel comfortable with the concepts.

If you’re still finding it challenging to compare fractions, don’t hesitate to seek help from a teacher, tutor, or online resources like COMPARE.EDU.VN. We are here to provide you with the tools and support you need to succeed. Our team at COMPARE.EDU.VN is dedicated to making learning accessible and enjoyable. Visit us at 333 Comparison Plaza, Choice City, CA 90210, United States, or reach out via WhatsApp at +1 (626) 555-9090. Explore our website, compare.edu.vn, to discover a wealth of resources that can help you compare and make the best choices. We’re here to help you compare fractions and many other things with ease and confidence.