Comparing multiple fractions can seem daunting, but it’s a fundamental skill with applications in everyday life, from cooking to finance. At COMPARE.EDU.VN, we provide simple yet effective methods to master this skill. Learn how to compare fractions effectively and boost your math proficiency with these comparison techniques.
1. Understanding the Basics of Fractions
Before diving into the comparison methods, it’s essential to understand what a fraction represents. A fraction is a part of a whole, expressed as a numerator (the top number) over a denominator (the bottom number). The numerator indicates how many parts of the whole you have, while the denominator indicates the total number of equal parts that make up the whole. Different types of fractions exist, including:
- Proper Fractions: The numerator is less than the denominator (e.g., 2/5).
- Improper Fractions: The numerator is greater than or equal to the denominator (e.g., 7/3).
- Mixed Numbers: A whole number and a proper fraction combined (e.g., 2 1/4).
Understanding these different forms is the first step in mastering fraction comparison.
2. Comparing Fractions with the Same Denominator
The easiest scenario for comparing fractions is when they share the same denominator. In this case, the fraction with the larger numerator is the larger fraction.
Example:
Consider the fractions 3/8, 5/8, and 1/8. Since they all have the same denominator (8), we simply compare the numerators:
- 1 < 3 < 5
Therefore:
- 1/8 < 3/8 < 5/8
This method works because the denominator represents the size of each part of the whole. If the parts are the same size, then more parts mean a larger fraction.
3. Comparing Fractions with the Same Numerator
When fractions have the same numerator but different denominators, the fraction with the smaller denominator is the larger fraction. This might seem counterintuitive at first, but it makes sense when you consider what the denominator represents. The denominator indicates how many parts the whole is divided into. If the whole is divided into fewer parts, each part is larger.
Example:
Consider the fractions 2/3, 2/5, and 2/7. They all have the same numerator (2), so we compare the denominators:
- 3 < 5 < 7
Therefore:
- 2/3 > 2/5 > 2/7
In this case, 2/3 represents two larger parts of the whole, while 2/7 represents two smaller parts of the whole.
4. Finding a Common Denominator: The LCM Method
When fractions have different numerators and different denominators, we need a method to make a direct comparison. The most common method is to find a common denominator. The least common denominator (LCD) is the least common multiple (LCM) of the denominators of the fractions.
4.1. How to Find the LCM
There are several ways to find the LCM of two or more numbers:
- Listing Multiples: List the multiples of each number until you find a common multiple. The smallest common multiple is the LCM.
- Prime Factorization: Find the prime factorization of each number. The LCM is the product of the highest power of each prime factor that appears in any of the factorizations.
Example:
Find the LCM of 4, 6, and 10:
- Listing Multiples:
- Multiples of 4: 4, 8, 12, 16, 20, 24, 28, 32, 36, 40, 44, 48, 52, 56, 60…
- Multiples of 6: 6, 12, 18, 24, 30, 36, 42, 48, 54, 60…
- Multiples of 10: 10, 20, 30, 40, 50, 60…
- The LCM is 60.
- Prime Factorization:
- 4 = 2^2
- 6 = 2 x 3
- 10 = 2 x 5
- LCM = 2^2 x 3 x 5 = 60
4.2. Converting Fractions to Equivalent Fractions
Once you have the LCM, you need to convert each fraction to an equivalent fraction with the LCM as the new denominator. To do this, divide the LCM by the original denominator, and then multiply both the numerator and denominator of the original fraction by the result.
Example:
Compare the fractions 1/4, 2/6, and 3/10. We found that the LCM of 4, 6, and 10 is 60. Now we convert each fraction:
- 1/4 = (1 x 15) / (4 x 15) = 15/60
- 2/6 = (2 x 10) / (6 x 10) = 20/60
- 3/10 = (3 x 6) / (10 x 6) = 18/60
Now that all the fractions have the same denominator, we can easily compare the numerators:
- 15 < 18 < 20
Therefore:
- 15/60 < 18/60 < 20/60
- 1/4 < 3/10 < 2/6
This method ensures that you’re comparing fractions with equal-sized parts, making the comparison accurate.
5. Comparing Fractions Using Cross-Multiplication
Cross-multiplication is a quick method for comparing two fractions at a time. To use this method, multiply the numerator of the first fraction by the denominator of the second fraction, and multiply the numerator of the second fraction by the denominator of the first fraction. Then, compare the two products.
Example:
Compare 3/7 and 5/9:
- 3 x 9 = 27
- 5 x 7 = 35
Since 27 < 35, we know that 3/7 < 5/9.
5.1. Extending Cross-Multiplication to Multiple Fractions
While cross-multiplication is efficient for comparing two fractions, it can be extended to compare multiple fractions by comparing them in pairs. However, this approach becomes less efficient as the number of fractions increases.
Example:
Compare 1/3, 2/5, and 3/8:
- Compare 1/3 and 2/5:
- 1 x 5 = 5
- 2 x 3 = 6
- Since 5 < 6, 1/3 < 2/5
- Compare 2/5 and 3/8:
- 2 x 8 = 16
- 3 x 5 = 15
- Since 16 > 15, 2/5 > 3/8
Combining these results, we get:
- 1/3 < 3/8 < 2/5
Keep in mind that this method can be more prone to errors when dealing with a large number of fractions, as each comparison is separate and needs to be carefully tracked.
6. Converting Fractions to Decimals
Another way to compare fractions is to convert them to decimals. To do this, divide the numerator by the denominator. Then, compare the decimal values.
Example:
Compare 3/8, 5/12, and 7/20:
- 3/8 = 0.375
- 5/12 = 0.4166… (approximately 0.417)
- 7/20 = 0.35
Comparing the decimal values:
- 0.35 < 0.375 < 0.417
Therefore:
- 7/20 < 3/8 < 5/12
6.1. Advantages and Disadvantages of Decimal Conversion
The decimal conversion method is straightforward and can be particularly useful when dealing with fractions that have large or difficult-to-work-with denominators. However, it’s important to note that some fractions result in repeating decimals, which may need to be rounded for comparison. Rounding can introduce slight inaccuracies, so it’s essential to use an appropriate level of precision.
7. Using Benchmarks for Quick Comparison
Benchmarks are common fractions that you can use as reference points to quickly estimate the size of other fractions. Common benchmarks include 0, 1/4, 1/2, 3/4, and 1. By comparing fractions to these benchmarks, you can often get a good sense of their relative size without performing exact calculations.
Example:
Compare 2/5, 5/8, and 1/3 using benchmarks:
- 2/5 is slightly less than 1/2
- 5/8 is slightly more than 1/2
- 1/3 is slightly more than 1/4
From this, we can infer that:
- 1/3 < 2/5 < 5/8
Benchmarks are especially helpful for quick mental estimations and can provide a useful check on the results of more precise methods.
8. Visual Models: Comparing Fractions Geometrically
Visual models, such as pie charts, bar diagrams, and number lines, can be powerful tools for understanding and comparing fractions. These models provide a geometric representation of fractions, making it easier to see their relative sizes.
8.1. Pie Charts
Pie charts represent the whole as a circle, with each fraction represented as a slice of the pie. The size of the slice corresponds to the fraction’s value.
Example:
To compare 1/4, 1/3, and 1/2 using pie charts, draw three circles of the same size. Divide each circle into the appropriate number of slices (4, 3, and 2, respectively), and shade one slice in each circle. By visually comparing the shaded areas, you can see that 1/4 < 1/3 < 1/2.
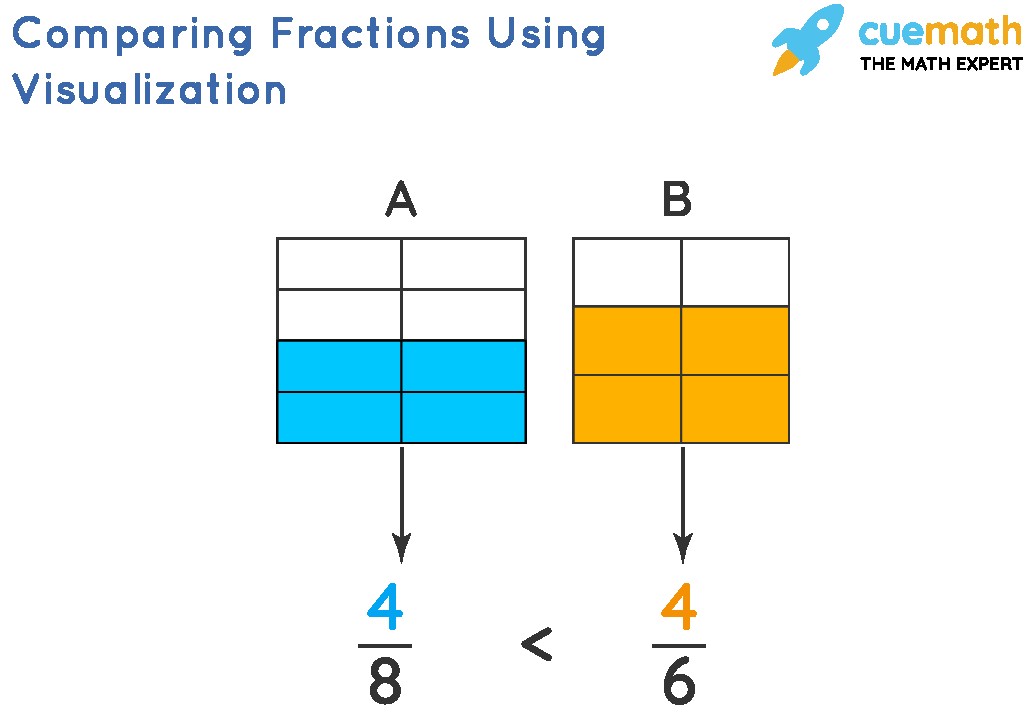
8.2. Bar Diagrams
Bar diagrams represent the whole as a rectangle, with each fraction represented as a portion of the bar. The length of the portion corresponds to the fraction’s value.
Example:
To compare 2/5, 3/4, and 1/2 using bar diagrams, draw three rectangles of the same size. Divide each rectangle into the appropriate number of sections (5, 4, and 2, respectively), and shade the appropriate number of sections (2, 3, and 1, respectively). By visually comparing the shaded lengths, you can see that 1/2 < 2/5 < 3/4.
8.3. Number Lines
Number lines represent fractions as points along a line, with the whole represented as the interval from 0 to 1. The position of each point corresponds to the fraction’s value.
Example:
To compare 1/4, 2/3, and 1/2 using a number line, draw a line and mark 0 and 1. Divide the line into appropriate intervals to represent each fraction (fourths, thirds, and halves). Mark the points corresponding to 1/4, 2/3, and 1/2. By visually comparing the positions of the points, you can see that 1/4 < 1/2 < 2/3.
9. Tips and Tricks for Efficient Fraction Comparison
- Simplify Fractions First: Before comparing, simplify each fraction to its lowest terms. This makes the numbers smaller and easier to work with.
- Look for Relationships: Sometimes, you can quickly compare fractions by noticing relationships between them. For example, if one fraction is clearly greater than 1/2 and another is clearly less than 1/2, you don’t need to perform any calculations to compare them.
- Practice Regularly: The more you practice comparing fractions, the faster and more confident you’ll become.
10. Real-World Applications of Fraction Comparison
Fraction comparison is not just an abstract mathematical concept. It has many practical applications in everyday life.
- Cooking: When following a recipe, you often need to compare fractions of ingredients to adjust quantities or substitute ingredients.
- Finance: Comparing fractions is essential when calculating interest rates, discounts, or investment returns.
- Construction: When building or renovating, you may need to compare fractions of measurements to ensure accurate dimensions.
- Time Management: Comparing fractions of time can help you prioritize tasks and allocate your time effectively.
By mastering fraction comparison, you’ll be better equipped to make informed decisions and solve problems in a variety of real-world contexts.
11. Common Mistakes to Avoid
- Assuming Larger Denominator Means Larger Fraction: Remember that when numerators are the same, a larger denominator means a smaller fraction.
- Forgetting to Find a Common Denominator: When comparing fractions with different denominators, you must find a common denominator before comparing the numerators.
- Making Errors in LCM Calculation: Double-check your LCM calculations to ensure accuracy.
- Rounding Decimals Too Early: If converting to decimals, avoid rounding too early, as this can introduce significant errors.
- Not Simplifying Fractions: Simplifying fractions before comparing makes the process easier and less prone to errors.
12. Advanced Techniques for Complex Comparisons
For more complex comparisons involving a large number of fractions or fractions with very large denominators, consider using computer-based tools or calculators to assist with calculations. These tools can quickly find common denominators, convert fractions to decimals, and perform other calculations with high precision.
13. Fraction Comparison and Number Sense
Developing a strong number sense is crucial for understanding and working with fractions. Number sense involves having an intuitive understanding of the relative size and relationships between numbers. To develop your number sense with fractions:
- Estimate: Before performing any calculations, try to estimate the relative size of the fractions.
- Visualize: Use visual models to represent fractions and understand their relationships.
- Relate to Benchmarks: Compare fractions to common benchmarks to get a sense of their size.
- Explain Your Reasoning: Articulate your thought process when comparing fractions. This helps solidify your understanding and identify any misconceptions.
14. Educational Resources for Learning Fraction Comparison
Many excellent educational resources are available to help you learn and practice fraction comparison:
- Online Tutorials: Websites like Khan Academy and COMPARE.EDU.VN offer free tutorials and practice exercises on fraction comparison.
- Textbooks: Math textbooks typically cover fraction comparison in detail, with examples and exercises.
- Workbooks: Math workbooks provide additional practice exercises and problem-solving opportunities.
- Interactive Games: Online math games can make learning fraction comparison more engaging and fun.
15. Practice Problems with Step-by-Step Solutions
To solidify your understanding of fraction comparison, work through the following practice problems:
Problem 1:
Compare 2/3, 3/4, and 5/6.
Solution:
- Find the LCM of 3, 4, and 6. The LCM is 12.
- Convert each fraction to an equivalent fraction with a denominator of 12:
- 2/3 = (2 x 4) / (3 x 4) = 8/12
- 3/4 = (3 x 3) / (4 x 3) = 9/12
- 5/6 = (5 x 2) / (6 x 2) = 10/12
- Compare the numerators: 8 < 9 < 10
- Therefore, 2/3 < 3/4 < 5/6
Problem 2:
Compare 1/2, 3/5, and 4/7.
Solution:
- Find the LCM of 2, 5, and 7. The LCM is 70.
- Convert each fraction to an equivalent fraction with a denominator of 70:
- 1/2 = (1 x 35) / (2 x 35) = 35/70
- 3/5 = (3 x 14) / (5 x 14) = 42/70
- 4/7 = (4 x 10) / (7 x 10) = 40/70
- Compare the numerators: 35 < 40 < 42
- Therefore, 1/2 < 4/7 < 3/5
Problem 3:
Compare 5/8, 7/12, and 9/16.
Solution:
- Find the LCM of 8, 12, and 16. The LCM is 48.
- Convert each fraction to an equivalent fraction with a denominator of 48:
- 5/8 = (5 x 6) / (8 x 6) = 30/48
- 7/12 = (7 x 4) / (12 x 4) = 28/48
- 9/16 = (9 x 3) / (16 x 3) = 27/48
- Compare the numerators: 27 < 28 < 30
- Therefore, 9/16 < 7/12 < 5/8
16. Conclusion: Mastering Fraction Comparison
Comparing multiple fractions is a fundamental skill with numerous applications in mathematics and everyday life. By mastering the methods and techniques outlined in this guide, you can confidently compare fractions, solve problems, and make informed decisions. Remember to practice regularly, use visual models, and develop your number sense to become proficient in fraction comparison.
Ready to take your understanding of comparisons to the next level? Visit COMPARE.EDU.VN, where you’ll find a wealth of detailed, objective comparisons across various topics. Whether you’re weighing different products, services, or ideas, our comprehensive analyses provide the insights you need to make the best choice.
17. FAQs About Comparing Multiple Fractions
1. What is the easiest method to compare multiple fractions?
The easiest method depends on the fractions themselves. If they have the same denominator, simply compare the numerators. If they have different denominators, converting them to decimals or finding a common denominator are common approaches.
2. Can I use cross-multiplication to compare more than two fractions?
Cross-multiplication is best suited for comparing two fractions at a time. To compare multiple fractions, you can compare them in pairs, but this method can become cumbersome and prone to errors.
3. What is the least common denominator (LCD) and why is it important?
The least common denominator (LCD) is the least common multiple (LCM) of the denominators of the fractions. It’s important because it allows you to convert fractions to equivalent fractions with the same denominator, making it easy to compare their numerators.
4. How do I find the least common multiple (LCM)?
You can find the LCM by listing multiples of each number until you find a common multiple, or by using prime factorization.
5. What are benchmarks and how can they help in comparing fractions?
Benchmarks are common fractions, such as 0, 1/4, 1/2, 3/4, and 1, that you can use as reference points to quickly estimate the size of other fractions.
6. Is it always necessary to find the exact value of a fraction to compare it?
No, especially when using benchmarks or visual models. Sometimes, you can get a good sense of the relative size of fractions without performing exact calculations.
7. What are some real-world applications of comparing fractions?
Real-world applications include cooking, finance, construction, and time management.
8. What are some common mistakes to avoid when comparing fractions?
Common mistakes include assuming a larger denominator means a larger fraction (when numerators are the same), forgetting to find a common denominator, making errors in LCM calculation, and rounding decimals too early.
9. How can visual models help in understanding and comparing fractions?
Visual models, such as pie charts, bar diagrams, and number lines, provide a geometric representation of fractions, making it easier to see their relative sizes.
10. Where can I find more resources to learn and practice fraction comparison?
You can find more resources on websites like Khan Academy and COMPARE.EDU.VN, in math textbooks and workbooks, and through online math games.
Address: 333 Comparison Plaza, Choice City, CA 90210, United States
Whatsapp: +1 (626) 555-9090
Website: COMPARE.EDU.VN
Explore COMPARE.EDU.VN today and make smarter, more informed decisions. Don’t just compare; understand. Let compare.edu.vn be your guide in a world full of choices.