Comparing fractions with different denominators can seem daunting, but COMPARE.EDU.VN makes it easy to understand and master this essential math skill. This guide will equip you with the knowledge and strategies to confidently compare any fractions, ensuring accuracy and efficiency. Explore effective methods for comparing fractions with unlike denominators.
1. Understanding Fractions: A Quick Review
Before diving into the methods of comparing fractions with different denominators, let’s revisit the basics of what a fraction represents. A fraction is a part of a whole, symbolized by a numerator (the top number) and a denominator (the bottom number). The numerator indicates how many parts of the whole you have, while the denominator indicates how many equal parts the whole is divided into. For example, in the fraction 3/4, 3 is the numerator, and 4 is the denominator. This means you have 3 parts out of a total of 4 equal parts. Understanding this fundamental concept is crucial for grasping how to compare fractions effectively.
2. Why Different Denominators Pose a Challenge
The main challenge in comparing fractions with different denominators arises because the fractions are not expressed in terms of the same “unit” size. Imagine trying to compare apples and oranges directly without converting them into a common unit like “pieces of fruit.” Similarly, fractions with different denominators need to be converted to a common denominator before you can directly compare their numerators. This ensures that you’re comparing portions of the same sized whole.
3. The Key Method: Finding the Least Common Denominator (LCD)
The most common and reliable method for comparing fractions with different denominators is to find the Least Common Denominator (LCD). The LCD is the smallest multiple that the denominators of both fractions share. Once you find the LCD, you can convert each fraction into an equivalent fraction with the LCD as its new denominator.
3.1. How to Find the LCD
There are a couple of ways to find the LCD:
-
Listing Multiples: List the multiples of each denominator until you find a common multiple. The smallest one is the LCD.
- For example, to compare 1/3 and 1/4:
- Multiples of 3: 3, 6, 9, 12, 15…
- Multiples of 4: 4, 8, 12, 16…
- The LCD is 12.
- For example, to compare 1/3 and 1/4:
-
Prime Factorization: Find the prime factorization of each denominator. The LCD is the product of the highest power of each prime factor present in either factorization.
- For example, to compare 5/6 and 3/8:
- Prime factorization of 6: 2 x 3
- Prime factorization of 8: 2 x 2 x 2 = 2³
- LCD = 2³ x 3 = 8 x 3 = 24
- For example, to compare 5/6 and 3/8:
3.2. Converting Fractions to Equivalent Fractions
Once you’ve found the LCD, the next step is to convert each fraction into an equivalent fraction with the LCD as the denominator. To do this, divide the LCD by the original denominator and then multiply both the numerator and the denominator of the original fraction by the result. This ensures that you are scaling the fraction proportionally, maintaining its value while changing its representation.
- Example 1: Comparing 1/3 and 1/4 (LCD = 12)
- For 1/3: 12 ÷ 3 = 4. Multiply both numerator and denominator by 4: (1 x 4) / (3 x 4) = 4/12
- For 1/4: 12 ÷ 4 = 3. Multiply both numerator and denominator by 3: (1 x 3) / (4 x 3) = 3/12
- Example 2: Comparing 5/6 and 3/8 (LCD = 24)
- For 5/6: 24 ÷ 6 = 4. Multiply both numerator and denominator by 4: (5 x 4) / (6 x 4) = 20/24
- For 3/8: 24 ÷ 8 = 3. Multiply both numerator and denominator by 3: (3 x 3) / (8 x 3) = 9/24
Now that both fractions have the same denominator, you can easily compare them by looking at their numerators.
4. Comparing Numerators: The Final Step
After converting the fractions to equivalent fractions with the LCD, the final step is straightforward: compare the numerators. The fraction with the larger numerator is the larger fraction.
- Example 1 (continued): Comparing 4/12 and 3/12. Since 4 > 3, 4/12 > 3/12. Therefore, 1/3 > 1/4.
- Example 2 (continued): Comparing 20/24 and 9/24. Since 20 > 9, 20/24 > 9/24. Therefore, 5/6 > 3/8.
5. Alternative Methods for Comparing Fractions
While finding the LCD is the most reliable method, here are a couple of other approaches you can use, depending on the specific fractions you’re comparing.
5.1. Cross-Multiplication
Cross-multiplication is a shortcut that works well when comparing two fractions. Multiply the numerator of the first fraction by the denominator of the second fraction, and then multiply the numerator of the second fraction by the denominator of the first fraction. Compare the two products. The fraction corresponding to the larger product is the larger fraction.
- Example: Comparing 3/5 and 2/3
- 3 x 3 = 9
- 2 x 5 = 10
- Since 10 > 9, 2/3 > 3/5
5.2. Converting to Decimals
Another method is to convert each fraction to a decimal by dividing the numerator by the denominator. Then, compare the decimal values. This method is especially useful when you have a calculator handy.
- Example: Comparing 1/4 and 2/5
- 1/4 = 0.25
- 2/5 = 0.4
- Since 0.4 > 0.25, 2/5 > 1/4
5.3. Benchmarking
Sometimes, you can compare fractions by comparing them to a common benchmark, such as 1/2 or 1. For example, if one fraction is less than 1/2 and the other is greater than 1/2, you know which one is larger without needing to find the LCD.
- Example: Comparing 3/8 and 5/9
- 3/8 is less than 1/2 (since 3 is less than half of 8)
- 5/9 is greater than 1/2 (since 5 is more than half of 9)
- Therefore, 5/9 > 3/8
6. Real-World Applications
Understanding how to compare fractions with different denominators is useful in many real-life scenarios. Here are a few examples:
- Cooking: When adjusting recipes, you might need to compare fractional amounts of ingredients.
- Shopping: Comparing prices per unit when items are sold in different quantities (e.g., which is cheaper: $3.50 for 1/2 kg of cheese or $6.00 for 3/4 kg of cheese?)
- Time Management: Allocating time to different tasks, such as spending 1/3 of your day working and 2/5 of your day sleeping. Which activity takes up more time?
- Construction: When measuring materials, such as comparing the lengths of two pieces of wood that are 5/8 inch and 7/16 inch thick.
- Sports: Comparing batting averages or other statistics expressed as fractions.
7. Tips and Tricks for Mastering Fraction Comparison
- Practice Regularly: The more you practice, the more comfortable you’ll become with comparing fractions.
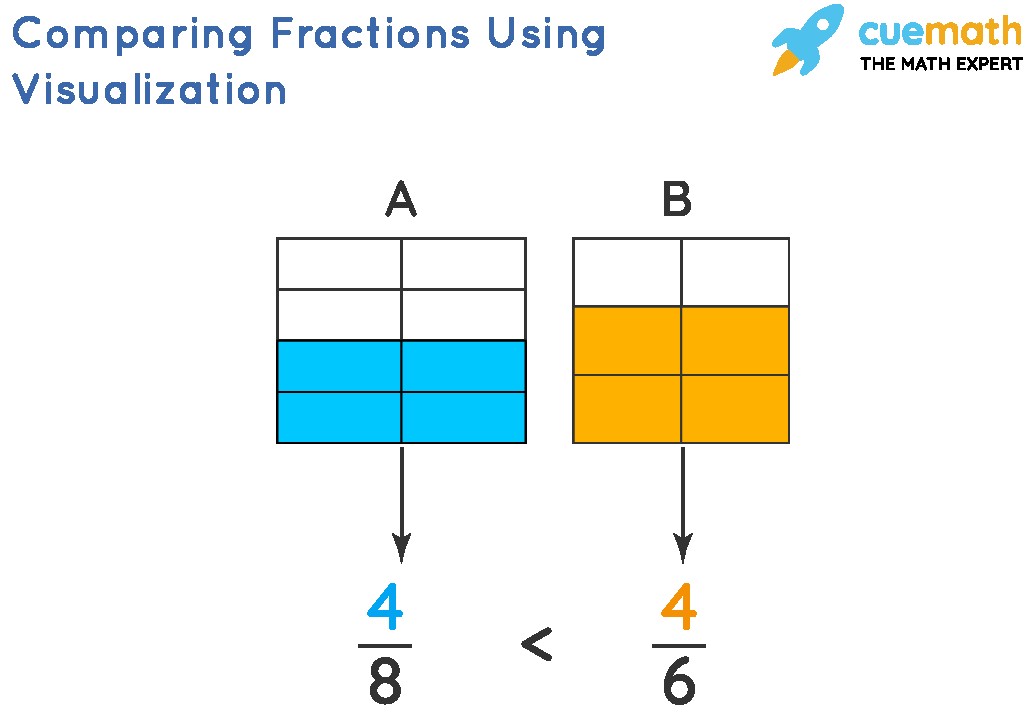
- Use Visual Aids: Drawing diagrams or using fraction bars can help you visualize the fractions and make comparisons easier.
- Check Your Work: Always double-check your calculations, especially when finding the LCD and converting fractions.
- Simplify First: If possible, simplify the fractions before comparing them. This can make the numbers smaller and easier to work with.
- Understand the “Why”: Focus on understanding the underlying concepts rather than just memorizing the steps. This will help you apply the methods correctly in different situations.
8. Common Mistakes to Avoid
- Forgetting to Find a Common Denominator: This is the most common mistake. You cannot accurately compare fractions unless they have the same denominator.
- Incorrectly Finding the LCD: Make sure you find the least common denominator, not just any common denominator. Using a larger common denominator will still work, but it will make the calculations more complicated.
- Only Changing the Denominator: When converting to equivalent fractions, remember to multiply both the numerator and the denominator by the same factor.
- Comparing Fractions with Different Wholes: Ensure that the fractions you’re comparing refer to the same whole. For example, you can’t directly compare 1/2 of a small pizza to 1/4 of a large pizza.
9. Advanced Techniques and Special Cases
9.1. Comparing More Than Two Fractions
The same principles apply when comparing more than two fractions. Find the LCD of all the denominators and convert each fraction to an equivalent fraction with the LCD. Then, compare the numerators to determine the order of the fractions from least to greatest.
- Example: Compare 1/2, 2/5, and 3/8
- LCD of 2, 5, and 8 is 40
- 1/2 = 20/40
- 2/5 = 16/40
- 3/8 = 15/40
- Therefore, 3/8 < 2/5 < 1/2
9.2. Comparing Mixed Numbers
When comparing mixed numbers (e.g., 2 1/3), you can compare the whole number parts first. If the whole number parts are different, the mixed number with the larger whole number is the larger number. If the whole number parts are the same, compare the fractional parts using the methods described above.
- Example: Compare 3 1/4 and 3 2/5
- The whole number parts are the same (3).
- Compare the fractional parts: 1/4 and 2/5
- LCD of 4 and 5 is 20
- 1/4 = 5/20
- 2/5 = 8/20
- Since 8/20 > 5/20, 2/5 > 1/4
- Therefore, 3 2/5 > 3 1/4
9.3. Comparing Improper Fractions
Improper fractions (where the numerator is greater than or equal to the denominator) can be compared using the same methods as proper fractions. Alternatively, you can convert them to mixed numbers and then compare the mixed numbers.
- Example: Compare 5/3 and 7/4
- LCD of 3 and 4 is 12
- 5/3 = 20/12
- 7/4 = 21/12
- Since 21/12 > 20/12, 7/4 > 5/3
10. How COMPARE.EDU.VN Can Help
Comparing fractions with different denominators can be simplified with the right resources. COMPARE.EDU.VN offers detailed guides, examples, and practice problems to improve your skills. Whether you’re a student learning the basics or someone who needs to use fractions in everyday life, COMPARE.EDU.VN is here to help.
11. Conclusion
Mastering how to compare fractions with different denominators is a fundamental skill that opens doors to understanding more complex mathematical concepts. By understanding the underlying principles and practicing the methods described in this guide, you can confidently compare fractions in any situation. So, whether you’re adjusting a recipe, comparing prices, or solving a math problem, you’ll be well-equipped to tackle it with ease.
Remember that the key to success is practice and a solid understanding of the concepts. Don’t be afraid to make mistakes – they are part of the learning process. Keep practicing, and you’ll soon become a fraction comparison expert.
Need more help with comparing fractions or other math topics? Visit COMPARE.EDU.VN for more resources and tools to help you succeed. Our comprehensive guides, examples, and practice problems will make learning math easier and more enjoyable.
Ready to take your fraction skills to the next level? Head over to COMPARE.EDU.VN now and explore our wide range of resources!
For any questions or assistance, feel free to contact us at:
Address: 333 Comparison Plaza, Choice City, CA 90210, United States
Whatsapp: +1 (626) 555-9090
Website: compare.edu.vn
12. FAQs on Comparing Fractions with Different Denominators
Q1: Why can’t I just compare the numerators when the denominators are different?
When denominators differ, fractions don’t represent the same-size pieces. Comparing numerators directly is like comparing apples to oranges; you need a common unit (the common denominator) to make a fair comparison.
Q2: Is finding the LCD always necessary?
While LCD is the most systematic approach, you can sometimes use other methods like cross-multiplication or converting to decimals, especially when dealing with only two fractions.
Q3: What if I use a common denominator that is not the LCD?
Using a common denominator that isn’t the LCD will still lead to the correct comparison, but the numbers will be larger, potentially making the calculations more complex.
Q4: Can I use a calculator to compare fractions?
Yes, converting fractions to decimals using a calculator is a convenient way to compare them, especially for complex fractions.
Q5: How do I compare negative fractions?
With negative fractions, remember that the fraction closer to zero is larger. Convert to a common denominator and compare, keeping in mind the negative sign. For example, -1/3 is greater than -1/2.
Q6: What’s the best way to visualize comparing fractions?
Using fraction bars or pie charts can help visualize the size of fractions and make comparisons more intuitive. Online tools and apps can also be helpful.
Q7: Is cross-multiplication always the fastest method?
Cross-multiplication is quick for two fractions but can be cumbersome with more. LCD is more versatile for multiple fractions.
Q8: How does comparing fractions relate to real-world math?
Comparing fractions is crucial in cooking, measuring, finance, and many other practical applications where proportional relationships matter.
Q9: What if the fractions are part of a more complex equation?
In complex equations, isolate the fractions first, then compare them using the standard methods.
Q10: How can I help my child understand comparing fractions better?
Use real-world examples, hands-on activities (like dividing food), and visual aids to make the concept more concrete and engaging for children.