Comparing equivalent fractions can be straightforward with the right strategies. At COMPARE.EDU.VN, we provide clear and effective methods to help you determine if fractions are equal, greater than, or less than each other. Discover simplified comparison techniques and boost your understanding with our expert insights.
Table of Contents
- Understanding Equivalent Fractions
- Why Compare Equivalent Fractions?
- Methods to Compare Equivalent Fractions
- Step-by-Step Examples
- Advanced Techniques and Tips
- Common Mistakes to Avoid
- Real-World Applications of Comparing Fractions
- Tools and Resources for Comparing Fractions
- The Role of COMPARE.EDU.VN in Fraction Comparisons
- Expert Insights on Fraction Comparison
- Interactive Exercises and Quizzes
- Advanced Topics in Fraction Comparison
- Future Trends in Fraction Education
- Conclusion: Mastering Fraction Comparison with COMPARE.EDU.VN
- Frequently Asked Questions (FAQs)
1. Understanding Equivalent Fractions
Equivalent fractions represent the same value, although they may have different numerators and denominators. Comparing equivalent fractions involves determining whether two or more fractions are equal in value. This skill is fundamental in mathematics and has numerous practical applications. When determining How To Compare Equivalent Fractions, COMPARE.EDU.VN offers insights into foundational concepts that make the process accessible. Understanding how to compare fractional values is essential for grasping more complex mathematical concepts, aiding in everything from basic arithmetic to advanced algebra. Master this skill to enhance your math proficiency.
2. Why Compare Equivalent Fractions?
Comparing equivalent fractions is essential for several reasons. It helps in simplifying mathematical problems, making calculations easier and more efficient. It is also crucial in real-life situations, such as adjusting recipes, managing finances, and understanding data. Mastering the comparison of fractional values is vital for effective problem-solving across various disciplines. This understanding allows for more accurate calculations and informed decision-making in practical scenarios. By learning how to compare fractional values, individuals can enhance their analytical abilities and apply them in diverse contexts.
3. Methods to Compare Equivalent Fractions
There are several methods to effectively compare equivalent fractions. Each technique offers a unique approach, allowing for flexibility and accuracy in determining the equality or difference between fractions. Understanding these methods empowers individuals to tackle a wide range of fraction comparison problems with confidence.
3.1. Simplifying Fractions
Simplifying fractions involves reducing them to their simplest form. Divide both the numerator and denominator by their greatest common factor (GCF). If two fractions simplify to the same form, they are equivalent. Simplification is a fundamental step in the process of comparing and understanding fractions.
3.2. Finding a Common Denominator
To compare fractions with different denominators, find a common denominator. The least common denominator (LCD) is the easiest to work with. Convert each fraction to an equivalent fraction with the common denominator and then compare the numerators. Using a common denominator allows for straightforward comparison of fractions by focusing solely on their numerators.
3.3. Cross-Multiplication Method
The cross-multiplication method is a quick way to compare two fractions. Multiply the numerator of the first fraction by the denominator of the second fraction, and vice versa. Compare the resulting products; if they are equal, the fractions are equivalent. This method provides a direct comparison without the need to find a common denominator.
3.4. Decimal Conversion Method
Convert each fraction to a decimal by dividing the numerator by the denominator. Then, compare the decimal values. If the decimal values are the same, the fractions are equivalent. This method transforms fractions into a familiar format, making comparison simple and intuitive.
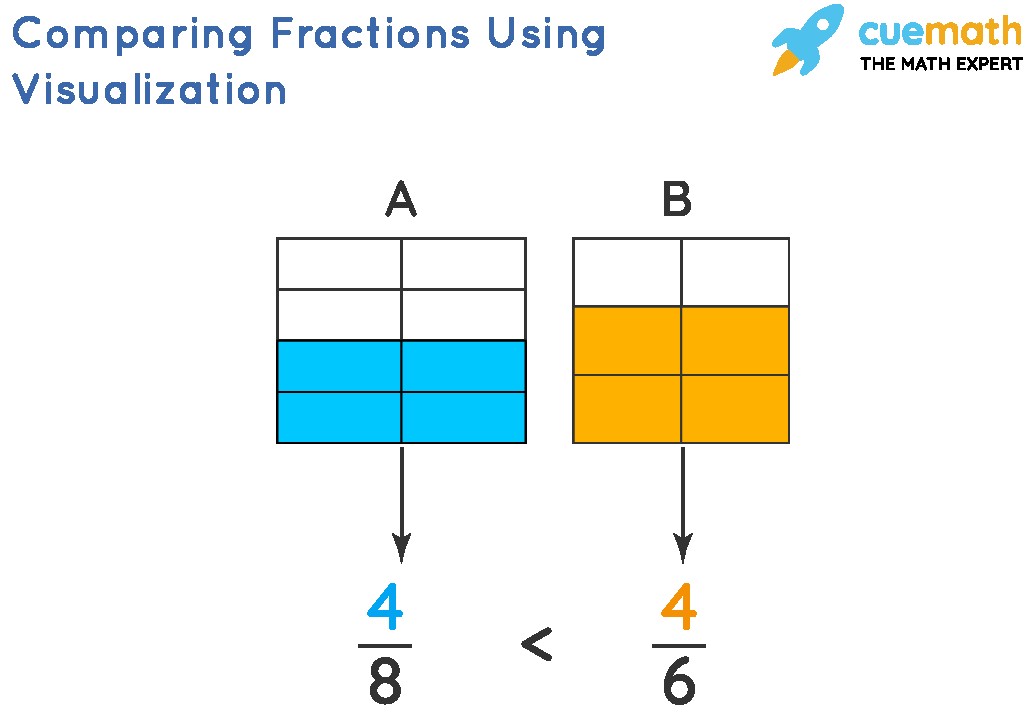
3.5. Visual Comparison Method
Use visual aids, such as pie charts or bar models, to represent the fractions. This method is particularly helpful for understanding and comparing fractions conceptually. By visually representing fractions, it becomes easier to see their relative sizes and determine their equivalence.
4. Step-by-Step Examples
To illustrate each method, let’s walk through several examples. These examples will provide practical insights into how to apply each technique effectively.
4.1. Example 1: Using Simplification
Problem: Determine if 4/8 and 1/2 are equivalent.
Solution:
- Simplify 4/8: Divide both the numerator and denominator by their greatest common factor, which is 4.
- Simplify: 4 ÷ 4 = 1, and 8 ÷ 4 = 2.
- Result: 4/8 simplifies to 1/2.
- Conclusion: Since 4/8 simplifies to 1/2, the fractions are equivalent.
4.2. Example 2: Finding a Common Denominator
Problem: Determine if 2/3 and 4/6 are equivalent.
Solution:
- Find the LCD: The least common denominator of 3 and 6 is 6.
- Convert 2/3 to have a denominator of 6: Multiply both the numerator and denominator by 2.
- Convert: (2 × 2) / (3 × 2) = 4/6.
- Result: 2/3 is equivalent to 4/6.
- Conclusion: Since 2/3 converts to 4/6, the fractions are equivalent.
4.3. Example 3: Applying Cross-Multiplication
Problem: Determine if 3/4 and 6/8 are equivalent.
Solution:
- Cross-multiply: Multiply 3 by 8 and 4 by 6.
- Calculate: 3 × 8 = 24, and 4 × 6 = 24.
- Compare: Both products are equal.
- Conclusion: Since both products are equal, the fractions are equivalent.
4.4. Example 4: Decimal Conversion in Action
Problem: Determine if 1/4 and 0.25 are equivalent.
Solution:
- Convert 1/4 to a decimal: Divide 1 by 4.
- Calculate: 1 ÷ 4 = 0.25.
- Compare: The decimal value of 1/4 is 0.25.
- Conclusion: Since 1/4 converts to 0.25, they are equivalent.
4.5. Example 5: Visualizing Equivalent Fractions
Problem: Determine if 2/4 and 1/2 are equivalent using visual models.
Solution:
- Draw a visual model for 2/4: Divide a rectangle into four equal parts and shade two parts.
- Draw a visual model for 1/2: Divide a rectangle into two equal parts and shade one part.
- Compare: The shaded areas in both models cover the same amount of space.
- Conclusion: Since both visual models represent the same area, the fractions are equivalent.
5. Advanced Techniques and Tips
For more complex fraction comparisons, consider using advanced techniques. These strategies can help in efficiently determining equivalence and relative size.
5.1. Using Benchmarks
Use benchmark fractions like 0, 1/2, and 1 to estimate and compare fractions. Determine whether a fraction is closer to 0, 1/2, or 1 to make quick comparisons. This method provides a relative sense of each fraction’s value.
5.2. Estimating and Approximating
When exact values are not necessary, estimate or approximate fractions to simplify the comparison process. Round fractions to the nearest whole number or benchmark fraction for easier evaluation. Estimation is particularly useful in situations where speed is more important than precision.
5.3. Handling Mixed Numbers
To compare mixed numbers, convert them to improper fractions first. Then, use any of the methods discussed to compare the improper fractions. Converting mixed numbers to improper fractions simplifies the comparison process and ensures accuracy.
5.4. Comparing More Than Two Fractions
To compare more than two fractions, find a common denominator for all fractions and then compare the numerators. This approach ensures a consistent basis for comparison across multiple fractions.
6. Common Mistakes to Avoid
Avoid common mistakes when comparing fractions to ensure accuracy. These errors can lead to incorrect conclusions and misunderstandings.
6.1. Incorrect Simplification
Ensure you are dividing both the numerator and denominator by their greatest common factor. Failing to do so can result in an incorrect simplified fraction. Always double-check your simplification to ensure accuracy.
6.2. Miscalculating the Common Denominator
Double-check your calculations when finding the least common denominator. An incorrect LCD will lead to flawed comparisons. Take the time to verify your LCD to avoid errors.
6.3. Errors in Cross-Multiplication
Ensure you are multiplying the correct numerators and denominators. Reversing the multiplication can lead to incorrect results. Always double-check the cross-multiplication to maintain accuracy.
6.4. Decimal Conversion Errors
Verify your decimal conversions to avoid inaccuracies. Use a calculator or long division to ensure correct results. Accurate decimal conversions are crucial for reliable comparisons.
6.5. Misinterpreting Visual Models
Ensure your visual models accurately represent the fractions being compared. Misinterpreting the models can lead to incorrect conclusions. Pay close attention to the details of your visual representations.
7. Real-World Applications of Comparing Fractions
Comparing fractions has numerous real-world applications across various fields. Understanding these applications highlights the importance of mastering fraction comparisons.
7.1. Cooking and Baking
Recipes often require adjusting ingredient quantities. Comparing fractions helps in scaling recipes accurately. This ensures the correct proportions and outcomes in cooking and baking.
7.2. Construction and Engineering
Measurements in construction and engineering frequently involve fractions. Comparing fractions ensures precise cuts and fits. Accuracy in measurements is crucial for structural integrity and safety.
7.3. Finance and Budgeting
Managing finances involves comparing different expenses and investments. Comparing fractions can help in making informed financial decisions. This ensures efficient budgeting and financial planning.
7.4. Data Analysis and Statistics
Analyzing data often requires comparing fractional parts of a whole. Comparing fractions helps in understanding proportions and trends. Accurate data interpretation relies on precise fraction comparisons.
7.5. Everyday Problem Solving
Everyday situations, like splitting a bill or sharing a pizza, involve comparing fractions. Mastering fraction comparisons enables fair and accurate divisions. This ensures equitable distribution in everyday activities.
8. Tools and Resources for Comparing Fractions
Several tools and resources are available to assist with comparing fractions. These resources can aid in learning, practice, and problem-solving.
8.1. Online Fraction Calculators
Online fraction calculators can quickly compare fractions and provide step-by-step solutions. These tools offer immediate assistance and verification.
8.2. Educational Websites and Apps
Educational websites and apps offer interactive lessons and exercises on comparing fractions. These platforms provide engaging and effective learning experiences.
8.3. Printable Worksheets and Exercises
Printable worksheets and exercises provide opportunities for practice and reinforcement. These resources can be used for homework or self-study.
8.4. Textbooks and Study Guides
Textbooks and study guides offer comprehensive explanations and examples on comparing fractions. These resources provide in-depth knowledge and structured learning.
9. The Role of COMPARE.EDU.VN in Fraction Comparisons
COMPARE.EDU.VN provides resources for understanding and comparing equivalent fractions. Our platform offers comprehensive guides, step-by-step examples, and expert insights. We simplify complex concepts, making learning accessible and effective. At COMPARE.EDU.VN, we are committed to helping you master fraction comparisons.
10. Expert Insights on Fraction Comparison
Gain valuable insights from experts in various fields on the importance and application of comparing fractions. These perspectives highlight the relevance of this skill across different domains.
10.1. From Educators
Educators emphasize that mastering fraction comparisons is crucial for building a strong foundation in mathematics. This skill enables students to tackle more advanced topics with confidence.
10.2. From Mathematicians
Mathematicians highlight that understanding fraction comparisons is essential for mathematical reasoning and problem-solving. This skill is fundamental in various mathematical disciplines.
10.3. From Financial Analysts
Financial analysts point out that accurate fraction comparisons are vital for making informed financial decisions and managing budgets effectively. This skill ensures sound financial planning and management.
11. Interactive Exercises and Quizzes
Engage in interactive exercises and quizzes to test your understanding of comparing fractions. These activities provide immediate feedback and reinforce your learning.
12. Advanced Topics in Fraction Comparison
Explore advanced topics related to fraction comparisons to deepen your understanding and expand your knowledge.
12.1. Fractions and Ratios
Understand the relationship between fractions and ratios and how they are used in comparisons. This knowledge enhances your ability to solve complex problems involving proportions.
12.2. Fractions and Percentages
Learn how to convert fractions to percentages and use them in comparative analysis. This skill is essential for understanding statistical data and financial reports.
12.3. Fractions in Algebra
Explore how fractions are used in algebraic expressions and equations. This knowledge is crucial for solving algebraic problems involving fractional coefficients.
13. Future Trends in Fraction Education
Stay informed about future trends in fraction education, including the use of technology and innovative teaching methods. These trends are shaping the way fractions are taught and learned.
14. Conclusion: Mastering Fraction Comparison with COMPARE.EDU.VN
Mastering how to compare equivalent fractions is essential for success in mathematics and various real-world applications. With the resources and guidance available at COMPARE.EDU.VN, you can develop a strong understanding of fraction comparisons and enhance your problem-solving skills.
15. Frequently Asked Questions (FAQs)
Q1: What does it mean to compare equivalent fractions?
Comparing equivalent fractions means determining if two or more fractions have the same value, even if they have different numerators and denominators.
Q2: Why is comparing fractions important?
Comparing fractions is important because it helps in simplifying mathematical problems, making calculations easier, and understanding proportions in real-life situations.
Q3: What is the easiest method to compare fractions?
One of the easiest methods is to convert fractions to decimals and compare the decimal values.
Q4: How do you compare fractions with different denominators?
To compare fractions with different denominators, find a common denominator and convert each fraction to an equivalent fraction with the common denominator, then compare the numerators.
Q5: What is cross-multiplication?
Cross-multiplication is a method where you multiply the numerator of one fraction by the denominator of the other and compare the resulting products to determine if the fractions are equivalent.
Q6: How can visual models help in comparing fractions?
Visual models, such as pie charts or bar models, can help visualize the fractions, making it easier to see their relative sizes and determine their equivalence.
Q7: What should you do when comparing mixed numbers?
When comparing mixed numbers, convert them to improper fractions first, and then use any of the methods to compare the improper fractions.
Q8: What are some common mistakes to avoid when comparing fractions?
Common mistakes include incorrect simplification, miscalculating the common denominator, errors in cross-multiplication, and decimal conversion errors.
Q9: How are fractions used in real-world applications?
Fractions are used in various real-world applications such as cooking, construction, finance, and data analysis.
Q10: Where can I find reliable resources for learning how to compare fractions?
Reliable resources include online fraction calculators, educational websites and apps, printable worksheets, textbooks, and study guides. COMPARE.EDU.VN also offers comprehensive guides and expert insights.
For more in-depth comparisons and expert advice, visit us at compare.edu.vn or contact us at 333 Comparison Plaza, Choice City, CA 90210, United States. You can also reach us via Whatsapp at +1 (626) 555-9090.