How To Compare Decimals To Fractions: A Simple Guide

Comparing decimals to fractions can seem tricky, but it’s a fundamental skill in mathematics and everyday life. At COMPARE.EDU.VN, we aim to provide you with clear and comprehensive guidance on mastering this skill, offering practical examples and strategies to make the process easy and efficient, enhancing numerical literacy, computation skills, and mathematical proficiency. This guide breaks down the process into simple steps, ensuring you can confidently compare these two types of numbers.

1. Understanding Decimals and Fractions

Before diving into How To Compare Decimals To Fractions, let’s define what each term means:

1.1. What is a Decimal?

A decimal is a number that uses a decimal point to show values less than one. Each digit to the right of the decimal point represents a fraction with a denominator of 10, 100, 1000, and so on. For example:

• 0.1 is one-tenth (1/10)
• 0.25 is twenty-five hundredths (25/100 or 1/4)
• 0.750 is seven hundred fifty thousandths (750/1000 or 3/4)

1.2. What is a Fraction?

A fraction represents a part of a whole. It consists of two parts:

• The numerator (the top number) indicates how many parts you have.
• The denominator (the bottom number) indicates the total number of parts that make up the whole.

For example:

• 1/2 represents one part out of two equal parts.
• 3/4 represents three parts out of four equal parts.
• 5/8 represents five parts out of eight equal parts.

1.3. Why Compare Decimals and Fractions?

Comparing decimals and fractions is essential for various reasons:

• Practical Applications: Everyday tasks such as cooking, measuring, and managing finances often require comparing these two types of numbers.
• Mathematical Proficiency: Understanding how decimals and fractions relate to each other enhances your overall mathematical skills and problem-solving abilities.
• Decision Making: Whether you’re comparing prices, quantities, or proportions, knowing how to compare decimals and fractions helps you make informed decisions.

2. Methods to Compare Decimals and Fractions

There are two primary methods to compare decimals and fractions: converting fractions to decimals and converting decimals to fractions. Each method has its advantages, depending on the numbers you’re working with.

2.1. Method 1: Converting Fractions to Decimals

The most straightforward way to compare a fraction and a decimal is to convert the fraction into a decimal. Here’s how to do it:

2.1.1. Division Method

Divide the numerator of the fraction by its denominator. This will give you the decimal equivalent of the fraction.

Example: Convert 3/4 to a decimal.

• Divide 3 by 4 (3 ÷ 4 = 0.75)
• Therefore, 3/4 = 0.75

2.1.2. Equivalent Fractions Method

If possible, find an equivalent fraction with a denominator of 10, 100, 1000, etc. This makes it easy to write the fraction as a decimal.

Example: Convert 1/2 to a decimal.

• Multiply both the numerator and denominator by 5 to get a denominator of 10: (1 × 5) / (2 × 5) = 5/10
• Therefore, 1/2 = 5/10 = 0.5

2.1.3. Examples of Converting Fractions to Decimals

Let’s look at more examples:

• 1/5: Divide 1 by 5 (1 ÷ 5 = 0.2)
• 2/5: Divide 2 by 5 (2 ÷ 5 = 0.4)
• 7/10: Divide 7 by 10 (7 ÷ 10 = 0.7)
• 1/4: Divide 1 by 4 (1 ÷ 4 = 0.25)
• 3/8: Divide 3 by 8 (3 ÷ 8 = 0.375)

2.1.4. When to Use This Method

This method is particularly useful when you have a fraction that is easy to divide or convert into an equivalent fraction with a denominator that is a power of 10. It simplifies the comparison process by expressing both numbers in the same format.

2.2. Method 2: Converting Decimals to Fractions

Another way to compare decimals and fractions is to convert the decimal into a fraction. Here’s how to do it:

2.2.1. Identify the Place Value

Determine the place value of the last digit in the decimal. This will be your denominator.

Example: Convert 0.6 to a fraction.

• The last digit, 6, is in the tenths place.

2.2.2. Write the Fraction

Write the decimal as a fraction using the identified place value as the denominator.

Example:

• 0.6 = 6/10

2.2.3. Simplify the Fraction

Simplify the fraction to its lowest terms by dividing both the numerator and denominator by their greatest common divisor (GCD).

Example:

• 6/10 can be simplified by dividing both numbers by 2: (6 ÷ 2) / (10 ÷ 2) = 3/5
• Therefore, 0.6 = 3/5

2.2.4. Examples of Converting Decimals to Fractions

Let’s look at more examples:

• 0.75: The last digit is in the hundredths place, so 0.75 = 75/100. Simplify by dividing both numbers by 25: (75 ÷ 25) / (100 ÷ 25) = 3/4
• 0.125: The last digit is in the thousandths place, so 0.125 = 125/1000. Simplify by dividing both numbers by 125: (125 ÷ 125) / (1000 ÷ 125) = 1/8
• 0.2: The last digit is in the tenths place, so 0.2 = 2/10. Simplify by dividing both numbers by 2: (2 ÷ 2) / (10 ÷ 2) = 1/5
• 0.5: The last digit is in the tenths place, so 0.5 = 5/10. Simplify by dividing both numbers by 5: (5 ÷ 5) / (10 ÷ 5) = 1/2
• 0.8: The last digit is in the tenths place, so 0.8 = 8/10. Simplify by dividing both numbers by 2: (8 ÷ 2) / (10 ÷ 2) = 4/5

2.2.5. When to Use This Method

This method works well when the decimal has a clear and finite number of digits. It can be particularly useful when you need to express the decimal as a simple fraction for further calculations or comparisons.

2.3. Comparing Numbers After Conversion

Once you’ve converted either the fraction to a decimal or the decimal to a fraction, you can easily compare the two numbers.

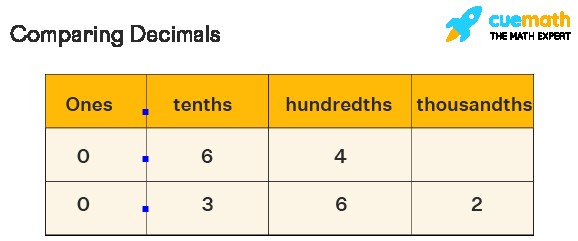
2.3.1. Comparing Decimals

When comparing decimals, align the decimal points and compare the digits from left to right.

Example: Compare 0.75 (which is 3/4) and 0.7.

• Align the decimal points:

  0.75
  0.70 (add a zero to make the number of digits equal)

• Compare the digits from left to right: The tenths place is the same (7), but the hundredths place in 0.75 (5) is greater than that in 0.70 (0).
• Therefore, 0.75 is greater than 0.7.

2.3.2. Comparing Fractions

When comparing fractions, ensure they have the same denominator. Then, compare the numerators.

Example: Compare 3/5 (which is 0.6) and 1/2.

• Find a common denominator (in this case, 10):
  • 3/5 = 6/10
  • 1/2 = 5/10
• Compare the numerators: 6 is greater than 5.
• Therefore, 3/5 is greater than 1/2.

3. Practical Examples

Let’s work through some practical examples to solidify your understanding.

3.1. Example 1: Comparing a Simple Fraction and a Decimal

Problem: Which is larger, 1/4 or 0.2?

Solution:

1. Convert the fraction to a decimal:
   • 1/4 = 0.25 (1 ÷ 4 = 0.25)
2. Compare the decimals:
   • 0.25 > 0.2

Answer: 1/4 is larger than 0.2.

3.2. Example 2: Comparing a More Complex Fraction and a Decimal

Problem: Which is smaller, 3/8 or 0.4?

Solution:

1. Convert the fraction to a decimal:
   • 3/8 = 0.375 (3 ÷ 8 = 0.375)
2. Compare the decimals:
   • 0.375 < 0.4

Answer: 3/8 is smaller than 0.4.

3.3. Example 3: Converting Decimals to Fractions for Comparison

Problem: Which is larger, 0.6 or 2/5?

Solution:

1. Convert the decimal to a fraction:
   • 0.6 = 6/10 = 3/5
2. Compare the fractions:
   • 3/5 > 2/5

Answer: 0.6 is larger than 2/5.

3.4. Example 4: Real-World Application

Problem: You have two measuring cups. One is filled with 0.7 liters of water, and the other is filled with 3/5 liters of water. Which cup has more water?

Solution:

1. Convert the fraction to a decimal:
   • 3/5 = 0.6 (3 ÷ 5 = 0.6)
2. Compare the decimals:
   • 0.7 > 0.6

Answer: The cup with 0.7 liters has more water.

4. Tips and Tricks for Comparing Decimals and Fractions

Here are some useful tips and tricks to make comparing decimals and fractions even easier:

4.1. Use Benchmarks

Memorize common fraction-decimal equivalents like 1/2 = 0.5, 1/4 = 0.25, and 3/4 = 0.75. These benchmarks can help you quickly estimate and compare other values.

4.2. Estimate First

Before doing any calculations, try to estimate the values. This can help you catch any obvious errors and give you a sense of which number should be larger.

4.3. Practice Regularly

The more you practice converting and comparing decimals and fractions, the more comfortable and proficient you’ll become.

4.4. Use Visual Aids

Visual aids like number lines, pie charts, and bar models can be helpful, especially when you’re first learning. These tools provide a concrete representation of the values you’re comparing.

4.5. Simplify When Possible

Always simplify fractions before comparing. This makes the numbers smaller and easier to work with.

5. Common Mistakes to Avoid

When comparing decimals and fractions, it’s easy to make mistakes. Here are some common pitfalls to watch out for:

5.1. Misaligning Decimal Points

When comparing decimals, always align the decimal points. Misalignment can lead to incorrect comparisons.

Example of Incorrect Alignment:

 0.25
1.2

Correct Alignment:

 0.25
 1.20 (add a zero for alignment)

5.2. Ignoring Place Value

Pay close attention to place value. A digit in the tenths place has a different value than a digit in the hundredths place.

5.3. Not Simplifying Fractions

Always simplify fractions to their lowest terms before comparing. This makes the comparison easier and reduces the risk of errors.

5.4. Forgetting to Convert

Make sure to convert either the fraction to a decimal or the decimal to a fraction before comparing. Comparing them in different formats can lead to mistakes.

5.5. Overlooking Negative Signs

When comparing negative decimals and fractions, remember that the number closer to zero is larger. For example, -0.2 is greater than -0.5.

6. Advanced Techniques

For those looking to deepen their understanding, here are some advanced techniques for comparing decimals and fractions.

6.1. Using Percentages

Converting both decimals and fractions to percentages can simplify comparisons, as percentages are essentially fractions with a common denominator of 100.

Example: Compare 0.8 and 3/4.

1. Convert the decimal to a percentage:
   • 0.8 = 80%
2. Convert the fraction to a percentage:
   • 3/4 = 75%
3. Compare the percentages:
   • 80% > 75%
   • Therefore, 0.8 is greater than 3/4.

6.2. Cross-Multiplication

Cross-multiplication is a technique used to compare two fractions. Multiply the numerator of the first fraction by the denominator of the second fraction, and vice versa. Then, compare the results.

Example: Compare 2/3 and 3/4.

1. Cross-multiply:
   • 2 × 4 = 8
   • 3 × 3 = 9
2. Compare the results:
   • 8 < 9
   • Therefore, 2/3 is less than 3/4.

6.3. Using Approximations

In some cases, you can use approximations to quickly compare decimals and fractions, especially when dealing with irrational numbers or complex fractions.

Example: Compare √2/2 and 0.7.

1. Approximate the irrational number:
   • √2 ≈ 1.414
   • √2/2 ≈ 1.414/2 ≈ 0.707
2. Compare the approximations:
   • 0.707 > 0.7
   • Therefore, √2/2 is greater than 0.7.

7. Real-World Applications of Comparing Decimals and Fractions

The ability to compare decimals and fractions is crucial in many real-world scenarios. Here are some examples:

7.1. Cooking and Baking

Recipes often use both fractions and decimals for measurements. Knowing how to compare them ensures you use the correct amounts.

Example: A recipe calls for 0.5 cups of flour and 1/3 cup of sugar. Which ingredient do you need more of?

1. Convert the fraction to a decimal:
   • 1/3 ≈ 0.33
2. Compare the decimals:
   • 0.5 > 0.33
   • You need more flour than sugar.

7.2. Shopping and Finance

Comparing prices, discounts, and interest rates often involves working with decimals and fractions.

Example: A store offers a 20% discount on an item priced at $25. Another store offers 1/4 off the same item. Which store offers a better deal?

1. Calculate the discount in the first store:
   • 20% of $25 = 0.20 × $25 = $5
2. Calculate the discount in the second store:
   • 1/4 of $25 = 0.25 × $25 = $6.25
3. Compare the discounts:
   • $6.25 > $5
   • The second store offers a better deal.

7.3. Construction and Measurement

In construction and measurement, precise calculations are essential, and these often involve decimals and fractions.

Example: A blueprint calls for a beam that is 2.75 meters long. You have a beam that is 2 3/4 meters long. Is it long enough?

1. Convert the mixed number to a decimal:
   • 2 3/4 = 2.75
2. Compare the decimals:
   • 2.75 = 2.75
   • The beam is exactly long enough.

7.4. Science and Engineering

Scientists and engineers frequently use decimals and fractions to represent measurements, ratios, and proportions.

Example: An experiment requires a solution that is 0.6 parts water and 2/5 parts acid. Which component is more concentrated in the solution?

1. Convert the fraction to a decimal:
   • 2/5 = 0.4
2. Compare the decimals:
   • 0.6 > 0.4
   • Water is more concentrated in the solution.

8. Resources for Further Learning

To continue improving your skills in comparing decimals and fractions, here are some valuable resources:

8.1. Online Tutorials and Courses

• Khan Academy: Offers comprehensive lessons and practice exercises on decimals and fractions.
• Coursera and edX: Provide courses on basic math skills that cover comparing decimals and fractions.
• YouTube: Channels like MathAntics and PatrickJMT offer clear explanations and examples.

8.2. Practice Websites and Apps

• Math Playground: Offers interactive games and activities for practicing decimal and fraction comparisons.
• Prodigy Math: A game-based learning platform that includes exercises on comparing numbers.
• IXL Math: Provides comprehensive practice exercises aligned with various math standards.

8.3. Textbooks and Workbooks

• Basic Mathematics by Serge Lang: A classic textbook that covers fundamental math concepts, including decimals and fractions.
• Math Practice Workbook by Chris McMullen: Offers a wide range of practice problems for improving math skills.

8.4. Tools and Calculators

• Online Fraction Calculators: Help convert fractions to decimals and simplify fractions.
• Decimal Comparison Tools: Allow you to compare decimals and fractions quickly and accurately.

9. Conclusion: Mastering Decimals and Fractions

Comparing decimals to fractions is a fundamental skill that enhances your mathematical proficiency and problem-solving abilities. By understanding the basic definitions, learning the conversion methods, and practicing regularly, you can confidently compare these two types of numbers in various real-world scenarios. Remember to avoid common mistakes, use helpful tips and tricks, and explore advanced techniques to deepen your understanding.

At COMPARE.EDU.VN, we are committed to providing you with the tools and knowledge you need to succeed. Whether you’re a student, a professional, or simply someone looking to improve your math skills, mastering decimals and fractions will undoubtedly benefit you in numerous ways. Keep practicing, stay curious, and continue exploring the fascinating world of mathematics.

Ready to put your skills to the test? Visit compare.edu.vn for more practice problems, quizzes, and resources to help you master comparing decimals and fractions. Make informed decisions with confidence! Contact us at 333 Comparison Plaza, Choice City, CA 90210, United States, or reach out via WhatsApp at +1 (626) 555-9090.

10. Frequently Asked Questions (FAQs)

10.1. What is the easiest way to compare decimals and fractions?

The easiest way to compare decimals and fractions is to convert the fraction to a decimal by dividing the numerator by the denominator. Then, compare the two decimals by aligning the decimal points and comparing the digits from left to right.

10.2. How do you convert a fraction to a decimal?

To convert a fraction to a decimal, divide the numerator (the top number) by the denominator (the bottom number). For example, to convert 1/4 to a decimal, divide 1 by 4, which equals 0.25.

10.3. How do you convert a decimal to a fraction?

To convert a decimal to a fraction, identify the place value of the last digit in the decimal. Write the decimal as a fraction using that place value as the denominator. Then, simplify the fraction to its lowest terms. For example, 0.75 can be written as 75/100, which simplifies to 3/4.

10.4. What are some common fraction-decimal equivalents that are useful to memorize?

Some common fraction-decimal equivalents include:

• 1/2 = 0.5
• 1/4 = 0.25
• 3/4 = 0.75
• 1/5 = 0.2
• 2/5 = 0.4
• 3/5 = 0.6
• 4/5 = 0.8
• 1/10 = 0.1

10.5. How do you compare two fractions with different denominators?

To compare two fractions with different denominators, find a common denominator for both fractions. Then, rewrite each fraction with the common denominator and compare the numerators. The fraction with the larger numerator is the larger fraction.

10.6. What is cross-multiplication, and how is it used to compare fractions?

Cross-multiplication is a technique used to compare two fractions. Multiply the numerator of the first fraction by the denominator of the second fraction, and vice versa. Then, compare the results. The fraction corresponding to the larger result is the larger fraction. For example, to compare 2/3 and 3/4, multiply 2 × 4 = 8 and 3 × 3 = 9. Since 9 is greater than 8, 3/4 is greater than 2/3.

10.7. How can visual aids help in comparing decimals and fractions?

Visual aids such as number lines, pie charts, and bar models can provide a concrete representation of decimals and fractions, making it easier to compare their values. These tools help visualize the relative sizes of the numbers and can be particularly helpful for learners who are new to the concepts.

10.8. What should I do if I have trouble comparing decimals and fractions?

If you have trouble comparing decimals and fractions, start by reviewing the basic definitions and conversion methods. Practice regularly with simple examples, and gradually increase the complexity of the problems. Use online resources, textbooks, and visual aids to reinforce your understanding. If needed, seek help from a tutor or math teacher.

10.9. How does comparing decimals and fractions relate to percentages?

Comparing decimals and fractions relates to percentages because percentages are essentially fractions with a common denominator of 100. Converting decimals and fractions to percentages can simplify comparisons, as you are then comparing numbers with the same base. For example, 0.75 is equal to 75%, and 3/4 is also equal to 75%.

10.10. Can calculators be used for comparing decimals and fractions?

Yes, calculators can be a useful tool for comparing decimals and fractions. You can use a calculator to convert fractions to decimals and then compare the resulting decimals. However, it’s important to understand the underlying concepts and methods so that you can perform these comparisons even without a calculator.