Comparing 3 fractions with different denominators can seem daunting, but it’s a straightforward process when you understand the underlying principles. This comprehensive guide, brought to you by COMPARE.EDU.VN, will break down the steps, offering various methods and practical examples to master this essential mathematical skill. Learn effective strategies and make informed comparisons today.
1. Understanding Fractions and Their Components
Before diving into the comparison of three fractions, it’s essential to grasp the fundamentals of what a fraction represents. A fraction is a numerical quantity that is not a whole number. It represents a part of a whole and consists of two primary components: the numerator and the denominator.
1.1 The Numerator: Identifying the Parts
The numerator is the number located above the fraction bar. It indicates the number of parts we are considering out of the whole. For example, in the fraction 3/4, the numerator is 3, signifying that we are looking at 3 parts.
1.2 The Denominator: Defining the Whole
The denominator is the number found below the fraction bar. It represents the total number of equal parts into which the whole is divided. In the fraction 3/4, the denominator is 4, indicating that the whole is divided into 4 equal parts.
1.3 Types of Fractions: Preparing for Comparison
Different types of fractions exist, each with its own characteristics that can influence the comparison process:
- Proper Fractions: The numerator is less than the denominator (e.g., 2/5).
- Improper Fractions: The numerator is greater than or equal to the denominator (e.g., 7/3).
- Mixed Numbers: A whole number combined with a proper fraction (e.g., 1 1/2).
Understanding these types is the first step in learning how to compare fractions effectively.
2. The Challenge of Different Denominators
The main challenge in comparing fractions arises when they have different denominators. It’s difficult to directly compare fractions like 1/2, 1/3, and 1/4 because the “wholes” are divided into different numbers of parts. This is where the process of finding a common denominator becomes crucial.
2.1 Why Common Denominators Matter
Using a common denominator allows us to express each fraction in terms of the same “whole.” Once the denominators are the same, we can easily compare the fractions by simply looking at their numerators. The fraction with the larger numerator is the larger fraction.
2.2 Introducing the Least Common Multiple (LCM)
The Least Common Multiple (LCM) is the smallest multiple that is common to two or more numbers. In the context of fractions, the LCM of the denominators is the ideal common denominator to use because it simplifies the fractions as much as possible.
2.3 Finding the LCM: Prime Factorization Method
One of the most reliable methods for finding the LCM is prime factorization. Here’s how it works:
- Prime Factorization: Break down each denominator into its prime factors.
- Identify Common Factors: Identify the common prime factors among the denominators.
- Calculate the LCM: Multiply the highest powers of all prime factors found in the factorizations.
For example, let’s find the LCM of 4, 6, and 10:
- 4 = 2^2
- 6 = 2 x 3
- 10 = 2 x 5
LCM (4, 6, 10) = 2^2 x 3 x 5 = 60
3. Step-by-Step Guide: Comparing Three Fractions
Now, let’s outline the process for comparing three fractions with different denominators, incorporating the concept of the LCM.
3.1 Step 1: Identify the Fractions
Clearly identify the three fractions you want to compare. For this example, let’s use 2/3, 3/4, and 5/6.
3.2 Step 2: Find the Least Common Multiple (LCM)
Determine the LCM of the denominators (3, 4, and 6).
- 3 = 3
- 4 = 2^2
- 6 = 2 x 3
LCM (3, 4, 6) = 2^2 x 3 = 12
3.3 Step 3: Convert Fractions to Equivalent Fractions
Convert each fraction into an equivalent fraction with the LCM as the new denominator:
- 2/3 = (2 x 4) / (3 x 4) = 8/12
- 3/4 = (3 x 3) / (4 x 3) = 9/12
- 5/6 = (5 x 2) / (6 x 2) = 10/12
3.4 Step 4: Compare the Numerators
Now that the fractions have the same denominator, you can compare the numerators:
- 8/12, 9/12, 10/12
Clearly, 8 < 9 < 10.
3.5 Step 5: Conclude the Comparison
Based on the comparison of the numerators, you can determine the order of the original fractions:
- 2/3 < 3/4 < 5/6
4. Alternative Methods for Comparing Fractions
While finding the LCM is a reliable method, there are other approaches you can use to compare fractions, especially when dealing with only two or three fractions.
4.1 Cross-Multiplication: Quick Comparisons
Cross-multiplication is a quick method for comparing two fractions. To compare a/b and c/d, cross-multiply and compare the products:
- If a x d > b x c, then a/b > c/d
- If a x d < b x c, then a/b < c/d
- If a x d = b x c, then a/b = c/d
This method works well for comparing two fractions but becomes cumbersome with three or more.
4.2 Decimal Conversion: Using Decimals for Comparison
Another method is to convert each fraction into a decimal. Divide the numerator by the denominator to get the decimal equivalent, and then compare the decimal values.
- 2/3 ≈ 0.667
- 3/4 = 0.75
- 5/6 ≈ 0.833
Comparing the decimals, we get 0.667 < 0.75 < 0.833, which confirms that 2/3 < 3/4 < 5/6.
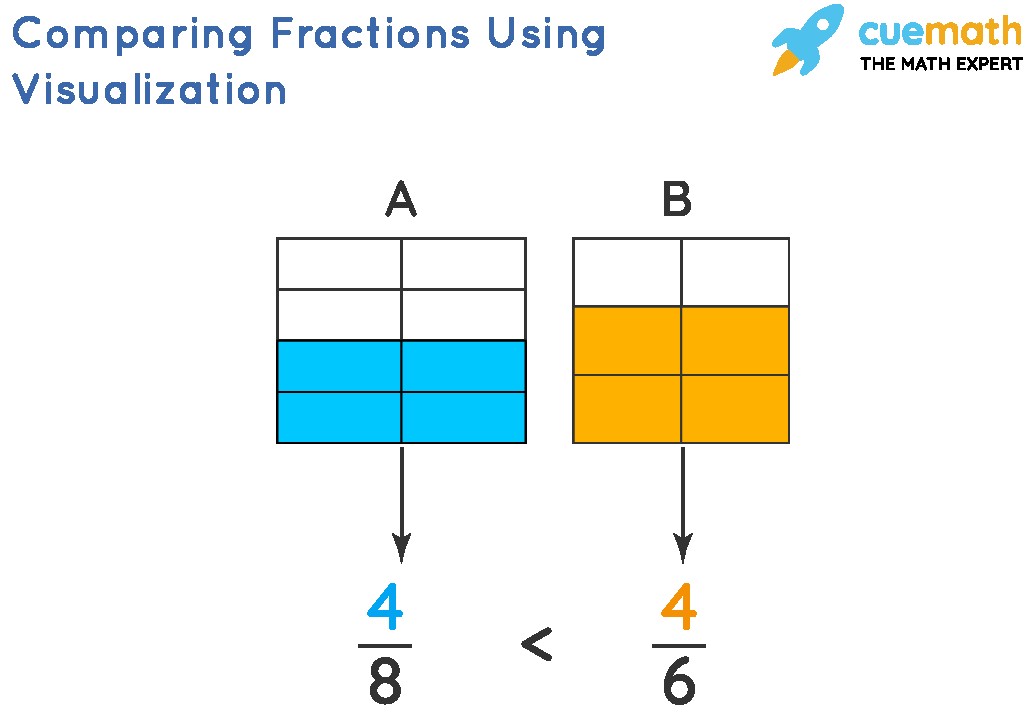
Visual representation of comparing fractions, showing how different fractions occupy different amounts of space.
5. Real-World Applications of Comparing Fractions
Understanding how to compare fractions is not just an academic exercise. It has numerous practical applications in everyday life.
5.1 Cooking and Baking: Adjusting Recipes
In cooking and baking, recipes often involve fractional amounts of ingredients. Knowing how to compare fractions allows you to adjust recipes accurately, whether you’re doubling a recipe or scaling it down.
5.2 Measuring and Construction: Ensuring Accuracy
In fields like construction and carpentry, precise measurements are crucial. Fractions are commonly used in measurements, and the ability to compare them ensures that materials are cut and assembled correctly.
5.3 Financial Planning: Comparing Investments
When comparing investment options, you might encounter fractional returns or interest rates. Being able to compare these fractions helps you make informed decisions about where to allocate your money.
5.4 Time Management: Allocating Time Effectively
Dividing your time among various tasks often involves working with fractions. Comparing fractions of time can help you allocate your schedule efficiently, ensuring that you dedicate enough time to each activity.
6. Advanced Techniques: Handling Complex Fractions
As you become more comfortable with comparing fractions, you may encounter more complex scenarios that require advanced techniques.
6.1 Comparing Mixed Numbers: Combining Whole Numbers and Fractions
To compare mixed numbers, first compare the whole number parts. If the whole numbers are different, the mixed number with the larger whole number is greater. If the whole numbers are the same, compare the fractional parts using the methods described earlier.
For example, compare 3 1/4 and 3 2/5:
- The whole numbers are the same (3).
- Compare the fractions 1/4 and 2/5.
- 1/4 = 5/20 and 2/5 = 8/20.
- Since 8/20 > 5/20, 3 2/5 > 3 1/4.
6.2 Comparing Improper Fractions: Converting to Mixed Numbers
Improper fractions can be compared by converting them to mixed numbers. Once they are in mixed number form, you can compare the whole number parts and then the fractional parts, as described above.
For example, compare 7/3 and 9/4:
- 7/3 = 2 1/3
- 9/4 = 2 1/4
- The whole numbers are the same (2).
- Compare the fractions 1/3 and 1/4.
- 1/3 = 4/12 and 1/4 = 3/12.
- Since 4/12 > 3/12, 7/3 > 9/4.
6.3 Comparing Fractions with Negative Signs: Understanding Negative Values
When comparing fractions with negative signs, remember that the fraction closer to zero is greater. For example, -1/2 is greater than -3/4 because -1/2 is closer to zero on the number line.
7. Common Mistakes to Avoid When Comparing Fractions
Even with a solid understanding of the methods, it’s easy to make mistakes when comparing fractions. Here are some common pitfalls to avoid:
7.1 Assuming Numerators Alone Determine Size
A common mistake is to assume that the fraction with the larger numerator is always the larger fraction, without considering the denominators. Remember that the denominator plays a crucial role in determining the size of a fraction.
7.2 Incorrectly Finding the LCM
Errors in finding the LCM can lead to incorrect comparisons. Double-check your prime factorizations and calculations to ensure you have the correct LCM.
7.3 Not Simplifying Fractions First
Sometimes, simplifying fractions before comparing them can make the process easier. Look for common factors in the numerator and denominator and reduce the fraction to its simplest form before proceeding.
7.4 Misinterpreting Decimal Values
When using the decimal conversion method, ensure you correctly interpret the decimal values. Pay attention to place values and rounding to avoid errors in comparison.
An illustration of cross multiplication, demonstrating how to quickly compare two fractions.
8. Practice Exercises: Sharpening Your Skills
To reinforce your understanding of comparing fractions, here are some practice exercises. Work through these problems, applying the methods and techniques discussed in this guide.
8.1 Exercise 1: Comparing Proper Fractions
Compare the following fractions:
- 1/3, 2/5, 3/8
- 2/7, 3/10, 4/15
8.2 Exercise 2: Comparing Improper Fractions
Compare the following fractions:
- 5/2, 7/3, 9/4
- 8/5, 11/7, 13/8
8.3 Exercise 3: Comparing Mixed Numbers
Compare the following mixed numbers:
- 2 1/4, 2 2/5, 2 3/10
- 3 1/3, 3 2/7, 3 4/15
8.4 Exercise 4: Real-World Application
John spends 1/4 of his day working, 1/6 of his day exercising, and 3/8 of his day sleeping. Order these activities from least to most time spent.
9. Frequently Asked Questions (FAQs)
To address common questions and misconceptions about comparing fractions, here’s a comprehensive FAQ section.
9.1 What is the Importance of Comparing Fractions?
Comparing fractions is crucial for developing a strong number sense and understanding the relative sizes of different quantities. It is a fundamental skill that is applied in various real-world scenarios, from cooking to financial planning.
9.2 How Do I Compare Fractions with the Same Denominator?
When fractions have the same denominator, simply compare the numerators. The fraction with the larger numerator is the larger fraction.
9.3 Can I Always Use Cross-Multiplication?
Cross-multiplication is a useful shortcut for comparing two fractions. However, it can become cumbersome when comparing three or more fractions. In such cases, finding the LCM is a more efficient method.
9.4 What If I Have a Mix of Proper and Improper Fractions?
Convert improper fractions to mixed numbers. Then, compare the whole number parts first. If the whole numbers are the same, compare the fractional parts.
9.5 Is There a Quick Way to Estimate Fractions Without Exact Calculations?
Yes, you can estimate fractions by rounding them to the nearest whole number or common benchmark (e.g., 0, 1/2, 1). This can give you a rough idea of their relative sizes without performing exact calculations.
9.6 How Does Comparing Fractions Relate to Percentages?
Fractions, decimals, and percentages are all interconnected. Comparing fractions is similar to comparing percentages because both represent parts of a whole. Converting fractions to percentages (or vice versa) can make comparisons easier in some cases.
9.7 What Are Equivalent Fractions, and How Do They Help in Comparing?
Equivalent fractions are fractions that have the same value but different numerators and denominators (e.g., 1/2 and 2/4). Converting fractions to equivalent forms with a common denominator is a key step in comparing fractions with different denominators.
9.8 How Do I Compare Fractions with Negative Signs?
When comparing negative fractions, remember that the fraction closer to zero is greater. You can also think of it as comparing the absolute values of the fractions and then reversing the inequality sign.
9.9 Can I Use a Calculator to Compare Fractions?
Yes, you can use a calculator to convert fractions to decimals and then compare the decimal values. This can be a quick and accurate method, especially for complex fractions.
9.10 Where Can I Find More Resources on Comparing Fractions?
COMPARE.EDU.VN offers a wealth of resources, including detailed guides, examples, and practice exercises to help you master the art of comparing fractions. Additionally, you can find helpful materials in textbooks, online math tutorials, and educational websites.
10. Conclusion: Mastering Fraction Comparison
Comparing fractions with different denominators is a fundamental skill that is essential for success in mathematics and various real-world applications. By understanding the underlying principles, mastering the methods, and practicing regularly, you can confidently compare fractions and make informed decisions based on their relative sizes. Remember to identify the fractions, find the LCM, convert to equivalent fractions, compare the numerators, and draw your conclusion.
COMPARE.EDU.VN is your go-to resource for mastering comparisons across various subjects. Whether you’re comparing fractions, financial investments, or consumer products, we provide the tools and knowledge you need to make informed decisions.
Ready to make confident comparisons? Visit COMPARE.EDU.VN today and explore our comprehensive resources. Make informed decisions with ease.
Contact us:
Address: 333 Comparison Plaza, Choice City, CA 90210, United States
WhatsApp: +1 (626) 555-9090
Website: compare.edu.vn
Navigate life’s choices with clarity and confidence – start comparing today!