Comparing decimals can seem tricky, but with a clear understanding of place value and a systematic approach, it becomes a straightforward process. This guide, brought to you by COMPARE.EDU.VN, provides a detailed exploration of how to compare decimal numbers effectively. Learn how to compare decimals with confidence.
1. Understanding the Basics of Decimal Comparison
Comparing decimals is about determining which decimal number has a greater value. This involves examining the digits in each place value position, starting from the left and moving towards the right. Just like comparing whole numbers, we need to consider the magnitude each digit represents. For a deeper dive and more comparisons, explore COMPARE.EDU.VN.
1.1. What Does It Mean to Compare Decimals?
At its core, comparing decimals is similar to comparing any other number. The key is to understand that each digit after the decimal point represents a fraction of one, with the first digit being tenths, the second hundredths, the third thousandths, and so on. When comparing decimals, you are essentially comparing these fractional parts to determine which number is larger or smaller. This skill is essential for making informed decisions in various real-world scenarios. For example, consider prices at the grocery store or measurements in a science experiment, this comparison is the bedrock of these types of decisions. You can find more practical examples on COMPARE.EDU.VN.
1.2. The Importance of Place Value
Place value is critical to comparing decimals. Each position to the right of the decimal point represents a decreasing power of ten. The first digit is the tenths place (1/10), the second is the hundredths place (1/100), the third is the thousandths place (1/1000), and so on. Understanding the place value of each digit helps determine its contribution to the overall value of the decimal. For instance, 0.3 is greater than 0.09 because 3 tenths is more significant than 9 hundredths. For interactive tools to practice place value, visit COMPARE.EDU.VN.
1.3. Common Mistakes to Avoid
A common mistake when comparing decimals is focusing solely on the number of digits after the decimal point. For example, some might incorrectly assume that 0.123 is greater than 0.4 because 0.123 has more digits. However, 0.4 is actually larger because 4 tenths is greater than 1 tenth. Always start by comparing the digits in the largest place value position first and move to the right only if those digits are equal. Another mistake is not aligning the decimal points correctly, which can lead to misinterpretation of the place values. Stay informed and avoid these errors with resources at COMPARE.EDU.VN.
2. Methods for Comparing Decimals
There are several methods for comparing decimals, each offering a slightly different approach. Here are the most common and effective techniques.
2.1. The Place Value Chart Method
The place value chart method involves aligning the decimal numbers in a chart that clearly shows each place value position. This method helps visually organize the numbers and makes it easier to compare the digits in each position. Start by writing the numbers in the chart, aligning the decimal points. Then, compare the digits from left to right, starting with the largest place value position. This method is particularly helpful for beginners as it provides a clear, structured way to compare decimals. Discover more educational charts and resources at COMPARE.EDU.VN.
Example: Compare 2.345 and 2.354
| Place Value | Ones | Tenths | Hundredths | Thousandths |
|---|---|---|---|---|
| 2.345 | 2 | 3 | 4 | 5 |
| 2.354 | 2 | 3 | 5 | 4 |
In this case, the ones and tenths places are the same. However, in the hundredths place, 5 is greater than 4. Therefore, 2.354 is greater than 2.345.
2.2. Adding Zeros as Placeholders
Adding zeros as placeholders can simplify the comparison process, especially when the decimals have a different number of digits after the decimal point. By adding zeros to the end of the shorter decimal, you make both decimals have the same number of digits, making it easier to compare them. Remember that adding zeros to the right of the last digit after the decimal point does not change the value of the number. This method is useful for ensuring you are comparing digits in the correct place value positions. Explore more tips and tricks at COMPARE.EDU.VN.
Example: Compare 0.6 and 0.58
To compare, add a zero to 0.6 to make it 0.60. Now compare 0.60 and 0.58. Since 60 hundredths is greater than 58 hundredths, 0.6 is greater than 0.58.
2.3. Converting Decimals to Fractions
Converting decimals to fractions can provide another way to compare decimal numbers, especially if you are more comfortable working with fractions. To convert a decimal to a fraction, write the decimal as a fraction with a denominator of 10, 100, 1000, or whatever power of ten corresponds to the number of digits after the decimal point. Then, simplify the fraction if possible. Once you have both numbers as fractions, compare the fractions using the rules for comparing fractions. This method can be helpful for understanding the underlying values of the decimals. Learn more about decimal-to-fraction conversions at COMPARE.EDU.VN.
Example: Compare 0.75 and 0.8
Convert 0.75 to 75/100, which simplifies to 3/4. Convert 0.8 to 8/10, which simplifies to 4/5. To compare 3/4 and 4/5, find a common denominator, which is 20. 3/4 becomes 15/20 and 4/5 becomes 16/20. Since 16/20 is greater than 15/20, 0.8 is greater than 0.75.
2.4. Using a Number Line
A number line is a visual tool that can help you compare decimals by representing them as points on a line. To use a number line, draw a line and mark the decimals on it in their appropriate positions. The decimal that is further to the right on the number line is the larger number. This method is particularly useful for students who are visual learners. Discover how to use this method effectively at COMPARE.EDU.VN.
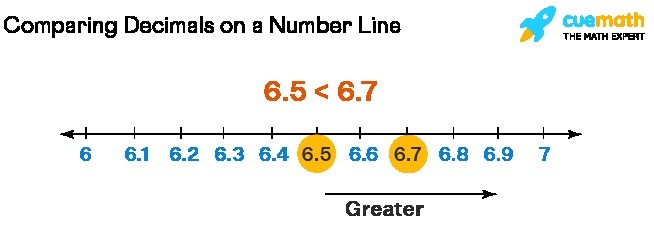Comparing decimals on number line
Example: Compare 3.2 and 3.5
On a number line, 3.5 would be to the right of 3.2, indicating that 3.5 is greater than 3.2.
3. Step-by-Step Guide to Comparing Decimals
Here’s a detailed, step-by-step process for comparing decimals:
3.1. Step 1: Align the Decimal Points
The first step in comparing decimals is to align the decimal points vertically. This ensures that you are comparing digits in the same place value positions. Write the numbers one above the other, making sure the decimal points are in a straight line. This alignment is crucial for accurate comparison. For visual aids and examples, visit COMPARE.EDU.VN.
Example:
12.45
9.873.2. Step 2: Add Zeros as Placeholders (if needed)
If the decimals have a different number of digits after the decimal point, add zeros to the end of the shorter decimal so that both decimals have the same number of digits. This does not change the value of the decimal but makes it easier to compare the digits. This step is particularly helpful when one decimal has fewer digits than the other. Learn why this works mathematically at COMPARE.EDU.VN.
Example: Compare 5.6 and 5.678
Add zeros to 5.6 to make it 5.600. Now you can compare 5.600 and 5.678 more easily.
3.3. Step 3: Compare the Whole Number Part
Compare the whole number parts of the decimals. If the whole number parts are different, the decimal with the larger whole number is the larger decimal. This is the quickest way to determine which decimal is larger if the whole numbers differ. Find practice problems at COMPARE.EDU.VN.
Example: Compare 15.23 and 12.89
Since 15 is greater than 12, 15.23 is greater than 12.89.
3.4. Step 4: Compare the Tenths Place
If the whole number parts are the same, compare the digits in the tenths place (the first digit to the right of the decimal point). The decimal with the larger digit in the tenths place is the larger decimal. This step is crucial when the whole numbers are identical, and you need to move to the fractional part of the number. For more details, check COMPARE.EDU.VN.
Example: Compare 7.45 and 7.32
The whole number parts are the same (7). Comparing the tenths place, 4 is greater than 3. Therefore, 7.45 is greater than 7.32.
3.5. Step 5: Compare the Hundredths Place (if needed)
If the tenths places are also the same, move to the hundredths place (the second digit to the right of the decimal point) and compare the digits. The decimal with the larger digit in the hundredths place is the larger decimal. Continue this process for each subsequent place value until you find a difference. Enhance your skills with quizzes at COMPARE.EDU.VN.
Example: Compare 2.567 and 2.561
The whole number and tenths places are the same (2.5). Comparing the hundredths place, 7 is greater than 1. Therefore, 2.567 is greater than 2.561.
3.6. Step 6: Continue Comparing Place Values as Needed
Continue comparing the digits in each subsequent place value position (thousandths, ten-thousandths, etc.) until you find a difference. The decimal with the larger digit in the first place where the digits differ is the larger decimal. This iterative process ensures you account for even the smallest differences in value. Improve your accuracy with detailed examples at COMPARE.EDU.VN.
Example: Compare 9.1234 and 9.1235
The whole number, tenths, hundredths, and thousandths places are the same (9.123). Comparing the ten-thousandths place, 5 is greater than 4. Therefore, 9.1235 is greater than 9.1234.
4. Comparing Decimals with Different Numbers of Decimal Places
Comparing decimals with different numbers of decimal places can be tricky, but with the right approach, it becomes simple. The key is to ensure that both numbers have the same number of digits after the decimal point before comparing them.
4.1. Why Adding Zeros Works
Adding zeros to the end of a decimal number does not change its value because you are adding zeros in place value positions that are already “empty”. For example, 0.5 is the same as 0.50 and 0.500. Adding these zeros simply clarifies the place value without altering the quantity represented. Understand the mathematical principles at COMPARE.EDU.VN.
Example: 0.5 = 5/10 = 50/100 = 500/1000
4.2. Examples of Comparing Decimals with Different Numbers of Decimal Places
Example 1: Compare 0.8 and 0.75
Add a zero to 0.8 to make it 0.80. Now compare 0.80 and 0.75. Since 80 hundredths is greater than 75 hundredths, 0.8 is greater than 0.75.
Example 2: Compare 1.25 and 1.3
Add a zero to 1.3 to make it 1.30. Now compare 1.25 and 1.30. Since 30 hundredths is greater than 25 hundredths, 1.3 is greater than 1.25.
Example 3: Compare 4.5 and 4.567
Add zeros to 4.5 to make it 4.500. Now compare 4.500 and 4.567. Since 567 thousandths is greater than 500 thousandths, 4.567 is greater than 4.5.
5. Real-World Applications of Comparing Decimals
Comparing decimals is not just a mathematical exercise; it has numerous practical applications in everyday life.
5.1. Shopping and Finance
In shopping, comparing decimals helps you determine which product is cheaper or offers better value. For example, if one item costs $2.45 and another costs $2.50, comparing the decimals allows you to see that the first item is cheaper. In finance, decimals are used to represent interest rates, stock prices, and currency exchange rates. Being able to compare these decimals is essential for making informed financial decisions. Make smarter purchase decisions with insights from COMPARE.EDU.VN.
/dotdash_Final_Comparing_Prices_Oct_2020-01-7dd90c6d615a4384a8b3b4901772ad99.jpg)
5.2. Cooking and Measurement
In cooking, recipes often call for ingredients measured in decimals, such as 2.5 cups of flour or 0.75 teaspoons of salt. Comparing these measurements ensures accuracy in your recipes. Similarly, in other types of measurement, such as construction or scientific experiments, comparing decimals is critical for precision. Avoid kitchen mishaps with tips from COMPARE.EDU.VN.
5.3. Sports and Performance
In sports, decimals are used to measure times, distances, and scores. For example, a runner’s time might be recorded as 10.25 seconds, while another runner’s time is 10.3 seconds. Comparing these decimals determines who ran faster. In performance metrics, decimals help athletes track their progress and identify areas for improvement. Stay on top of your game with expert analysis at COMPARE.EDU.VN.
5.4. Science and Engineering
In science and engineering, decimals are used to represent precise measurements and calculations. For example, the length of an object might be measured as 3.456 meters, or the concentration of a solution might be 0.25 moles per liter. Comparing these decimals is essential for accurate data analysis and experimentation. Achieve precision in your projects with resources from COMPARE.EDU.VN.
6. Advanced Tips for Comparing Decimals
To enhance your understanding and skills in comparing decimals, here are some advanced tips.
6.1. Estimating Decimal Values
Estimating decimal values can help you quickly determine which of two decimals is larger without going through a detailed comparison. For example, if you are comparing 5.89 and 5.23, you can estimate that 5.89 is closer to 6, while 5.23 is closer to 5. This quick estimation can give you a general idea of which decimal is larger. Improve your estimation skills with tutorials at COMPARE.EDU.VN.
6.2. Converting to Percentages for Comparison
Converting decimals to percentages can sometimes make comparisons easier, especially if you are comfortable working with percentages. To convert a decimal to a percentage, multiply it by 100. For example, 0.75 becomes 75%, and 0.8 becomes 80%. Comparing the percentages is then straightforward. Master this technique with practical examples at COMPARE.EDU.VN.
Example: Compare 0.65 and 0.7
Convert 0.65 to 65% and 0.7 to 70%. Since 70% is greater than 65%, 0.7 is greater than 0.65.
6.3. Using Mental Math Techniques
Mental math techniques can help you compare decimals quickly and efficiently. For example, you can break down the decimals into their place value components and compare each component separately. This can be particularly useful for mental calculations in everyday situations. Develop your mental math skills with exercises at COMPARE.EDU.VN.
Example: Compare 3.45 and 3.42
Break down the decimals: 3.45 = 3 + 0.4 + 0.05 and 3.42 = 3 + 0.4 + 0.02. Comparing the components, you can see that 0.05 is greater than 0.02, so 3.45 is greater than 3.42.
7. Practice Problems
To reinforce your understanding of comparing decimals, here are some practice problems:
7.1. Basic Comparison Problems
- Compare 0.25 and 0.3
- Compare 1.4 and 1.35
- Compare 5.67 and 5.6
- Compare 12.89 and 12.9
- Compare 0.05 and 0.045
7.2. Intermediate Comparison Problems
- Compare 2.345 and 2.35
- Compare 7.8 and 7.789
- Compare 10.123 and 10.12
- Compare 0.9 and 0.899
- Compare 4.56 and 4.5678
7.3. Advanced Comparison Problems
- Compare 3.14159 and 3.1416
- Compare 8.2 and 8.1999
- Compare 11.001 and 11.0009
- Compare 0.0005 and 0.00049
- Compare 6.789 and 6.78899
8. Resources for Further Learning
To continue improving your understanding of comparing decimals, here are some resources you can explore:
8.1. Online Tutorials and Videos
Numerous online tutorials and videos can provide visual and interactive explanations of comparing decimals. Websites like Khan Academy, YouTube, and Coursera offer comprehensive lessons and practice problems. These resources can be particularly helpful for visual learners. Access curated learning materials at COMPARE.EDU.VN.
8.2. Interactive Practice Websites
Interactive practice websites offer a fun and engaging way to practice comparing decimals. Websites like Math Playground, IXL, and Cool Math Games provide a variety of exercises and games that can help you improve your skills. These platforms often offer immediate feedback and track your progress. Discover engaging learning tools at COMPARE.EDU.VN.
8.3. Math Textbooks and Workbooks
Math textbooks and workbooks provide structured lessons and practice problems for comparing decimals. These resources can be particularly helpful for students who prefer a traditional learning approach. Look for textbooks and workbooks that cover decimals and place value in detail. Find recommended reading lists at COMPARE.EDU.VN.
9. Frequently Asked Questions (FAQs)
Here are some frequently asked questions about comparing decimals:
9.1. How do I compare decimals with different signs (positive and negative)?
When comparing decimals with different signs, the positive decimal is always greater than the negative decimal. For example, 2.5 is greater than -3.7. If both decimals are negative, the one closer to zero is greater. For example, -1.2 is greater than -4.5. Learn more about comparing signed numbers at COMPARE.EDU.VN.
9.2. What if the whole number parts of the decimals are very large?
If the whole number parts of the decimals are very large, you can still follow the same steps for comparing decimals. First, compare the whole number parts. If they are the same, move to the tenths place and continue comparing each place value until you find a difference. Large numbers do not change the fundamental process. Get tips for handling large numbers at COMPARE.EDU.VN.
9.3. Can I use a calculator to compare decimals?
Yes, you can use a calculator to compare decimals by subtracting one from the other. If the result is positive, the first decimal is larger. If the result is negative, the second decimal is larger. However, understanding the underlying principles of comparing decimals is still important for developing your math skills. Find recommended calculator models at COMPARE.EDU.VN.
9.4. How does comparing decimals relate to comparing fractions?
Comparing decimals and comparing fractions are closely related because decimals are just another way to represent fractions. To compare a decimal and a fraction, you can either convert the fraction to a decimal or convert the decimal to a fraction. Then, use the appropriate method to compare the two numbers. Explore the relationship between fractions and decimals at COMPARE.EDU.VN.
9.5. What is the importance of understanding place value when comparing decimals?
Understanding place value is essential for comparing decimals because it helps you determine the value of each digit in the decimal number. Each digit represents a different power of ten, and knowing these values allows you to compare the decimals accurately. Without a solid understanding of place value, it is easy to make mistakes when comparing decimals. Reinforce your understanding of place value at COMPARE.EDU.VN.
9.6. How do I compare repeating decimals?
Comparing repeating decimals can be tricky, but the key is to write out enough digits of the repeating decimal to see a pattern or difference. For example, if you are comparing 0.333… and 0.3333…, you can see that the second decimal has more 3s after the decimal point. In this case, 0.3333… is slightly greater than 0.333…. For more insights, visit COMPARE.EDU.VN.
9.7. What strategies can I use to teach children how to compare decimals?
Teaching children how to compare decimals involves using visual aids, such as place value charts and number lines, to help them understand the value of each digit. Start by comparing simple decimals and gradually increase the complexity. Use real-world examples to make the concept more relatable. Consider teaching them strategies at COMPARE.EDU.VN.
9.8. How do I compare decimals with exponents?
When comparing decimals with exponents, you first need to evaluate the exponents. For example, if you are comparing (0.2)^2 and (0.3)^2, you need to calculate 0.2 0.2 = 0.04 and 0.3 0.3 = 0.09. Then, compare the resulting decimals. Brush up on the basics of exponents at COMPARE.EDU.VN.
9.9. How to compare decimals using approximation?
Comparing decimals using approximation involves rounding the decimals to the nearest whole number or tenth to get an estimate of their values. This can help you quickly determine which decimal is larger. For example, if you are comparing 4.89 and 4.23, you can approximate 4.89 to 5 and 4.23 to 4. Since 5 is greater than 4, 4.89 is approximately greater than 4.23. Learn more about approximation techniques at COMPARE.EDU.VN.
10. Conclusion: Mastering Decimal Comparison
Mastering the art of comparing decimals is a fundamental skill with wide-ranging applications. By understanding place value, utilizing various comparison methods, and practicing regularly, you can confidently compare decimal numbers in any context. Whether you’re shopping, cooking, or working on complex scientific calculations, a solid understanding of decimal comparison is essential. For more comprehensive resources and practical exercises, visit COMPARE.EDU.VN.
Do you find yourself struggling to compare different options and make informed decisions? Visit COMPARE.EDU.VN today and discover a wealth of detailed comparisons tailored to your needs. Our comprehensive guides and expert analysis will help you make confident choices, every time. Contact us at 333 Comparison Plaza, Choice City, CA 90210, United States, or reach out via Whatsapp at +1 (626) 555-9090. Let compare.edu.vn be your trusted partner in decision-making!
