Comparing fractions with different denominators can seem tricky, but it’s a fundamental skill in mathematics. At COMPARE.EDU.VN, we provide clear and effective methods to help you master this concept, making fraction comparison straightforward. Learn how to find common denominators and utilize other techniques to accurately compare fractions and improve your numerical literacy and quantitative reasoning skills.
1. What Does It Mean to Compare Fractions?
Comparing fractions involves determining which fraction has a greater, lesser, or equal value relative to another. This comparison is crucial in various real-life scenarios, from cooking and baking to financial calculations and understanding proportions. There are several approaches to comparing fractions, including finding common denominators, using decimals, visualizing the fractions, or applying cross-multiplication.
2. Comparing Fractions: The Same Denominator Rule
When comparing fractions that share the same denominator, the process is straightforward. The fraction with the larger numerator is the greater fraction. If the numerators are equal, the fractions are equivalent.
- Example: Comparing 3/7 and 5/7. Since both fractions have the same denominator (7), we compare their numerators. Since 5 is greater than 3, 5/7 is greater than 3/7.
 Comparing Fractions With The Same Denominator
Comparing Fractions With The Same Denominator
3. How to Compare Fractions When Denominators Are Different: Finding the Common Denominator
When fractions have different denominators, you must first convert them to equivalent fractions with a common denominator. This involves finding the least common multiple (LCM) of the denominators.
3.1. Finding the Least Common Multiple (LCM)
The LCM is the smallest multiple that two or more numbers share. Finding the LCM of the denominators allows you to express the fractions with a common base, making comparison easier.
- Example: To compare 1/3 and 1/4, we first find the LCM of 3 and 4, which is 12.
3.2. Converting Fractions to Equivalent Forms
Once you’ve found the LCM, convert each fraction into an equivalent fraction with the LCM as the denominator.
- Example: Converting 1/3 and 1/4 to equivalent fractions with a denominator of 12:
- 1/3 = (1 x 4) / (3 x 4) = 4/12
- 1/4 = (1 x 3) / (4 x 3) = 3/12
3.3. Comparing Fractions With Common Denominators
After converting the fractions to a common denominator, compare the numerators to determine which fraction is larger.
- Example: Comparing 4/12 and 3/12. Since 4 is greater than 3, 4/12 (or 1/3) is greater than 3/12 (or 1/4).
4. Alternative Methods for Comparing Fractions with Different Denominators
4.1. Cross-Multiplication Method
Cross-multiplication is a quick way to compare two fractions without finding a common denominator.
- Procedure:
- Multiply the numerator of the first fraction by the denominator of the second fraction.
- Multiply the numerator of the second fraction by the denominator of the first fraction.
- Compare the two products. The fraction corresponding to the larger product is the larger fraction.
- Example: Comparing 2/5 and 3/7:
- 2 x 7 = 14
- 3 x 5 = 15
- Since 15 is greater than 14, 3/7 is greater than 2/5.
4.2. Decimal Conversion Method
Another approach is to convert each fraction into a decimal and then compare the decimal values. This method is particularly useful when dealing with fractions that are not easily converted to a common denominator.
- Procedure:
- Divide the numerator of each fraction by its denominator to obtain a decimal.
- Compare the decimal values to determine which fraction is larger.
- Example: Comparing 3/8 and 5/12:
- 3/8 = 0.375
- 5/12 ≈ 0.417
- Since 0.417 is greater than 0.375, 5/12 is greater than 3/8.
4.3. Benchmark Fractions Method
Using benchmark fractions like 0, 1/2, and 1 can help quickly estimate and compare fractions.
- Procedure:
- Assess where each fraction falls in relation to the benchmark fractions.
- Compare their relative positions to determine which fraction is larger.
- Example: Comparing 4/9 and 5/8:
- 4/9 is slightly less than 1/2.
- 5/8 is greater than 1/2.
- Therefore, 5/8 is greater than 4/9.
5. Common Mistakes When Comparing Fractions
- Ignoring the Denominator: Directly comparing numerators without considering the denominators.
- Incorrect LCM: Miscalculating the least common multiple.
- Decimal Rounding: Rounding decimal conversions too early, leading to inaccurate comparisons.
- Misapplying Rules: Forgetting which rule applies when numerators or denominators are the same.
6. Practical Examples of Comparing Fractions in Everyday Life
Comparing fractions is not just an academic exercise; it’s a practical skill that applies to numerous real-life situations.
6.1. Cooking and Baking
In cooking and baking, recipes often require comparing fractional amounts of ingredients. For example, a recipe might call for 2/3 cup of flour and 1/2 cup of sugar. Comparing these fractions helps ensure the correct proportions are used.
- Example: A recipe calls for 2/3 cup of flour and 1/2 cup of sugar. To compare, find a common denominator (6):
- 2/3 = 4/6
- 1/2 = 3/6
- Therefore, you need more flour than sugar.
6.2. Financial Calculations
Understanding fractions is essential when dealing with percentages, discounts, and interest rates.
- Example: Comparing discounts: A store offers 1/4 off on one item and 2/5 off on another. To determine which discount is better, convert to decimals or find a common denominator:
- 1/4 = 0.25 or 5/20
- 2/5 = 0.40 or 8/20
- The 2/5 discount is better.
6.3. Time Management
Fractions help in managing time effectively, such as allocating portions of your day to different activities.
- Example: If you spend 1/3 of your day working and 1/4 sleeping, you can compare these fractions to understand how much of your day is spent on each activity.
- 1/3 = 4/12
- 1/4 = 3/12
- You spend more time working than sleeping.
6.4. Measuring and Construction
In fields like construction and engineering, accuracy in measurements is crucial, and fractions are often used to represent these measurements.
- Example: Comparing the length of two pieces of wood: one is 3/8 inch thick, and the other is 1/3 inch thick. To decide which is thicker:
- 3/8 = 9/24
- 1/3 = 8/24
- The 3/8 inch piece is thicker.
6.5. Sports and Games
Fractions are used to calculate winning percentages, statistics, and other performance metrics.
- Example: A basketball player makes 7/10 of their free throws, while another makes 3/4. To compare their success rates:
- 7/10 = 14/20
- 3/4 = 15/20
- The second player has a slightly better free-throw percentage.
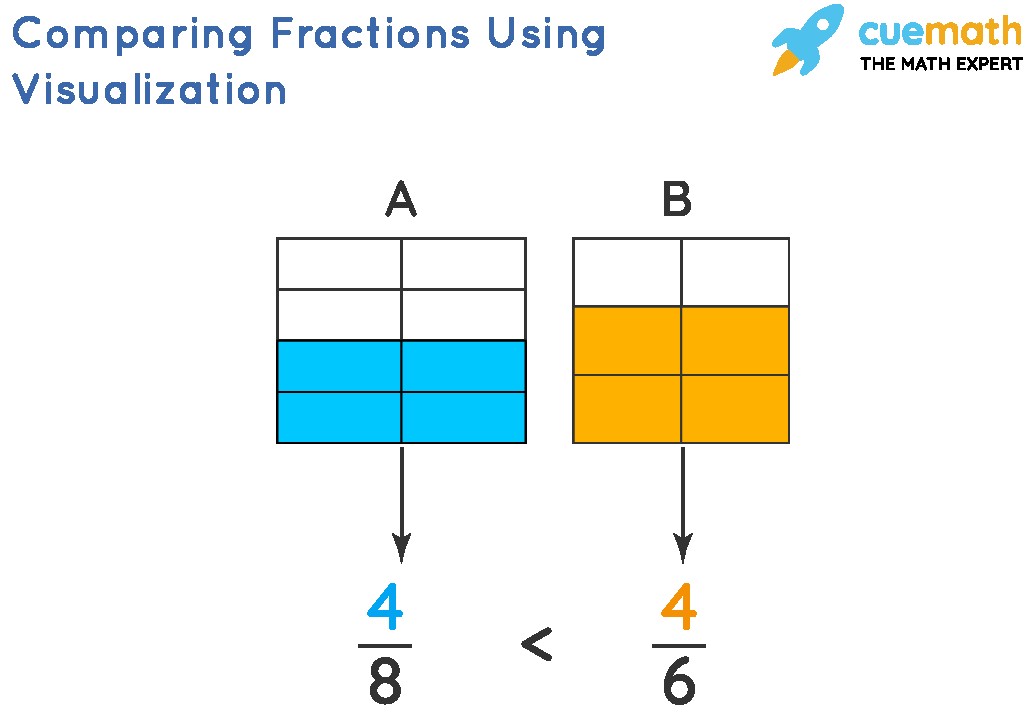
7. Visual Aids and Tools for Comparing Fractions
7.1 Fraction Bars and Circles
Fraction bars and circles are visual aids that help illustrate the size and proportion of different fractions. These tools are particularly useful for beginners as they provide a concrete representation of abstract numerical concepts. By comparing the shaded areas of fraction bars or the segments of fraction circles, students can easily see which fraction is larger or smaller.
7.2 Online Fraction Calculators
Numerous online fraction calculators are available to assist with comparing fractions. These tools can quickly convert fractions to decimals, find common denominators, and perform other operations that simplify the comparison process. They are valuable for checking answers and understanding the steps involved.
7.3 Educational Apps
Educational apps designed to teach fraction concepts often include interactive games and activities that focus on comparing fractions. These apps provide a fun and engaging way for students to practice and reinforce their understanding of fraction comparisons.
8. Tips and Tricks for Quick Fraction Comparisons
8.1 Comparing to a Half
A useful strategy for quickly comparing fractions is to use 1/2 as a benchmark. If one fraction is greater than 1/2 and another is less than 1/2, the comparison is straightforward. For example, when comparing 3/5 and 2/7, note that 3/5 is greater than 1/2, and 2/7 is less than 1/2, making 3/5 the larger fraction.
8.2 Looking at the Numerator and Denominator Difference
Another trick involves examining the difference between the numerator and the denominator. If the differences are the same for two fractions, the fraction with the larger numerator is greater. For instance, when comparing 3/4 and 5/6, both fractions have a difference of 1 between the numerator and denominator. Since 5 is greater than 3, 5/6 is the larger fraction.
8.3 Using the Butterfly Method
The butterfly method is a visual technique that involves cross-multiplying the fractions and comparing the products. Draw lines connecting the numerator of each fraction to the denominator of the other, forming a butterfly shape. Multiply along these lines and write the products above the corresponding numerators. Compare the products to determine which fraction is larger.
9. How Comparing Fractions Relates to More Advanced Math
9.1 Algebra
Understanding how to compare fractions is crucial in algebra when solving equations involving rational expressions. Simplifying complex fractions often requires comparing and combining fractional terms, making this foundational skill indispensable.
9.2 Calculus
In calculus, comparing fractions is essential when dealing with limits, series, and integrals. Evaluating the convergence or divergence of series, for example, may involve comparing fractional terms to determine the behavior of the series as it approaches infinity.
9.3 Statistics
Fractions are fundamental to statistics, where they are used to represent probabilities, proportions, and confidence intervals. Comparing fractions allows statisticians to analyze data, make inferences, and draw meaningful conclusions.
10. FAQs About Comparing Fractions
10.1. What does “comparing fractions” mean?
Comparing fractions means determining which of two or more fractions is larger, smaller, or if they are equal in value.
10.2. What is the basic rule for comparing fractions with the same denominator?
When fractions have the same denominator, the fraction with the larger numerator is the larger fraction.
10.3. How do you compare fractions with different denominators?
To compare fractions with different denominators, find the least common multiple (LCM) of the denominators, convert each fraction to an equivalent fraction with the LCM as the denominator, and then compare the numerators.
10.4. Can you use cross-multiplication to compare fractions?
Yes, cross-multiplication is a quick method for comparing two fractions. Multiply the numerator of the first fraction by the denominator of the second, and the numerator of the second fraction by the denominator of the first. Compare the two products to determine which fraction is larger.
10.5. What is the decimal method for comparing fractions?
The decimal method involves converting each fraction to a decimal by dividing the numerator by the denominator. Then, compare the decimal values to determine which fraction is larger.
10.6. Are there any common mistakes to avoid when comparing fractions?
Yes, common mistakes include ignoring the denominators, miscalculating the LCM, rounding decimal conversions too early, and misapplying the rules when numerators or denominators are the same.
10.7. How does comparing fractions relate to real-life situations?
Comparing fractions is useful in cooking, financial calculations, time management, measuring, construction, and sports, among other applications.
10.8. What are benchmark fractions, and how can they help with comparison?
Benchmark fractions like 0, 1/2, and 1 can help quickly estimate and compare fractions by assessing where each fraction falls in relation to these benchmarks.
10.9. Why is it important to understand how to compare fractions?
Understanding how to compare fractions is important for developing number sense, understanding proportions, and applying mathematical concepts in various real-life situations and more advanced mathematical studies.
10.10. What tools can help in comparing fractions?
Tools that can help in comparing fractions include fraction bars, fraction circles, online fraction calculators, and educational apps.
11. Conclusion: Mastering Fraction Comparisons with COMPARE.EDU.VN
Mastering how to compare fractions, especially when the denominators are different, is essential for a strong foundation in mathematics. Whether you use the common denominator method, cross-multiplication, decimal conversion, or benchmark fractions, the ability to accurately compare fractions is invaluable. At COMPARE.EDU.VN, we are dedicated to providing you with clear, comprehensive guides and resources to enhance your mathematical skills and build confidence in tackling numerical challenges.
Do you want to make confident decisions based on comprehensive comparisons? Visit COMPARE.EDU.VN today to explore a wide range of comparisons and discover the best choices for your needs. Whether it’s educational resources, financial products, or everyday services, we help you compare and choose with confidence.
Contact Information:
- Address: 333 Comparison Plaza, Choice City, CA 90210, United States
- WhatsApp: +1 (626) 555-9090
- Website: compare.edu.vn