How Can You Compare Two Fractions Easily?

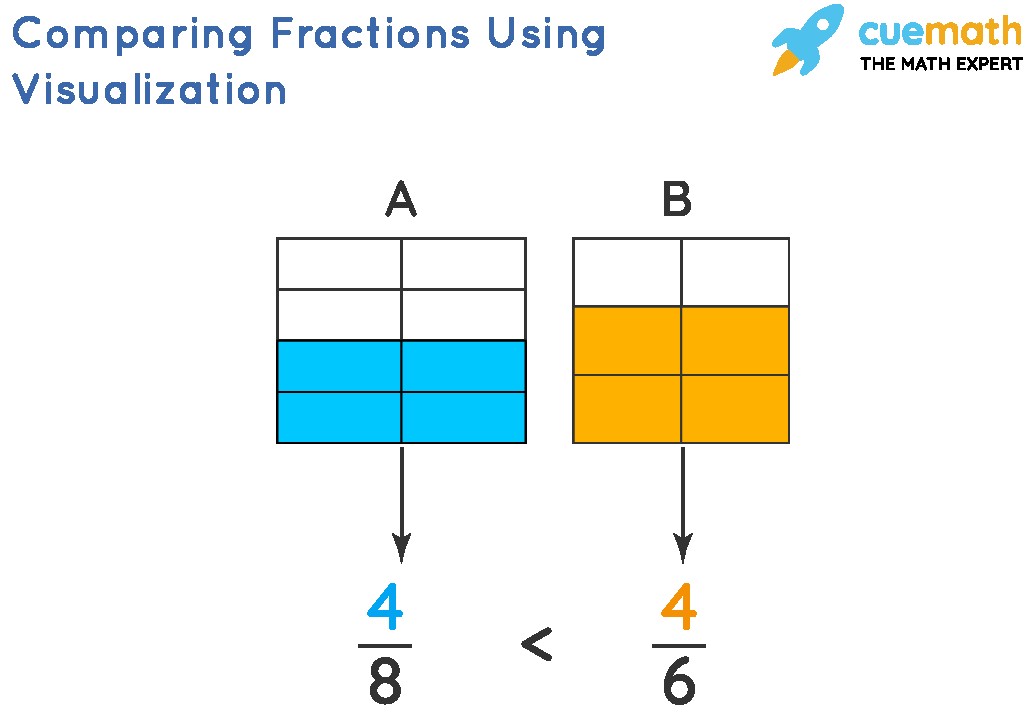
Comparing fractions can seem tricky, but it’s an essential skill in mathematics and everyday life. At COMPARE.EDU.VN, we provide clear and comprehensive guides to make fraction comparison straightforward. Discover effective strategies for fraction comparison, including common denominators and cross-multiplication.

1. Understanding the Basics: What is a Fraction?

Before diving into How Can You Compare Two Fractions, it’s crucial to understand what a fraction represents. A fraction is a part of a whole, consisting of two primary components: the numerator and the denominator. The numerator (top number) indicates how many parts of the whole you have, while the denominator (bottom number) indicates the total number of equal parts that make up the whole.

For instance, in the fraction 3/4, ‘3’ is the numerator, showing that we have three parts, and ‘4’ is the denominator, indicating that the whole is divided into four equal parts. Visualizing fractions can greatly aid in understanding their values and how they relate to each other.

Understanding this fundamental concept is the first step towards mastering the comparison of fractions. From here, we can explore various methods to accurately determine the relative size of different fractions.

2. Why is Comparing Fractions Important?

Knowing how can you compare two fractions is more than just a mathematical exercise; it has practical applications in various real-life scenarios. Whether you’re adjusting a recipe, calculating proportions, or managing finances, understanding how fractions relate to each other is essential. For example:

Cooking: Imagine you need to halve a recipe that calls for 2/3 cup of flour. Understanding that half of 2/3 is 1/3 allows you to accurately adjust the ingredients.
Construction: Accurately measuring and cutting materials often involves fractions. Comparing fractions ensures precise dimensions.
Finance: Comparing fractions can help you understand discounts, interest rates, and investment returns. Knowing whether 1/4 off an item is a better deal than 20% off requires comparing fractions.

Furthermore, comparing fractions is a foundational skill for more advanced mathematical concepts such as algebra and calculus. A solid understanding of fraction comparison builds confidence and proficiency in handling mathematical problems across various disciplines. Therefore, mastering the techniques for comparing fractions is a valuable investment in your mathematical journey and everyday life.

3. Method 1: Comparing Fractions with the Same Denominator

When learning how can you compare two fractions, the simplest scenario is when the fractions have the same denominator. In this case, comparing the fractions becomes straightforward: you only need to compare the numerators.

The rule is: if two fractions have the same denominator, the fraction with the larger numerator is the larger fraction.

For example, let’s compare 3/7 and 5/7. Both fractions have the same denominator (7), so we compare their numerators:

3 is less than 5 (3 < 5)
Therefore, 3/7 is less than 5/7 (3/7 < 5/7)

Similarly, if we compare 8/11 and 2/11:

8 is greater than 2 (8 > 2)
Therefore, 8/11 is greater than 2/11 (8/11 > 2/11)

This method simplifies the comparison process by eliminating the need to adjust the fractions. It’s an excellent starting point for understanding how can you compare two fractions. The key takeaway is to always check the denominators first. If they are the same, focus on the numerators to determine which fraction is larger.

4. Method 2: Finding a Common Denominator

When the fractions you want to compare have different denominators, you need to find a common denominator. This allows you to rewrite the fractions so that they have the same denominator, making comparison straightforward. This method is crucial in understanding how can you compare two fractions when they don’t immediately lend themselves to easy comparison. Here’s how to do it:

Find the Least Common Multiple (LCM): The LCM of the denominators is the smallest number that both denominators can divide into evenly. This becomes your common denominator.
Convert the Fractions: Multiply both the numerator and denominator of each fraction by the number that makes its denominator equal to the LCM.

Let’s compare 1/3 and 2/5.

Find the LCM of 3 and 5: The LCM of 3 and 5 is 15.
Convert 1/3 to have a denominator of 15: Multiply both numerator and denominator by 5: (1 5) / (3 5) = 5/15
Convert 2/5 to have a denominator of 15: Multiply both numerator and denominator by 3: (2 3) / (5 3) = 6/15
Compare the New Fractions: Now we compare 5/15 and 6/15. Since 6 is greater than 5, 6/15 is greater than 5/15.

Therefore, 2/5 is greater than 1/3.

Finding a common denominator is a fundamental technique in understanding how can you compare two fractions that initially seem incomparable. Once the fractions have the same denominator, you can simply compare the numerators as described in the previous method. This ensures an accurate comparison, regardless of the original denominators.

5. Method 3: Cross-Multiplication for Comparing Fractions

Cross-multiplication offers a quick and effective way to compare two fractions without explicitly finding a common denominator. This technique is particularly useful when you need a fast answer and is another excellent method for understanding how can you compare two fractions. Here’s how it works:

Multiply Diagonally: Multiply the numerator of the first fraction by the denominator of the second fraction. Then, multiply the numerator of the second fraction by the denominator of the first fraction.
Compare the Products: The fraction that corresponds to the larger product is the larger fraction.

Let’s compare 3/4 and 5/7 using cross-multiplication:

Cross-multiply:
- 3 (numerator of the first fraction) × 7 (denominator of the second fraction) = 21
- 5 (numerator of the second fraction) × 4 (denominator of the first fraction) = 20
Compare the products:
- 21 is greater than 20 (21 > 20)
Determine the larger fraction:
- Since 21 corresponds to the first fraction (3/4), 3/4 is greater than 5/7.

Therefore, 3/4 > 5/7.

Cross-multiplication is a valuable tool because it streamlines the comparison process, eliminating the step of finding a common denominator. It’s an efficient method for quickly determining the relative size of two fractions. Understanding this method enhances your ability to confidently determine how can you compare two fractions in various scenarios.

6. Method 4: Converting Fractions to Decimals

Another effective method for how can you compare two fractions is to convert them into decimal form. This technique is particularly useful when dealing with complex fractions or when you prefer working with decimals. Here’s how to convert fractions to decimals and compare them:

Divide: Divide the numerator of each fraction by its denominator. The result is the decimal equivalent of the fraction.
Compare the Decimals: Compare the decimal values. The fraction with the larger decimal value is the larger fraction.

Let’s compare 3/8 and 4/9 by converting them to decimals:

Convert 3/8 to a decimal:
- 3 ÷ 8 = 0.375
Convert 4/9 to a decimal:
- 4 ÷ 9 = 0.444 (repeating)
Compare the decimal values:
- 1. 375 is less than 0.444 (0.375 < 0.444)
Determine the larger fraction:
- Since 0. 444 corresponds to the second fraction (4/9), 4/9 is greater than 3/8.

Therefore, 4/9 > 3/8.

Converting fractions to decimals is a straightforward method that allows for easy comparison, especially when the fractions have denominators that are not easily converted to a common denominator. By transforming fractions into decimals, you can quickly assess their relative sizes and confidently answer how can you compare two fractions using decimal values.

7. Method 5: Using Benchmarks for Fraction Comparison

Benchmarks are common fractions that serve as reference points to quickly estimate and compare other fractions. This method is especially helpful for developing number sense and providing a mental shortcut for how can you compare two fractions. Common benchmarks include 0, 1/2, and 1. Here’s how to use benchmarks to compare fractions:

Determine Proximity to Benchmarks: Assess whether each fraction is closer to 0, 1/2, or 1.
Compare Relative Positions: Compare the positions of the fractions relative to these benchmarks.

Let’s compare 3/7 and 5/8 using benchmarks:

Assess 3/7:
- Half of 7 is 3.5, so 3/7 is slightly less than 1/2.
Assess 5/8:
- Half of 8 is 4, so 5/8 is greater than 1/2.
Compare Relative Positions:
- Since 3/7 is less than 1/2 and 5/8 is greater than 1/2, we can conclude that 5/8 is larger than 3/7.

Therefore, 5/8 > 3/7.

Using benchmarks simplifies the comparison process by providing familiar reference points. This method allows you to quickly estimate and compare fractions without performing detailed calculations, making it a valuable skill for understanding how can you compare two fractions in everyday situations. By relating fractions to these common benchmarks, you can develop a better intuition for their values and relative sizes.

8. Special Case: Comparing Fractions with the Same Numerator

An interesting special case in understanding how can you compare two fractions arises when the fractions have the same numerator. In this scenario, the rule is: the fraction with the smaller denominator is the larger fraction. This may seem counterintuitive at first, but it becomes clear when you consider what the denominator represents.

The denominator indicates how many parts the whole is divided into. If you have the same number of parts (numerator) but the whole is divided into fewer pieces (smaller denominator), each piece must be larger.

Let’s compare 2/5 and 2/7. Both fractions have the same numerator (2), so we compare their denominators:

5 is less than 7 (5 < 7)
Therefore, 2/5 is greater than 2/7 (2/5 > 2/7)

This can be visualized by imagining two pizzas. One is divided into 5 slices, and you take 2 slices. The other is divided into 7 slices, and you also take 2 slices. The slices from the pizza divided into 5 pieces are clearly larger.

This rule provides a quick way to compare fractions with the same numerator, offering another valuable technique for answering how can you compare two fractions in specific situations.

9. Common Mistakes to Avoid When Comparing Fractions

When learning how can you compare two fractions, it’s important to be aware of common mistakes that can lead to incorrect conclusions. Here are a few pitfalls to avoid:

Incorrectly Applying Whole Number Rules: One common mistake is assuming that the rules for comparing whole numbers apply directly to fractions. For example, assuming that because 5 is greater than 3, then 1/5 must be greater than 1/3. Remember, with fractions, the larger the denominator, the smaller the fraction when the numerators are the same.
Ignoring Denominators: When fractions have different denominators, you can’t simply compare the numerators. You must first find a common denominator or use another method like cross-multiplication or converting to decimals.
Arithmetic Errors: Make sure to double-check your calculations when finding common denominators or converting fractions to decimals. Even a small arithmetic error can lead to an incorrect comparison.
Not Simplifying Fractions First: Sometimes, simplifying fractions before comparing them can make the process easier. Always check if the fractions can be reduced to simpler forms before applying any comparison method.
Rushing the Process: Take your time and carefully follow the steps for each comparison method. Rushing can lead to careless mistakes and incorrect answers.

By being mindful of these common mistakes, you can improve your accuracy and confidence when comparing fractions. Understanding what not to do is just as important as knowing the correct methods for how can you compare two fractions.

10. Practice Exercises: Test Your Skills

To solidify your understanding of how can you compare two fractions, practice is essential. Here are some exercises to help you test and improve your skills:

Compare 2/3 and 3/4.
Which is larger: 5/8 or 7/12?
Compare 1/5 and 2/9.
Is 4/7 greater than or less than 5/9?
Compare 3/10 and 2/7.
Which is smaller: 6/11 or 7/13?
Compare 1/4, 2/5, and 3/8.
Is 5/6 greater than, less than, or equal to 10/12?
Compare 4/15 and 3/11.
Which is larger: 8/9 or 9/10?

Answer Key:

3/4 > 2/3 (3/4 is larger)
7/12 > 5/8 (7/12 is larger)
2/9 > 1/5 (2/9 is larger)
4/7 < 5/9 (4/7 is less than 5/9)
2/7 > 3/10 (2/7 is larger)
6/11 < 7/13 (6/11 is smaller)
2/5 > 3/8 > 1/4 (2/5 is the largest, 1/4 is the smallest)
5/6 = 10/12 (equal)
3/11 > 4/15 (3/11 is larger)
9/10 > 8/9 (9/10 is larger)

Work through these exercises using the methods discussed earlier, such as finding a common denominator, cross-multiplication, or converting to decimals. Check your answers against the key to see how well you’ve grasped the concepts. Consistent practice will build your confidence and proficiency in how can you compare two fractions.

11. Advanced Techniques for Complex Fractions

As you become more comfortable with the basics, you can explore advanced techniques for comparing more complex fractions. These methods are particularly useful when dealing with mixed numbers, improper fractions, or fractions with large denominators. Here are some advanced techniques to further enhance your understanding of how can you compare two fractions:

Converting Mixed Numbers to Improper Fractions: When comparing mixed numbers (e.g., 2 1/3), first convert them to improper fractions (e.g., 7/3). This makes comparison easier, especially when the whole number parts are different.
Simplifying Fractions: Before comparing, simplify fractions to their lowest terms. This reduces the size of the numbers you’re working with and can make the comparison process more manageable.
Using Proportional Reasoning: For fractions with large denominators, use proportional reasoning to estimate and compare. For example, if one fraction is slightly less than 1/2 and the other is slightly more than 1/2, you can quickly determine which is larger without precise calculations.
Comparing Differences from a Common Value: Instead of comparing the fractions directly, compare their differences from a common value, such as 1. For example, if one fraction is closer to 1 than the other, it is the larger fraction.
Combining Methods: Use a combination of methods to compare fractions. For example, you might simplify fractions first, then find a common denominator, and finally compare the numerators.

By mastering these advanced techniques, you’ll be well-equipped to tackle even the most challenging fraction comparison problems. These skills will deepen your understanding of how can you compare two fractions and enhance your overall mathematical proficiency.

12. Real-World Applications of Fraction Comparison

Understanding how can you compare two fractions is not just an academic exercise; it’s a practical skill with numerous real-world applications. Here are some examples of how fraction comparison is used in everyday life:

Cooking and Baking: Recipes often involve fractional measurements. Comparing fractions allows you to adjust recipes accurately, whether you’re doubling, halving, or making other modifications.
Construction and Home Improvement: Measuring materials and calculating dimensions frequently involve fractions. Comparing fractions ensures precise cuts and accurate project execution.
Finance and Budgeting: Understanding fractions is essential for managing finances. Comparing fractions can help you determine discounts, interest rates, and investment returns. For example, knowing whether 1/4 off an item is a better deal than 20% off requires comparing fractions.
Time Management: Dividing tasks and scheduling activities often involve fractions of time. Comparing fractions helps you allocate time effectively and prioritize tasks.
Travel and Distance: Reading maps and planning routes can involve fractions of distances. Comparing fractions allows you to estimate travel times and choose the most efficient routes.
Sports and Statistics: Analyzing sports statistics often involves fractions, such as batting averages or shooting percentages. Comparing fractions helps you evaluate player performance and team strategies.
Healthcare and Medication: Administering medications and following medical instructions can involve fractional dosages. Comparing fractions ensures accurate measurements and safe practices.

From the kitchen to the construction site, from the bank to the sports field, fraction comparison is a valuable skill that empowers you to make informed decisions and solve practical problems. Mastering how can you compare two fractions enhances your ability to navigate everyday situations with confidence and accuracy.

13. How COMPARE.EDU.VN Can Help You Master Fraction Comparisons

At COMPARE.EDU.VN, we understand the importance of mastering fraction comparisons. We provide a wealth of resources designed to help you develop a deep and practical understanding of how can you compare two fractions. Here are some ways COMPARE.EDU.VN can assist you:

Comprehensive Guides: Our detailed guides cover all aspects of fraction comparison, from basic concepts to advanced techniques. We break down complex topics into easy-to-understand explanations and step-by-step instructions.
Interactive Tutorials: Engage with interactive tutorials that allow you to practice fraction comparisons in a dynamic and engaging way. These tutorials provide immediate feedback to help you identify and correct mistakes.
Practice Exercises: Test your skills with a wide range of practice exercises, ranging from simple comparisons to more challenging problems. Our exercises are designed to reinforce your understanding and build your confidence.
Real-World Examples: Explore real-world examples that illustrate the practical applications of fraction comparison. These examples demonstrate how fraction comparison is used in everyday life, from cooking to finance to construction.
Expert Tips and Strategies: Benefit from expert tips and strategies that simplify the comparison process. Learn shortcuts and techniques that make fraction comparisons faster and more efficient.
Community Support: Connect with a community of learners who are also working to master fraction comparisons. Share tips, ask questions, and collaborate with others to deepen your understanding.

With COMPARE.EDU.VN, you have access to a comprehensive suite of resources that will empower you to confidently and accurately compare fractions in any situation. Visit our website today to start your journey towards mastering how can you compare two fractions.

We are located at 333 Comparison Plaza, Choice City, CA 90210, United States. Contact us via Whatsapp: +1 (626) 555-9090 or visit our website: COMPARE.EDU.VN.

14. Frequently Asked Questions (FAQs) About Comparing Fractions

To further clarify your understanding of how can you compare two fractions, here are some frequently asked questions with detailed answers:

Q1: What is the basic principle behind comparing fractions?

A: The basic principle is to determine which fraction represents a larger portion of a whole. This can be done by ensuring both fractions refer to the same-sized “whole” (having a common denominator) or by converting them into a format that allows for direct comparison, such as decimals.

Q2: How do I compare fractions with different denominators?

A: To compare fractions with different denominators, find a common denominator (usually the least common multiple or LCM of the denominators). Convert each fraction to have this common denominator, then compare the numerators. The fraction with the larger numerator is the larger fraction.

Q3: Can I use cross-multiplication to compare any two fractions?

A: Yes, cross-multiplication is a quick method to compare any two fractions. Multiply the numerator of the first fraction by the denominator of the second fraction and vice versa. Compare the resulting products to determine which fraction is larger.

Q4: What if the fractions have the same numerator?

A: If the fractions have the same numerator, the fraction with the smaller denominator is the larger fraction. This is because the whole is divided into fewer parts, making each part larger.

Q5: Is it always necessary to find the LCM to compare fractions?

A: No, while finding the LCM is a reliable method, it’s not always necessary. You can also use cross-multiplication or convert the fractions to decimals for comparison.

Q6: How do I compare mixed numbers?

A: To compare mixed numbers, first compare the whole number parts. If they are different, the mixed number with the larger whole number is larger. If the whole number parts are the same, compare the fractional parts using any of the methods discussed (common denominator, cross-multiplication, etc.).

Q7: What is a benchmark fraction, and how is it useful?

A: A benchmark fraction is a common fraction like 0, 1/2, or 1 that serves as a reference point for estimating and comparing other fractions. By relating fractions to these benchmarks, you can quickly assess their relative sizes.

Q8: How do I compare three or more fractions at once?

A: To compare three or more fractions, find a common denominator for all fractions. Convert each fraction to have this common denominator, then compare the numerators.

Q9: What are some common mistakes to avoid when comparing fractions?

A: Common mistakes include incorrectly applying whole number rules, ignoring denominators, arithmetic errors, not simplifying fractions first, and rushing the process.

Q10: Where can I find more resources to practice comparing fractions?

A: COMPARE.EDU.VN offers comprehensive guides, interactive tutorials, practice exercises, real-world examples, expert tips, and community support to help you master comparing fractions. Visit our website for more information.

These FAQs address common questions and concerns related to how can you compare two fractions, providing clear and concise answers to help you build a solid understanding.

15. Take the Next Step: Master Fraction Comparison Today

Mastering fraction comparison is a valuable skill that can benefit you in numerous areas of life. By understanding and practicing the methods discussed in this guide, you can confidently and accurately compare fractions in any situation. Don’t let fractions intimidate you – with the right knowledge and resources, you can conquer them.

At COMPARE.EDU.VN, we are committed to providing you with the tools and support you need to succeed. Whether you’re a student, a professional, or simply someone who wants to improve their math skills, our comprehensive resources can help you master fraction comparison and other essential mathematical concepts.

Take the next step and unlock your full potential. Visit COMPARE.EDU.VN today to access our detailed guides, interactive tutorials, practice exercises, and community support. Start your journey towards mastering how can you compare two fractions and experience the confidence and empowerment that comes with mathematical proficiency.

Ready to take your fraction comparison skills to the next level? Visit COMPARE.EDU.VN now and discover the difference! Located at 333 Comparison Plaza, Choice City, CA 90210, United States. Whatsapp: +1 (626) 555-9090.

Don’t wait – start mastering fraction comparison today!

Call to Action:

Visit compare.edu.vn to discover comprehensive guides and resources that simplify fraction comparison. Make informed decisions with confidence today.