How Can a Ratio Be Used To Compare Quantities Effectively?

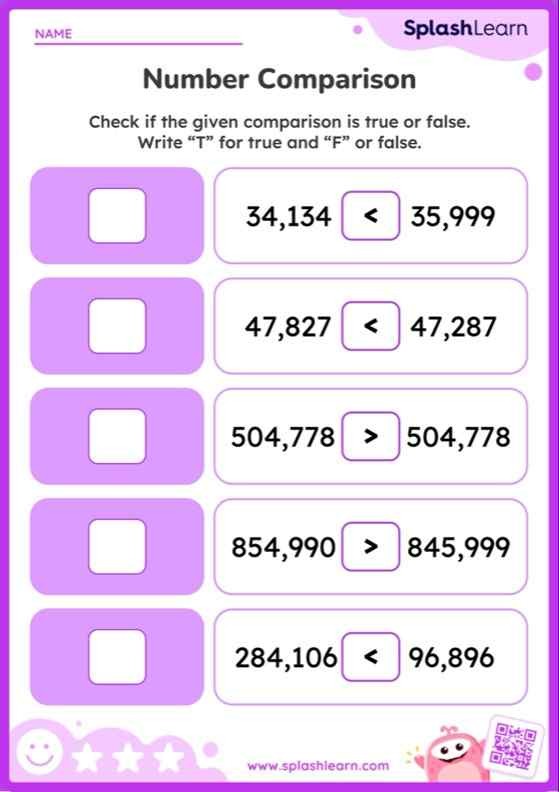
Comparing ratios is essential for understanding the relationship between different quantities. It allows us to determine if one ratio is less than, greater than, or equal to another, offering valuable insights in various fields. COMPARE.EDU.VN provides comprehensive comparisons to help you make informed decisions.

This article explores the methods and applications of ratio comparisons, providing you with the knowledge and tools to make well-informed comparisons. You will also learn about benchmark comparisons and comparative analysis.

1. Understanding the Essence of Ratios

A ratio is a comparison of two quantities of the same kind. It illustrates how much of one quantity is contained within another. Represented as a:b, a ratio helps in understanding the relative sizes or proportions of two amounts.

For instance, consider a recipe where the ratio of flour to sugar is 3:1. This signifies that for every 3 units of flour, 1 unit of sugar is required. Ratios simplify complex data, making comparisons straightforward.

In the ratio a:b, ‘a’ is known as the antecedent and ‘b’ is the consequent. Understanding these terms helps in interpreting and using ratios effectively.

2. Key Methods for Comparing Ratios

Several methods exist for comparing ratios, each with its own advantages. Here are some of the most common techniques:

2.1. The LCM Method

The Least Common Multiple (LCM) method is useful when the consequents (denominators) of the ratios are different. By finding the LCM and converting the ratios to equivalent forms with a common denominator, direct comparison becomes simple.

Step-by-Step Guide:

1. Identify the Ratios: Start with the ratios you want to compare, such as 2:3 and 4:5.

2. Write as Fractions: Convert the ratios into fraction form: $frac{2}{3}$ and $frac{4}{5}$.

3. Find the LCM: Determine the LCM of the denominators (3 and 5). In this case, LCM(3, 5) = 15.

4. Convert to Equivalent Fractions: Rewrite the fractions with the LCM as the common denominator.

   • $frac{2}{3} = frac{2 times 5}{3 times 5} = frac{10}{15}$
   • $frac{4}{5} = frac{4 times 3}{5 times 3} = frac{12}{15}$

5. Compare the Numerators: Now that the denominators are the same, compare the numerators. Since 10 < 12, $frac{10}{15} < frac{12}{15}$.

6. Conclude: Therefore, the ratio 4:5 is greater than the ratio 2:3.

The LCM method ensures accurate comparisons by standardizing the denominators, making it easier to assess the relative sizes of the ratios. This method can be applied to various scenarios, such as comparing the concentration of solutions in chemistry or analyzing financial ratios in business.

2.2. Cross Multiplication Method

Cross multiplication provides a quick way to compare two ratios by multiplying the numerator of one ratio by the denominator of the other and comparing the products.

Understanding the Principles:

• Equality: If two ratios are equal ($frac{a}{b} = frac{c}{d}$), then the product of cross multiplication is also equal ($a times d = b times c$).

  • Example: $frac{1}{2} = frac{2}{4}$ because $1 times 4 = 2 times 2$.

• Less Than: If $frac{a}{b} < frac{c}{d}$, then $a times d < b times c$.

  • Example: $frac{1}{2} < frac{3}{4}$ because $1 times 4 < 2 times 3$ (4 < 6).

• Greater Than: If $frac{a}{b} > frac{c}{d}$, then $a times d > b times c$.

  • Example: $frac{1}{10} > frac{1}{100}$ because $1 times 100 > 10 times 1$ (100 > 10).

How to Apply Cross Multiplication:

1. Set up the Ratios: Write the ratios you want to compare as fractions, e.g., $frac{a}{b}$ and $frac{c}{d}$.
2. Cross Multiply: Multiply the numerator of the first fraction by the denominator of the second fraction ($a times d$) and the numerator of the second fraction by the denominator of the first fraction ($b times c$).
3. Compare the Products: Compare the two products to determine which ratio is larger or if they are equal.

This method is particularly useful for quick comparisons and is often used in solving proportions and determining the relative sizes of different quantities. Cross multiplication is applicable in fields like finance for comparing investment returns or in physics for comparing velocities.

2.3. Using Ratio Tables

Ratio tables offer a structured way to compare multiple ratios. This method is especially useful when dealing with more than two ratios, providing a clear side-by-side comparison.

Creating and Using Ratio Tables:

1. List Ratios: Start by listing the ratios you want to compare.
2. Generate Equivalent Ratios: Create equivalent ratios for each original ratio. Equivalent ratios are obtained by multiplying both parts of the ratio by the same number.
3. Organize in a Table: Arrange the ratios and their equivalents in a table format.
4. Identify Common Denominators or Numerators: Look for equivalent ratios with common denominators or numerators. This simplifies the comparison.
5. Compare: Once you have common denominators or numerators, compare the remaining values to determine the relationship between the ratios.

Example: Comparing 2:3 and 4:5 Using a Table

To compare the ratios 2:3 and 4:5, list their equivalent ratios in a table:

Ratio 2:3
2	4	6	8	10
3	6	9	12	15

Ratio 4:5
4	8	12	16	20
5	10	15	20	25

From the table, we can see that $frac{10}{15}$ and $frac{12}{15}$ are equivalent ratios with the same denominator. Thus, since 10 < 12, we conclude that 2:3 < 4:5.

Applications of Ratio Tables:

Ratio tables are highly versatile and can be used in various contexts:

• Cooking: Adjusting recipes for different serving sizes.
• Construction: Scaling architectural plans.
• Business: Analyzing financial performance and comparing different investment options.

Determining Unit Ratios:

Ratio tables can also be used to find unit rates, which are ratios where the second term is 1. This is particularly useful in scenarios like determining who walks the fastest among a group of people, as shown in the example.

Ratio tables provide a clear and organized approach to comparing ratios, making them an essential tool for both simple and complex comparisons.

2.4. Converting to Decimal Numbers

Converting ratios to decimal numbers provides a straightforward method for comparison. By dividing the antecedent by the consequent, you obtain a decimal value that can be easily compared across different ratios.

Step-by-Step Conversion:

1. Write as Fractions: Express each ratio as a fraction. For example, the ratio 3:4 becomes $frac{3}{4}$.

2. Divide: Divide the numerator (antecedent) by the denominator (consequent) to get a decimal.

   • For 3:4, $frac{3}{4} = 0.75$
   • For 5:8, $frac{5}{8} = 0.625$

3. Compare Decimals: Compare the decimal values to determine which ratio is larger or smaller.

   • Since 0.75 > 0.625, the ratio 3:4 is greater than the ratio 5:8.

Advantages of Using Decimals:

• Ease of Comparison: Decimal numbers are easy to compare, especially with calculators or computers.
• Universality: This method works for any type of ratio, regardless of the magnitude of the numbers involved.

Example:

Compare the ratios 7:5 and 11:8.

• 7:5 = $frac{7}{5}$ = 1.4
• 11:8 = $frac{11}{8}$ = 1.375

Since 1.4 > 1.375, the ratio 7:5 is greater than the ratio 11:8.

2.5. Converting to Percentages

Converting ratios to percentages is one of the simplest and most intuitive ways to compare them. This method involves transforming each ratio into a percentage, allowing for direct comparison on a standardized scale.

How to Convert Ratios to Percentages:

1. Write as Fractions: Start by expressing the ratio as a fraction. For instance, the ratio 3:4 becomes $frac{3}{4}$.

2. Multiply by 100: Multiply the fraction by 100 to convert it to a percentage.

   • For 3:4: $frac{3}{4} times 100 = 75%$
   • For 1:2: $frac{1}{2} times 100 = 50%$

3. Compare Percentages: Compare the resulting percentages to determine which ratio is larger or smaller.

   • Since 75% > 50%, the ratio 3:4 is greater than the ratio 1:2.

Advantages of Using Percentages:

• Standardized Scale: Percentages provide a standardized scale (0-100), making comparisons straightforward.
• Intuitive Understanding: Most people are familiar with percentages, making this method easy to understand and apply.
• Wide Applicability: This method can be used in various fields, from finance to statistics.

Examples:

1. Compare 9:10 and 7:5

   • $frac{9}{10} times 100 = 90%$
   • $frac{7}{5} times 100 = 140%$
   • Conclusion: $frac{9}{10} < frac{7}{5}$

2. Comparing Investment Returns

   • Investment A: Returns a profit of $30 for every $200 invested. Ratio: 30:200 = 15%
   • Investment B: Returns a profit of $45 for every $300 invested. Ratio: 45:300 = 15%
   • Conclusion: Both investments have the same return rate.

3. Practical Applications of Comparing Ratios

Comparing ratios has a wide array of practical applications across various fields, enabling informed decision-making and problem-solving.

3.1. Business and Finance

In business, ratios are crucial for financial analysis. For example, the debt-to-equity ratio compares a company’s total debt to its shareholder equity. A lower ratio indicates a more financially stable company. Investors use ratios to assess a company’s profitability, efficiency, and solvency.

• Profit Margin: Measures how much of each dollar of revenue a company keeps as profit.
• Return on Investment (ROI): Evaluates the efficiency of an investment by comparing the gain or loss from an investment relative to its cost.
• Liquidity Ratios: Assess a company’s ability to meet its short-term obligations.

3.2. Cooking and Baking

Ratios are fundamental in cooking and baking. A recipe might specify a ratio of water to rice as 2:1, ensuring the rice is cooked perfectly. Chefs use ratios to scale recipes up or down while maintaining the correct proportions of ingredients.

• Flour to Liquid Ratio: Determines the consistency of dough in baking.
• Sugar to Fat Ratio: Affects the texture and sweetness of baked goods.
• Spice Blends: Achieving the desired flavor profile by maintaining consistent ratios of spices.

3.3. Science and Engineering

Scientists and engineers use ratios to analyze experimental data, compare measurements, and design structures. For instance, the aspect ratio of an airplane wing (the ratio of its span to its chord) affects its aerodynamic performance.

• Concentration Ratios: Used in chemistry to express the amount of solute in a solution.
• Gear Ratios: Determine the speed and torque in mechanical systems.
• Mixing Ratios: Essential in material science for creating compounds with specific properties.

3.4. Healthcare

In healthcare, ratios are used to assess patient health and administer medication. For example, the body mass index (BMI) is a ratio of weight to height, used to categorize individuals as underweight, normal weight, overweight, or obese.

• Medication Dosage: Ensuring the correct amount of drug is administered based on body weight or age.
• Nurse-to-Patient Ratio: Affects the quality of patient care in hospitals.
• Ventilation Ratios: Maintaining proper oxygen and carbon dioxide levels in respiratory care.

3.5. Sports and Fitness

Athletes and trainers use ratios to optimize performance. For instance, the strength-to-weight ratio is crucial in sports like weightlifting and gymnastics, where athletes need to maximize their strength relative to their body weight.

• Macronutrient Ratios: Balancing protein, carbohydrates, and fats in a diet for optimal performance.
• Work-to-Rest Ratio: Structuring training sessions to maximize recovery and prevent overtraining.
• Win-Loss Ratio: Assessing a team’s or player’s success rate over a season.

4. Essential Facts about Comparing Ratios

To effectively compare ratios, it’s important to keep several key facts in mind. These facts ensure accurate interpretation and application of ratios in various contexts.

4.1. Ratios as Comparisons

A ratio is a method of comparing two quantities of the same kind by division. It provides insights into the relative sizes or proportions of these quantities.

4.2. Scale Factor

Ratios explain the scale factor, indicating how much something is enlarged or reduced in size. This is particularly useful in fields like cartography and architecture.

4.3. Unit Ratios

A unit ratio is in the form a:1, where the second term equals 1. Converting ratios to unit ratios simplifies comparison, as you only need to compare the first terms.

4.4. Art and Design

The golden ratio (approximately 1.618) is used in art and design to create visually pleasing compositions. Comparing ratios helps designers achieve balanced and harmonious layouts.

4.5. Cooking and Baking

Comparing ratios is essential for adjusting recipes. Knowing the correct ratio of ingredients ensures consistent and delicious results.

4.6. Same Units

Quantities must be expressed in the same units to compare them using ratios. For instance, comparing distances requires both measurements to be in miles or kilometers.

4.7. No Unit

A ratio has no unit because the units cancel out during division. This makes ratios pure numbers, suitable for comparison across different scales.

4.8. Order Matters

The order of terms in a ratio is critical. The ratio a:b is different from the ratio b:a, as they represent different comparisons.

4.9. Pi (π)

The ratio of a circle’s circumference to its diameter is always the same, approximately 3.14159. This constant ratio, denoted as pi (π), is fundamental in geometry and trigonometry.

4.10. Ratio Value

If the first term is greater than the second, the ratio is greater than 1. Conversely, if the ratio is less than 1, the second term is greater than the first.

5. Solved Examples: Mastering Ratio Comparisons

To solidify your understanding of comparing ratios, let’s walk through some solved examples using different methods.

Example 1: LCM Method

Problem: Compare the ratios 3:5 and 2:7 using the LCM method.

Solution:

1. Convert to Fractions:
   • 3:5 = $frac{3}{5}$
   • 2:7 = $frac{2}{7}$
2. Find the LCM: The LCM of 5 and 7 is 35.
3. Rewrite with Common Denominator:
   • $frac{3}{5} = frac{3 times 7}{5 times 7} = frac{21}{35}$
   • $frac{2}{7} = frac{2 times 5}{7 times 5} = frac{10}{35}$
4. Compare Numerators: Since 21 > 10, $frac{21}{35} > frac{10}{35}$.
5. Conclusion: Therefore, the ratio 3:5 is larger than the ratio 2:7.

Example 2: Cross-Multiplication Method

Problem: Compare the ratios 4:5 and 6:7 using the cross-multiplication method.

Solution:

1. Write as Fractions:
   • 4:5 = $frac{4}{5}$
   • 6:7 = $frac{6}{7}$
2. Cross Multiply:
   • $4 times 7 = 28$
   • $6 times 5 = 30$
3. Compare Products: Since 28 < 30, the ratio 4:5 is less than the ratio 6:7.
4. Conclusion: The ratio 4:5 is less than the ratio 6:7.

Example 3: Converting to Percentages

Problem: Compare the ratios 2:3 and 8:11 by converting them into percentages.

Solution:

1. Convert to Percentages:
   • $frac{2}{3} times 100 = 66.67%$
   • $frac{8}{11} times 100 = 72.72%$
2. Compare Percentages: Since 72.72% > 66.67%, the ratio 8:11 is greater than the ratio 2:3.
3. Conclusion: Therefore, 8:11 > 2:3.

Example 4: Unit Ratio

Problem: The information about the number of math problems solved by three students is given below. Who has solved the maximum number of problems in one minute?

Name of student	Number of math problems	Time (in minutes)
Alex	64	8
Holden	55	5
Jim	39	3

Solution:

1. Find Unit Rate:
   • Alex: $frac{64}{8} = 8$ problems per minute
   • Holden: $frac{55}{5} = 11$ problems per minute
   • Jim: $frac{39}{3} = 13$ problems per minute
2. Compare Unit Rates:
   • Alex: 8 problems/minute
   • Holden: 11 problems/minute
   • Jim: 13 problems/minute
3. Conclusion: Jim has the fastest rate in solving math problems.

These examples illustrate how to apply different methods to compare ratios effectively, providing you with practical skills for various real-world scenarios.

6. Frequently Asked Questions (FAQs) on Comparing Ratios

How can I determine which ratio is greater?

To determine which ratio is greater, use methods such as the LCM method, cross-multiplication, converting to decimals, or converting to percentages. These techniques allow you to compare the numerical values of the ratios directly.

How can we find the ratio of two numbers?

To find the ratio of two numbers, divide one number by the other. The resulting quotient gives you the ratio. For example, to find the ratio of 8 to 4, divide 8 by 4, resulting in a ratio of 2:1.

Can ratios be compared if the quantities have different units?

No, ratios cannot be compared if the quantities have different units. Ensure that the quantities are expressed in the same units before comparing them. For instance, convert both measurements to meters before comparing lengths.

What is the purpose of comparing ratios?

Comparing ratios allows you to see how two or more quantities relate to each other. It helps in making informed decisions, such as determining the best value for a product, optimizing recipes, or analyzing financial performance.

How do I convert a ratio to a percentage?

To convert a ratio to a percentage, express the ratio as a fraction, then multiply the fraction by 100. For example, the ratio 1:4 is equivalent to $frac{1}{4} times 100 = 25%$.

What is a unit ratio, and why is it useful?

A unit ratio is a ratio with a second term equal to 1 (e.g., a:1). It is useful because it simplifies comparison. Converting ratios to unit ratios allows you to compare only the first terms, making it easier to determine which ratio is larger or smaller.

Are ratios used in real-world applications outside of mathematics?

Yes, ratios are used extensively in real-world applications such as business, cooking, science, engineering, and healthcare. They are essential for analyzing data, making informed decisions, and solving problems across various fields.

What should I do if I encounter ratios with very large numbers?

When dealing with ratios with very large numbers, simplify the ratios by dividing both terms by their greatest common divisor (GCD). This reduces the numbers to a more manageable size without changing the ratio’s value.

How does COMPARE.EDU.VN help in comparing ratios?

COMPARE.EDU.VN provides detailed comparisons, benchmark data, and tools to analyze different options, making it easier to understand the relationships between various factors and make informed decisions.

Comparing ratios is a fundamental skill with vast applications. By understanding the different methods and their uses, you can effectively analyze and compare quantities to make well-informed decisions. Whether in business, cooking, science, or daily life, ratios provide valuable insights for better understanding and decision-making.

Are you struggling to compare different options and make informed decisions? Visit COMPARE.EDU.VN for comprehensive and objective comparisons that help you evaluate products, services, and ideas with ease. Our detailed analyses provide clear insights, enabling you to identify the best choices for your needs.

Make confident decisions today with COMPARE.EDU.VN!

Contact us at:

Address: 333 Comparison Plaza, Choice City, CA 90210, United States

WhatsApp: +1 (626) 555-9090

Website: compare.edu.vn