Comparing Integers can sometimes feel like navigating a maze, especially when negative numbers enter the picture. COMPARE.EDU.VN simplifies this process by providing clear explanations, practical examples, and proven strategies for mastering integer comparisons, including the use of inequality symbols and number lines. Discover effective methods to compare integers, understand their order, and confidently solve related problems with our comprehensive guide to integer comparison and ordering.
1. Understanding Integers: A Foundation for Comparison
Integers are whole numbers (not fractions) that can be positive, negative, or zero. Understanding what integers are is crucial before comparing them. Let’s break down the key components:
- Positive Integers: These are whole numbers greater than zero (1, 2, 3, …). They reside to the right of zero on a number line, representing values above zero.
- Negative Integers: These are whole numbers less than zero (-1, -2, -3, …). They reside to the left of zero on a number line, representing values below zero.
- Zero: Zero is an integer, but it is neither positive nor negative. It acts as the dividing line between positive and negative integers on the number line.
The number line is your friend when comparing integers. It provides a visual representation of the order of integers, making it easier to understand their relative values. Remember that numbers increase as you move to the right on the number line and decrease as you move to the left.
2. The Number Line: A Visual Tool for Comparing Integers
The number line is an invaluable tool for understanding and comparing integers. It provides a visual representation that simplifies the concept of ordering and comparing values. Here’s how to use it effectively:
- Visual Representation: The number line displays integers in their natural order, with positive numbers to the right of zero and negative numbers to the left.
- Direction Matters: Numbers increase in value as you move to the right along the number line, and they decrease as you move to the left. This directional property is key to comparing integers.
- Comparing Position: To compare two integers, locate them on the number line. The integer further to the right is the larger value, while the integer further to the left is the smaller value.
For example, consider comparing -3 and 2. On the number line, 2 is to the right of -3, indicating that 2 is greater than -3. Similarly, -5 is to the left of -1, meaning that -5 is less than -1.
Using the number line is particularly helpful when dealing with negative numbers, as it provides a clear visual reference for their relative values.
3. Ordering Integers: From Least to Greatest (and Vice Versa)
Ordering integers involves arranging a set of integers in a specific sequence, either from least to greatest (ascending order) or from greatest to least (descending order). The number line is particularly helpful when ordering integers.
3.1. Ordering From Least to Greatest
To order integers from least to greatest, follow these steps:
- Visualize the Number Line: Imagine a number line extending from negative infinity to positive infinity.
- Locate the Integers: Place each integer in the set on the number line.
- Read from Left to Right: Start from the leftmost point on the number line (representing the smallest value) and move to the right, noting the integers as you encounter them.
Example: Order the following set of integers from least to greatest: -7, 3, -2, 5, -1, 0
- Number Line: Visualize the number line.
- Locate: Place the integers on the number line.
- Order: Reading from left to right, we get: -7, -2, -1, 0, 3, 5
Therefore, the ordered set from least to greatest is: -7, -2, -1, 0, 3, 5.
3.2. Ordering From Greatest to Least
To order integers from greatest to least, the process is reversed:
- Visualize the Number Line: Imagine a number line.
- Locate the Integers: Place each integer on the number line.
- Read from Right to Left: Start from the rightmost point (representing the largest value) and move to the left, noting the integers as you encounter them.
Example: Order the following set of integers from greatest to least: 4, -3, 1, -5, 2, 0
- Number Line: Visualize the number line.
- Locate: Place the integers on the number line.
- Order: Reading from right to left, we get: 4, 2, 1, 0, -3, -5
Therefore, the ordered set from greatest to least is: 4, 2, 1, 0, -3, -5.
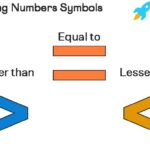
4. Inequality Symbols: Expressing Relationships Between Integers
Inequality symbols are mathematical symbols used to express the relationship between two values. These symbols indicate whether one value is greater than, less than, or equal to another value. Understanding these symbols is crucial for comparing integers effectively.
The primary inequality symbols are:
- > (Greater Than): This symbol indicates that the value on the left is greater than the value on the right. For example, 5 > 3 means “5 is greater than 3.”
- < (Less Than): This symbol indicates that the value on the left is less than the value on the right. For example, -2 < 1 means “-2 is less than 1.”
- = (Equal To): This symbol indicates that the value on the left is equal to the value on the right. For example, 4 = 4 means “4 is equal to 4.”
- ≥ (Greater Than or Equal To): This symbol indicates that the value on the left is greater than or equal to the value on the right. For example, x ≥ 7 means “x is greater than or equal to 7.”
- ≤ (Less Than or Equal To): This symbol indicates that the value on the left is less than or equal to the value on the right. For example, y ≤ -1 means “y is less than or equal to -1.”
Using inequality symbols provides a concise and precise way to express the relationship between integers. It is essential to correctly identify which symbol to use based on the relative values of the integers being compared.
5. Comparing Integers Using Inequality Symbols: Examples
Applying inequality symbols to compare integers requires a solid understanding of the symbols and the number line concept. Here are several examples to illustrate this process:
- Example 1: Compare 7 and 3.
- Since 7 is greater than 3, we use the “greater than” symbol: 7 > 3.
- Example 2: Compare -5 and -1.
- On the number line, -1 is to the right of -5, meaning -1 is greater than -5. Therefore, -5 < -1.
- Example 3: Compare -2 and 4.
- Positive numbers are always greater than negative numbers. So, -2 < 4.
- Example 4: Compare 0 and -6.
- Zero is greater than any negative number. Thus, 0 > -6.
- Example 5: Compare -8 and -8.
- Since both numbers are the same, we use the “equal to” symbol: -8 = -8.
These examples demonstrate how to use inequality symbols to accurately compare integers based on their relative values. Remember to consider the number line and the rules governing positive and negative numbers.
6. Strategies for Comparing Integers: Tips and Tricks
To effectively compare integers, keep these tips and tricks in mind:
- Positive vs. Negative: Positive integers are always greater than negative integers. When comparing a positive and a negative integer, the positive integer is always the larger value.
- Zero as a Reference Point: Zero is greater than all negative integers and less than all positive integers. Use zero as a reference point when comparing integers.
- Number Line Visualization: When comparing two negative integers, remember that the integer closer to zero on the number line is the larger value. For example, -1 is greater than -5.
- Absolute Value: Consider the absolute value of integers when comparing them. The absolute value is the distance of the number from zero. When comparing two negative integers, the one with the smaller absolute value is the larger number. For example, |-3| = 3 and |-5| = 5. Since 3 < 5, -3 > -5.
- Inequality Symbol Direction: Remember that the inequality symbol always points to the smaller number. For example, if 5 > 2, the symbol points to 2, indicating that 2 is the smaller value.
- Practice Regularly: Consistent practice is key to mastering integer comparison. Work through various examples and exercises to reinforce your understanding.
By applying these strategies, you can confidently compare integers and avoid common pitfalls.
7. Common Mistakes to Avoid When Comparing Integers
When comparing integers, it’s easy to make mistakes if you’re not careful. Here are some common pitfalls to avoid:
- Forgetting the Negative Sign: One of the most common mistakes is overlooking the negative sign. Always pay close attention to whether an integer is positive or negative.
- Assuming Larger Absolute Value Means Larger Number: With negative integers, a larger absolute value means a smaller number. For example, -5 has a larger absolute value than -2, but -5 is less than -2.
- Confusing “Greater Than” and “Less Than”: Ensure you understand the difference between the “>” (greater than) and “<” (less than) symbols. Visualize the number line to reinforce the direction each symbol represents.
- Ignoring Zero: Zero is a crucial reference point. Remember that zero is greater than all negative integers and less than all positive integers.
- Not Using the Number Line: The number line is a powerful visual tool. Use it, especially when you’re unsure, to help you compare integers accurately.
- Rushing Through Problems: Take your time and carefully consider each integer before comparing them. Rushing can lead to careless errors.
By being aware of these common mistakes, you can minimize errors and improve your accuracy when comparing integers.
8. Real-World Applications of Comparing Integers
Comparing integers isn’t just a theoretical exercise; it has numerous real-world applications across various fields:
- Finance: Comparing bank account balances (positive for deposits, negative for withdrawals) to determine financial status.
- Temperature: Comparing temperatures above and below zero to understand weather conditions or temperature changes.
- Altitude: Comparing elevations above and below sea level to determine the height or depth of geographical locations.
- Sports: Comparing scores or point differentials in games, where negative values might represent deficits.
- Debt: Comparing debt levels (represented as negative integers) to assets (represented as positive integers) to assess financial health.
- Game Development: Using integers to represent game scores, player positions (e.g., coordinates), and other game mechanics.
- Computer Science: Integers are fundamental in computer programming for representing quantities, indices, and various data structures.
- Science and Engineering: Comparing measurements, such as voltage or current, where negative values indicate opposite directions.
- Everyday Life: Comparing time before and after a specific event, such as “5 minutes before” (-5) versus “10 minutes after” (+10).
Understanding how to compare integers is essential for making informed decisions and interpreting data in various real-world scenarios.
9. Advanced Integer Concepts: Absolute Value and Opposites
To deepen your understanding of integers, it’s important to explore advanced concepts like absolute value and opposites.
9.1. Absolute Value
The absolute value of an integer is its distance from zero on the number line. It is always a non-negative value. The absolute value of a number x is denoted as |x|.
- Examples:
- |5| = 5 (The absolute value of 5 is 5 because it is 5 units away from zero.)
- |-3| = 3 (The absolute value of -3 is 3 because it is 3 units away from zero.)
- |0| = 0 (The absolute value of 0 is 0 because it is 0 units away from zero.)
Absolute value is useful in various contexts, such as determining the magnitude of a quantity regardless of its direction or sign.
9.2. Opposites
The opposite of an integer is the number that is the same distance from zero on the number line but in the opposite direction. The opposite of a number x is denoted as -x.
- Examples:
- The opposite of 7 is -7.
- The opposite of -4 is 4.
- The opposite of 0 is 0.
The sum of an integer and its opposite is always zero. For example, 5 + (-5) = 0.
Understanding absolute value and opposites provides a more complete understanding of integers and their properties.
10. Practice Problems: Testing Your Knowledge of Integer Comparison
To solidify your understanding of comparing integers, here are some practice problems:
- Order the following integers from least to greatest: -12, 5, -3, 0, 8, -1
- Order the following integers from greatest to least: 6, -7, 2, -4, 1, -9
- Compare the following pairs of integers using >, <, or =:
- -5 and 2
- -8 and -3
- 0 and -4
- 7 and 7
- -1 and -6
- What is the absolute value of:
- -15
- 9
- -2
- 0
- What is the opposite of:
- 11
- -6
- -1
- 0
Answer Key:
- -12, -3, -1, 0, 5, 8
- 6, 2, 1, -4, -7, -9
-
- -5 < 2
- -8 < -3
- 0 > -4
- 7 = 7
- -1 > -6
-
- |-15| = 15
- |9| = 9
- |-2| = 2
- |0| = 0
-
- -11
- 6
- 1
- 0
Working through these practice problems will help reinforce your skills in comparing integers and identifying common pitfalls.
11. Resources for Further Learning on Comparing Integers
To continue your learning journey with comparing integers, here are some valuable resources:
- Online Tutorials: Websites like Khan Academy (https://www.khanacademy.org/) offer free lessons and practice exercises on integers and number lines.
- Math Workbooks: Look for algebra or pre-algebra workbooks that cover integers, ordering, and comparing. These workbooks often provide structured lessons and practice problems.
- Educational Videos: YouTube channels dedicated to math education can offer visual explanations and step-by-step solutions to integer-related problems.
- Interactive Games: Online math games can make learning about integers fun and engaging. Search for games that focus on number lines, ordering, and comparing.
- School Resources: If you’re a student, utilize your school’s math resources, such as textbooks, teacher support, and tutoring services.
- Online Forums and Communities: Participate in online math forums or communities where you can ask questions, share your understanding, and learn from others.
By utilizing these resources, you can deepen your understanding of comparing integers and build a solid foundation for more advanced math concepts.
12. The Role of COMPARE.EDU.VN in Simplifying Comparisons
COMPARE.EDU.VN plays a vital role in simplifying complex comparison tasks. While this article focuses on comparing integers, COMPARE.EDU.VN extends its comparison expertise to various domains, providing users with comprehensive and unbiased information to make informed decisions.
At COMPARE.EDU.VN, we understand that making informed choices can be challenging, whether it’s selecting the right educational program, comparing product features, or evaluating service options. That’s why we offer a user-friendly platform where you can easily compare different options side-by-side.
Our team of experts is dedicated to providing accurate, up-to-date, and objective comparisons to help you navigate the complexities of decision-making. We carefully research and analyze a wide range of factors, including features, prices, reviews, and user feedback, to provide you with a comprehensive overview of your choices.
COMPARE.EDU.VN also provides an environment for users to share experiences to help each other reach an informed decision.
Whether you’re comparing integers or exploring other complex decisions, COMPARE.EDU.VN is here to empower you with the information you need to make the best choice for your specific needs.
13. Tips to Remember: A Quick Recap
Before you go, let’s recap the key tips to remember when comparing integers:
- Positive vs. Negative: Positive integers are always greater than negative integers.
- Zero as a Reference: Zero is greater than all negative integers and less than all positive integers.
- Number Line: Visualize the number line to understand the relative positions of integers.
- Absolute Value: Consider the absolute value when comparing negative integers; the smaller the absolute value, the larger the integer.
- Inequality Symbols: Use “>” (greater than), “<” (less than), and “=” (equal to) to express relationships accurately.
- Practice: Practice regularly to reinforce your skills and avoid common mistakes.
- Real-World Context: Apply integer comparison to real-world scenarios to see its practical applications.
By keeping these tips in mind, you’ll be well-equipped to compare integers confidently and accurately.
14. Conclusion: Mastering Integer Comparisons for Success
Mastering the art of comparing integers is more than just an academic exercise; it’s a fundamental skill that translates into various aspects of life. From managing finances to understanding scientific data, the ability to accurately compare integers empowers you to make informed decisions and solve problems effectively.
By understanding the number line, mastering inequality symbols, and avoiding common mistakes, you can confidently navigate the world of integers. Remember to practice regularly and apply your knowledge to real-world scenarios to reinforce your understanding.
COMPARE.EDU.VN is committed to providing you with the resources and guidance you need to succeed in your learning journey. Whether you’re comparing integers or exploring other complex topics, our platform offers comprehensive comparisons and expert insights to help you make informed decisions.
Ready to explore more comparisons and make confident decisions? Visit COMPARE.EDU.VN today and discover a world of valuable insights. Our team is dedicated to providing you with the tools and information you need to succeed.
Contact us:
Address: 333 Comparison Plaza, Choice City, CA 90210, United States
Whatsapp: +1 (626) 555-9090
Website: compare.edu.vn
15. Frequently Asked Questions (FAQ) About Comparing Integers
Here are some frequently asked questions about comparing integers:
- What are integers?
- Integers are whole numbers (not fractions or decimals) that can be positive, negative, or zero.
- How do I compare a positive integer and a negative integer?
- Positive integers are always greater than negative integers.
- How do I compare two negative integers?
- When comparing two negative integers, the integer with the smaller absolute value is the larger number. For example, -2 is greater than -5.
- What is the number line, and how can it help me compare integers?
- The number line is a visual representation of integers. Numbers increase as you move to the right on the number line and decrease as you move to the left. Use it to visualize the relative positions of integers.
- What are inequality symbols, and how are they used?
- Inequality symbols are mathematical symbols used to express the relationship between two values. The primary symbols are “>” (greater than), “<” (less than), and “=” (equal to).
- What is the absolute value of an integer?
- The absolute value of an integer is its distance from zero on the number line. It is always a non-negative value.
- What is the opposite of an integer?
- The opposite of an integer is the number that is the same distance from zero on the number line but in the opposite direction.
- Why is it important to learn how to compare integers?
- Comparing integers is a fundamental skill that translates into various aspects of life, from managing finances to understanding scientific data.
- What are some common mistakes to avoid when comparing integers?
- Common mistakes include forgetting the negative sign, assuming larger absolute value means larger number (for negative integers), and confusing “greater than” and “less than.”
- Where can I find more resources to learn about comparing integers?
- You can find resources online, in math workbooks, through educational videos, and by utilizing school resources.
Understanding these frequently asked questions can further clarify any confusion you may have about comparing integers.