Does A Table Compare The Products And Reactants Of Processes?

A table effectively compares the products and reactants of processes by visually organizing key information, allowing for easy analysis of reaction kinetics, chemical transformations, and equilibrium constants. For comprehensive comparisons and insightful analysis, visit COMPARE.EDU.VN. This facilitates informed decision-making and a deeper understanding of chemical reactions, offering an integrated rate law to simplify complex relationships.

1. Understanding Chemical Change: Thermodynamics vs. Kinetics

Thermodynamics and kinetics play distinct roles in understanding chemical change. Thermodynamics determines whether a reaction can occur spontaneously by examining energy changes, while kinetics investigates how fast a reaction occurs.

• Thermodynamics: Assesses the feasibility of a reaction by evaluating the change in Gibbs free energy (ΔG). A negative ΔG indicates a spontaneous reaction, while a positive ΔG suggests the reaction requires energy input.
• Kinetics: Focuses on the reaction rate and the factors that influence it, such as temperature, concentration, and catalysts. It explains the reaction mechanism and the steps involved in converting reactants to products.

2. Expressing Reaction Rates from Balanced Equations

Given a balanced chemical equation, the rate of reaction can be expressed in terms of the change in concentration of reactants or products over time.

For a reaction like:

aA + bB → cC + dD

The rate can be expressed as:

Rate = – (1/a) d[A]/dt = – (1/b) d[B]/dt = (1/c) d[C]/dt = (1/d) d[D]/dt

Here:

• d[A]/dt and d[B]/dt represent the rate of disappearance of reactants A and B.
• d[C]/dt and d[D]/dt represent the rate of appearance of products C and D.
• The coefficients a, b, c, and d are the stoichiometric coefficients from the balanced equation, ensuring the rate is consistent regardless of which species is monitored.

3. Visualizing Reaction Rates Over Time

A graph illustrating the instantaneous rate of a reaction over time typically shows a decreasing rate as reactants are consumed.

• Initial Rate: The rate is highest at the beginning when reactant concentrations are maximal.
• Decreasing Rate: As the reaction proceeds, reactant concentrations decrease, leading to a slower reaction rate.
• Equilibrium: Eventually, the rate approaches zero as the reaction nears equilibrium, where the forward and reverse rates are equal.

4. Determining Reaction Order from Experimental Data

For a reaction A → B + C, the order of the reaction can be determined from experimental data by observing how the concentration of products changes with time.

• Zero Order: The rate is independent of the concentration of A. A plot of [A] vs. time is linear.
• First Order: The rate is directly proportional to the concentration of A. A plot of ln[A] vs. time is linear.
• Second Order: The rate is proportional to the square of the concentration of A. A plot of 1/[A] vs. time is linear.

By analyzing the linearity of these plots, the reaction order can be determined.

5. Initial Rate and Isolation Methods for Multi-Reactant Reactions

In reactions with multiple reactants, determining the individual orders involves using the initial rate method and the isolation method.

• Initial Rate Method: Measure the initial rate of the reaction for different initial concentrations of each reactant while keeping others constant. The order with respect to each reactant can then be determined from the change in rate.
• Isolation Method: Keep the concentration of all reactants except one in large excess. The reaction order with respect to the reactant at non-excess concentrations can then be determined.

6. Differential vs. Integral Rate Laws

Differential and integral rate laws provide different perspectives on reaction kinetics.

• Differential Rate Law: Expresses the rate of a reaction in terms of the concentrations of reactants. It describes the instantaneous rate at any given time.

  Rate = k[A]^m[B]^n

• Integral Rate Law: Relates the concentration of reactants or products to time. It describes how the concentration changes over a period.

  • First Order: ln[A]t = -kt + ln[A]0
  • Second Order: 1/[A]t = kt + 1/[A]0

7. Concentration Changes in First-Order Reactions

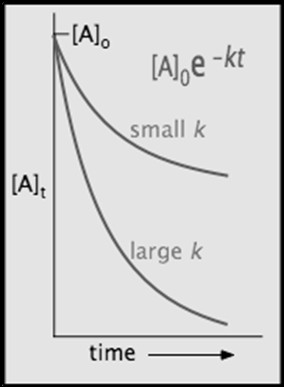
For a first-order reaction, a plot of ln[A] versus time yields a straight line with a negative slope equal to the rate constant k.

• Rate Constant Magnitude: A larger k indicates a faster reaction, resulting in a steeper slope. Conversely, a smaller k indicates a slower reaction and a shallower slope.
• Concentration Decay: The concentration of A decreases exponentially over time, following the equation [A]t = [A]0 * e^(-kt).

8. Defining Half-Life in Chemical Reactions

The half-life of a reaction is the time required for the concentration of a reactant to decrease to half of its initial value.

• First-Order Reactions: The half-life (t1/2) is constant and related to the rate constant (k) by the equation t1/2 = 0.693/k.
• Second-Order Reactions: The half-life depends on the initial concentration of the reactant and is given by t1/2 = 1/(k[A]0).
• Zero-Order Reactions: The half-life depends on the initial concentration of the reactant and is given by t1/2 = [A]0 / (2k).

9. Calculating Concentration After Multiple Half-Lives

Given the half-life for a first-order reaction and the initial concentration [A]0, the concentration [A]t after n half-lives can be calculated using the formula:

[A]t = [A]0 * (1/2)^n

For example, after 3 half-lives, the concentration will be:

[A]t = [A]0 * (1/2)^3 = [A]0 / 8

10. Zero-Order Reactions: Conditions and Characteristics

A reaction can appear to have an order of zero when the rate is independent of the concentration of the reactant under specific conditions.

• Catalysis: When a reaction is catalyzed by a solid surface or an enzyme, the rate may become independent of the reactant concentration if the catalyst is saturated.
• Excess Reactants: If one reactant is present in large excess, the change in its concentration during the reaction is negligible, making the reaction appear zero-order with respect to that reactant.

11. Integrating the Rate Law: A Closer Look

Integrating the rate law allows us to determine how reactant and product concentrations change over time. The integral rate laws differ based on the reaction order.

• Zero-Order: [A]t = [A]0 – kt
• First-Order: ln([A]t/[A]0) = -kt
• Second-Order: 1/[A]t = 1/[A]0 + kt

These integrated rate laws enable predictions of reactant and product concentrations at any point during the reaction.

12. Defining Reaction Completion

Defining the point at which a reaction is “completed” depends on the context and desired outcome.

• Equilibrium: A reaction is completed when it reaches equilibrium, and the concentrations of reactants and products no longer change.
• Reactant Depletion: In simpler cases, a reaction is considered complete when a reactant is entirely consumed, particularly when the equilibrium constant is very large.
• Arbitrary Fraction: For practical purposes, “completed” might be defined as when a reactant concentration falls to a specific fraction of its initial value (e.g., 90%, 70%).

13. Practical Considerations for Reaction Completion

Selecting a specific fraction to define reaction completion balances the cost of reactants against the value of products and the operational costs of running the process.

• Industrial Processes: In industrial settings, the cost of raw materials, energy consumption, and the value of the final product are all factors in determining the optimal point to consider the reaction “complete.”
• Economic Factors: The decision hinges on whether the incremental gain from converting more reactants into products justifies the additional time and resources required.

14. First-Order Reactions and Exponential Change

The consumption of a reactant in a first-order process follows the law of exponential change. This law is prevalent in various scientific and real-world scenarios.

• Exponential Decay: Describes the decrease in reactant concentration over time, radioactive decay, and other diminishing processes.
• Exponential Growth: Describes the increase in money in a continuously compounding bank account or the population growth of reproducing organisms.

15. The Integrated Rate Law for First-Order Reactions

The integrated rate law for a first-order reaction A → products is given by:

[A]t = [A]0 * e^(-kt)

Where:

• [A]t is the concentration of A at time t
• [A]0 is the initial concentration of A
• k is the first-order rate constant
• e is the base of the natural logarithm

This equation shows that the concentration of A decreases exponentially over time.

16. Linearizing First-Order Reactions

Taking the natural logarithm of the integrated rate law for a first-order reaction yields a linear equation:

ln[A]t = -kt + ln[A]0

This equation is in the form of a straight line, y = mx + b, where:

• y = ln[A]t
• m = -k (the slope)
• x = t (time)
• b = ln[A]0 (the y-intercept)

Plotting ln[A]t versus time gives a straight line with a slope of -k.

17. Half-Life and First-Order Reactions

After one half-life (t1/2), the concentration of the reactant A is half of its initial concentration:

[A](t1/2) = [A]0 / 2

Using the integrated rate law:

ln([A]0 / 2) = -kt1/2 + ln[A]0

Solving for t1/2:

t1/2 = ln(2) / k ≈ 0.693 / k

This shows that the half-life of a first-order reaction is constant and independent of the initial concentration.

18. Inverse Relationship Between Rate Constant and Half-Life

The rate constant (k) and the half-life (t1/2) of a first-order process are inversely related:

k = 0.693 / t1/2

A larger rate constant indicates a shorter half-life, meaning the reaction proceeds faster. Conversely, a smaller rate constant indicates a longer half-life, meaning the reaction proceeds slower.

19. Example: Calculating Rate Constant from Half-Life

If the half-life of a first-order reaction is 10 minutes, the rate constant can be calculated as:

k = 0.693 / (10 min) = 0.0693 min-1

To convert this to reciprocal seconds:

k = 0.0693 min-1 * (1 min / 60 s) = 0.001155 s-1

Thus, the rate constant is approximately 0.001155 s-1.

20. Radioactive Decay as a First-Order Process

Radioactive decay is always a first-order process. The rate at which radioactive nuclei decay is proportional to the number of nuclei present.

• Half-Life: Each radioactive isotope has a characteristic half-life, which is the time it takes for half of the nuclei in a sample to decay.
• Decay Constant: The decay constant (λ) is related to the half-life by the equation λ = 0.693 / t1/2.

21. Americium-241: Half-Life Applications

Americium-241 (Am241), used in smoke detectors, has a half-life of 432 years. We can calculate various properties based on this half-life.

• Fraction Decayed After 50 Years:

  1. Calculate the decay constant: λ = 0.693 / 432 years = 0.001604 years-1
  2. Use the equation: [A]t = [A]0 * e^(-λt)
  3. [A]t / [A]0 = e^(-0.001604 * 50) = e^(-0.0802) ≈ 0.923
  4. Fraction decayed = 1 – 0.923 = 0.077 or 7.7%

• Time for Activity to Decline to 80%:

  1. [A]t / [A]0 = 0.80
  2. 0. 80 = e^(-λt)
  3. ln(0.80) = -λt
  4. t = ln(0.80) / -0.001604 = -0.223 / -0.001604 ≈ 139 years

• Seventh-Life of Am241: The “seventh-life” refers to the time it takes for the activity to decline to 1/7 of its initial value.

  1. 1/7 = e^(-λt)
  2. ln(1/7) = -λt
  3. t = ln(1/7) / -0.001604 = -1.946 / -0.001604 ≈ 1213 years

22. Second-Order Reactions and Integration

The integration of the second-order rate law:

-d[A]/dt = k[A]^2

Yields:

1/[A] = 1/[A]0 + kt

This equation can be rearranged into the form of a straight line, where a plot of 1/[A] versus time will give a straight line with a slope of k and a y-intercept of 1/[A]0.

23. Half-Life of Second-Order Reactions

The half-life for a second-order reaction is given by:

t1/2 = 1 / (k[A]0)

Unlike first-order reactions, the half-life of a second-order reaction depends on the initial concentration of the reactant. As the reaction proceeds and [A]0 decreases, the half-life increases.

24. Zero-Order Processes and Rate Independence

In zero-order reactions, the rate is independent of the reactant concentration:

Rate = k[A]^0 = k

This implies that the rate of the reaction is constant regardless of the amount of reactant present.

25. Integrated Rate Law for Zero-Order Reactions

Integrating the differential rate law for a zero-order reaction yields:

[A]t = [A]0 – kt

This equation shows that the concentration of A decreases linearly with time.

26. Conditions Leading to Zero-Order Rates

Zero-order kinetics typically arises under specific conditions:

• Catalysis: Reactions catalyzed by solid surfaces or enzymes can exhibit zero-order kinetics when the catalyst is saturated.
• Excess Reactants: When one or more reactants are in large excess, the reaction rate may appear independent of their concentrations.

27. Surface Catalysis and Zero-Order Reactions

In surface catalysis, the reaction rate can become zero-order when all active sites on the catalyst surface are occupied.

For example, the decomposition of nitrous oxide (N2O) on a hot platinum wire:

N2O(g) → N2(g) + ½ O2(g)

The reaction is zero-order because the rate is limited by the number of N2O molecules adsorbed on the platinum surface.

28. Enzyme-Catalyzed Reactions and Zero-Order Kinetics

Enzyme-catalyzed reactions can appear zero-order when the enzyme is saturated with substrate. The reaction rate depends only on the enzyme concentration and the rate at which the enzyme can process the substrate.

29. Pseudo-Zero-Order Reactions

When a reaction involves multiple reactants, and one is present in great excess, the reaction may appear zero-order with respect to the reactant in excess. These are referred to as pseudo-zero-order reactions.

30. Comprehensive Rate Law Table

A table compares the rate parameters of zero-, first-, and second-order reactions:

Order	Differential Rate Law	Integrated Rate Law	Half-Life	Plot for Straight Line
Zero	Rate = k	[A] = [A]0 – kt	[A]0 / (2k)	[A] vs. t
First	Rate = k[A]	ln([A]0/[A]) = kt	0.693 / k	ln [A] vs. t
Second	Rate = k[A]^2	1/[A] – 1/[A]0 = kt	1 / (k[A]0)	1/[A] vs. t

This table simplifies the comparison of key characteristics for different reaction orders.

31. Applications of Understanding Reaction Kinetics

Understanding reaction kinetics is crucial in various fields, including:

• Chemical Engineering: Designing and optimizing chemical reactors.
• Pharmaceuticals: Determining drug stability and reaction rates in drug synthesis.
• Environmental Science: Studying the rates of pollutant degradation.
• Materials Science: Understanding material degradation and synthesis processes.

32. Factors Affecting Reaction Rates

Several factors can affect reaction rates:

• Temperature: Higher temperatures generally increase reaction rates.
• Concentration: Higher reactant concentrations usually increase reaction rates.
• Catalysts: Catalysts speed up reactions by lowering the activation energy.
• Surface Area: For heterogeneous reactions, a larger surface area increases the reaction rate.
• Pressure: For gas-phase reactions, pressure can affect reaction rates.

33. Techniques for Measuring Reaction Rates

Various techniques are used to measure reaction rates:

• Spectrophotometry: Measuring the change in absorbance of reactants or products.
• Conductometry: Measuring the change in conductivity of the reaction mixture.
• Titration: Measuring the concentration of reactants or products by titration.
• Gas Chromatography: Separating and quantifying gaseous reactants or products.
• Mass Spectrometry: Identifying and quantifying reactants and products based on their mass-to-charge ratio.

34. Transition State Theory

Transition state theory explains reaction rates based on the formation of an activated complex or transition state.

• Activation Energy: The energy required to form the transition state.
• Frequency Factor: Relates to the frequency of collisions and the probability of successful reactions.
• Arrhenius Equation: Describes the temperature dependence of reaction rates: k = A * e^(-Ea/RT)

35. Collision Theory

Collision theory states that for a reaction to occur, reactant molecules must collide with sufficient energy and proper orientation.

• Effective Collisions: Collisions that result in a reaction.
• Activation Energy: The minimum energy required for a collision to be effective.
• Orientation Factor: The fraction of collisions with the correct orientation for a reaction to occur.

36. Catalysis and Reaction Mechanisms

Catalysts provide an alternative reaction pathway with a lower activation energy, thus increasing the reaction rate.

• Homogeneous Catalysis: The catalyst is in the same phase as the reactants.
• Heterogeneous Catalysis: The catalyst is in a different phase from the reactants.
• Enzyme Catalysis: Enzymes are biological catalysts that facilitate specific biochemical reactions.

37. Reaction Mechanisms and Elementary Steps

A reaction mechanism is a series of elementary steps that describe how a reaction occurs.

• Elementary Step: A single step in a reaction mechanism.
• Rate-Determining Step: The slowest step in the mechanism, which determines the overall reaction rate.
• Intermediates: Species that are formed and consumed during the reaction but do not appear in the overall balanced equation.

38. Steady-State Approximation

The steady-state approximation assumes that the concentration of an intermediate remains constant during the reaction. This approximation simplifies the analysis of complex reaction mechanisms.

39. Chain Reactions

Chain reactions involve a series of steps in which reactive intermediates (radicals) are continuously generated and consumed.

• Initiation: The step that produces radicals.
• Propagation: Steps that involve the reaction of radicals with other molecules to produce more radicals.
• Termination: Steps that consume radicals and stop the chain reaction.

40. Photochemical Reactions

Photochemical reactions are initiated by the absorption of light. The energy of the light photons provides the activation energy needed to start the reaction.

41. Importance of Stoichiometry in Rate Expressions

Stoichiometry plays a vital role in expressing reaction rates accurately. The rate of disappearance of reactants and the rate of appearance of products are related through the stoichiometric coefficients in the balanced chemical equation.

42. Complex Reactions and Their Analysis

Complex reactions involve multiple steps and intermediates, making their analysis more challenging. Techniques such as the steady-state approximation, pre-equilibrium approximation, and computational modeling are used to study these reactions.

43. Temperature Dependence of Reaction Rates: Arrhenius Equation

The Arrhenius equation quantifies the temperature dependence of reaction rates:

k = A * e^(-Ea/RT)

Where:

• k is the rate constant
• A is the frequency factor
• Ea is the activation energy
• R is the gas constant
• T is the absolute temperature

This equation highlights that reaction rates increase exponentially with temperature.

44. Advanced Kinetic Models

Advanced kinetic models, such as those based on computational chemistry and molecular dynamics simulations, provide detailed insights into reaction mechanisms and rates. These models can predict reaction behavior under various conditions.

45. Applications in Environmental Chemistry

Understanding reaction kinetics is crucial in environmental chemistry for studying the fate and transport of pollutants. Reaction rates determine how quickly pollutants degrade and transform in the environment.

46. Applications in Biochemistry

In biochemistry, enzyme kinetics plays a central role in understanding metabolic pathways and enzyme function. Michaelis-Menten kinetics and other models are used to analyze enzyme-catalyzed reactions.

47. Industrial Applications and Reactor Design

Reaction kinetics is essential in industrial applications for reactor design and optimization. Understanding reaction rates and mechanisms allows engineers to design efficient reactors that maximize product yield and minimize waste.

48. Current Research and Future Directions

Current research in reaction kinetics focuses on developing new catalysts, understanding complex reaction mechanisms, and creating more accurate predictive models. Future directions include the use of artificial intelligence and machine learning to accelerate the discovery of new reactions and catalysts.

49. Case Studies: Applying Kinetic Principles

Examining case studies from various fields illustrates the practical application of kinetic principles. These examples provide valuable insights into how kinetics is used to solve real-world problems.

50. Further Exploration of Chemical Kinetics

Further exploration of chemical kinetics involves delving into advanced topics such as non-elementary reactions, oscillating reactions, and stochastic kinetics. These areas provide a deeper understanding of complex chemical systems.

Ready to dive deeper and make informed decisions? Visit COMPARE.EDU.VN today to explore comprehensive comparisons and insightful analysis. Our team of experts is dedicated to providing you with the tools you need to succeed. Whether you’re comparing chemical transformations, evaluating reaction kinetics, or assessing equilibrium constants, COMPARE.EDU.VN is your go-to resource. Contact us at 333 Comparison Plaza, Choice City, CA 90210, United States. Reach out via Whatsapp at +1 (626) 555-9090 or visit our website compare.edu.vn for more information.

FAQ: Understanding Reaction Rates and Kinetics

1. What is the difference between reaction rate and rate constant?

Reaction rate refers to the speed at which a chemical reaction occurs, typically measured as the change in concentration of reactants or products per unit time. The rate constant (k) is a proportionality constant that relates the reaction rate to the concentrations of reactants.

2. How does temperature affect the rate of a reaction?

Generally, increasing the temperature increases the rate of a reaction. This is because higher temperatures provide more energy, leading to more frequent and energetic collisions between reactant molecules, thus increasing the likelihood of successful reactions. The Arrhenius equation quantifies this relationship.

3. What is activation energy?

Activation energy (Ea) is the minimum energy required for a chemical reaction to occur. It represents the energy barrier that reactant molecules must overcome to transform into products. Catalysts lower the activation energy, thereby increasing the reaction rate.

4. What is a catalyst, and how does it affect reaction kinetics?

A catalyst is a substance that speeds up a chemical reaction without being consumed in the process. Catalysts provide an alternative reaction pathway with a lower activation energy, making it easier for the reaction to occur.

5. How do you determine the order of a reaction experimentally?

The order of a reaction can be determined experimentally using methods such as the initial rate method and the isolation method. These methods involve measuring the reaction rate for different initial concentrations of reactants and analyzing how the rate changes with concentration.

6. What is a rate-determining step?

In a multi-step reaction mechanism, the rate-determining step is the slowest step. This step limits the overall rate of the reaction. Identifying the rate-determining step is crucial for understanding and optimizing reaction kinetics.

7. What is the Michaelis-Menten kinetics model?

The Michaelis-Menten kinetics model describes the rate of enzyme-catalyzed reactions. It relates the reaction rate to the substrate concentration and enzyme properties, such as the Michaelis constant (Km) and the maximum reaction rate (Vmax).

8. How does a heterogeneous catalyst work?

A heterogeneous catalyst is in a different phase from the reactants. The reaction typically occurs on the surface of the catalyst. Reactant molecules adsorb onto the surface, undergo reaction, and then desorb as products. The surface area of the catalyst is an important factor in determining the reaction rate.

9. What is a reaction mechanism?

A reaction mechanism is a detailed step-by-step description of how a chemical reaction occurs. It includes all elementary steps, intermediates, and transition states involved in the transformation of reactants to products.

10. What are some applications of studying reaction kinetics in real life?

Studying reaction kinetics has numerous applications, including:

• Designing and optimizing chemical reactors
• Developing new catalysts
• Understanding drug stability and metabolism
• Studying environmental pollutant degradation
• Improving industrial processes and product yields