A Ratio Comparing Two Quantities With Different Kinds Of Units is known as a rate, and COMPARE.EDU.VN is here to break down this essential concept. Understanding rates is crucial for solving everyday problems, from calculating speed to determining the best deals. Let’s explore this concept in detail, focusing on practical applications and clear explanations.
1. What is a Rate in Mathematics?
In mathematics, a rate is a specialized type of ratio that compares two quantities measured in different units. This means that the numerator and denominator of the rate represent distinct types of measurements. Common examples of rates include speed (miles per hour), price (dollars per pound), and flow rate (liters per minute). The “per” in these expressions indicates the division between the two quantities. Rates are fundamental in various real-world applications, from calculating fuel efficiency to understanding economic indicators. COMPARE.EDU.VN provides tools and resources to help you master these concepts.
2. Understanding Unit Rate
A unit rate is a specific type of rate where the denominator is always one. It simplifies comparisons by expressing the quantity of the numerator for a single unit of the denominator. For instance, if a car travels 120 miles in 2 hours, the unit rate is 60 miles per hour (120 miles / 2 hours = 60 miles/hour). This tells you the distance covered in one hour. Unit rates are widely used to compare prices, speeds, and other measures, making them easier to understand and contrast. Determining the best value when shopping is often done by calculating and comparing unit rates.
3. Exploring the Definition of Ratio
A ratio is a comparison of two quantities that have the same units. It can be expressed as a fraction, using a colon, or with the word “to”. For example, if there are 12 apples and 16 oranges, the ratio of apples to oranges is 12:16, which simplifies to 3:4. Ratios are useful for understanding proportions and relationships between quantities. Unlike rates, ratios compare quantities of the same kind, making them essential in fields like finance and cooking. Understanding the difference between rates and ratios is fundamental in mathematics and everyday problem-solving.
4. Key Differences Between Rate and Ratio
Rate and ratio are related concepts, but they differ significantly in their application and meaning. The main difference lies in the units of the quantities being compared. Here’s a breakdown:
| Feature | Rate | Ratio |
|---|---|---|
| Unit Type | Different units (e.g., miles and hours) | Same units (e.g., apples and oranges) |
| Purpose | Measures change or comparison over time or units | Compares quantities of the same type |
| Examples | Miles per hour, price per pound | 3:4 ratio of apples to oranges |
| Representation | Fraction with different units | Fraction, colon, or “to” |
| Use Cases | Speed, price, flow rate | Proportions, mixtures, scaling |

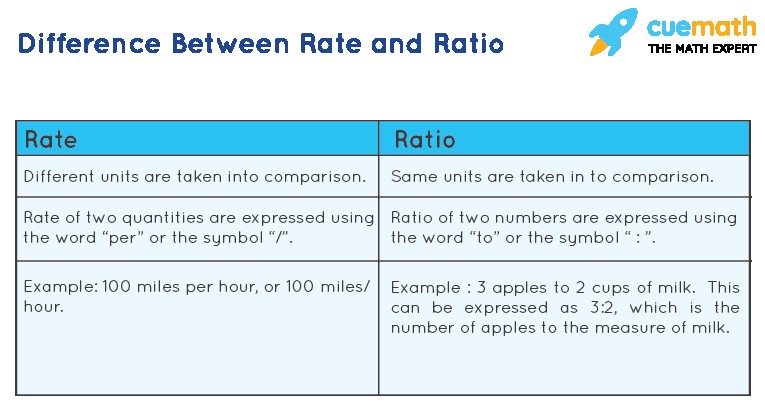
Rates are used to measure how one quantity changes relative to another with different units, while ratios compare quantities of the same kind. Recognizing this distinction is crucial for accurate problem-solving in mathematics and practical scenarios.
5. Common Examples of Rates in Everyday Life
Rates are ubiquitous in everyday life. Here are some common examples:
- Speed: Miles per hour (mph) or kilometers per hour (km/h)
- Price: Dollars per pound ($/lb) or euros per kilogram (€/kg)
- Wage: Dollars per hour ($/hr)
- Flow Rate: Liters per minute (L/min) or gallons per minute (gal/min)
- Heart Rate: Beats per minute (bpm)
- Typing Speed: Words per minute (wpm)
- Fuel Efficiency: Miles per gallon (mpg) or liters per 100 kilometers (L/100km)
These examples illustrate how rates are used to measure and compare different quantities in various contexts. Understanding rates helps in making informed decisions, from driving efficiently to managing personal finances.
6. How to Calculate a Rate: A Step-by-Step Guide
Calculating a rate involves several straightforward steps. Here’s a guide:
- Identify the Quantities: Determine the two quantities you need to compare.
- Note the Units: Ensure the quantities have different units.
- Set up the Ratio: Write the rate as a fraction with the first quantity as the numerator and the second quantity as the denominator.
- Simplify: Reduce the fraction to its simplest form.
- Include Units: Always include the units in your final answer.
Example:
Suppose a car travels 300 miles in 5 hours.
- Quantities: Distance (300 miles) and Time (5 hours)
- Units: Miles and hours (different units)
- Ratio: 300 miles / 5 hours
- Simplify: 60 miles / 1 hour
- Answer: 60 miles per hour (60 mph)
This systematic approach ensures accurate calculation and clear communication of rates.
7. Delving into Time Rate: Measuring Change Over Time
Time rate is a specific type of rate that measures how a quantity changes over time. It is expressed as the amount of change per unit of time. Examples include:
- Speed: Distance traveled per unit of time (e.g., miles per hour)
- Growth Rate: Increase in population per year
- Interest Rate: Percentage of principal earned per year
- Depreciation Rate: Decrease in value per year
Time rates are crucial in fields like physics, economics, and finance, where understanding how things change over time is essential. Whether it’s tracking the speed of a vehicle or the growth of an investment, time rates provide valuable insights.
8. Cost Price Rate: Evaluating Value for Money
Cost price rate is used to compare the cost of different items based on their quantity or weight. It is expressed as the cost per unit. Examples include:
- Price per Pound: Cost of food items like fruits or vegetables
- Price per Ounce: Cost of liquids like juice or soda
- Price per Item: Cost of individual products like pens or notebooks
Cost price rates help consumers make informed purchasing decisions by allowing them to compare the value of different products. For example, comparing the price per ounce of different brands of juice can reveal which offers the best value.
9. Understanding Literacy Rate as a Statistical Measure
Literacy rate is a statistical measure that represents the percentage of a population that can read and write. It is an important indicator of a country’s education level and overall development. Literacy rate is typically calculated by dividing the number of literate individuals by the total population and multiplying by 100. This rate helps governments and organizations assess the effectiveness of educational programs and identify areas that need improvement. High literacy rates are generally associated with better health outcomes, economic growth, and social progress.
10. Exploring Population Rate: Dynamics of Population Change
Population rate measures how a population changes over a specific period. It includes birth rates, death rates, immigration rates, and emigration rates. These rates are typically expressed as the number of events per 1,000 people per year. Population rates are essential for understanding demographic trends, planning public services, and addressing issues related to population growth or decline. Analyzing population rates can help predict future population sizes and inform policy decisions related to healthcare, education, and infrastructure.
11. Formula for Rate: Expressing Rate Mathematically
The formula for rate is a simple mathematical expression that defines rate as the ratio of two quantities with different units. It is written as:
Rate = Quantity 1 / Quantity 2
Where:
- Quantity 1 is the amount of one item
- Quantity 2 is the amount of a different item
This formula is versatile and can be applied to various situations, such as calculating speed, price per unit, or flow rate. Understanding and using this formula is fundamental for solving rate-related problems in mathematics and real-world scenarios.
12. Steps to Calculate Rate Effectively
To calculate rate effectively, follow these steps:
- Identify the Quantities: Determine the two quantities that need to be compared and ensure they have different units.
- Write the Ratio: Express the rate as a fraction with the first quantity in the numerator and the second quantity in the denominator.
- Simplify the Fraction: Reduce the fraction to its simplest form by dividing both the numerator and denominator by their greatest common factor.
- Include Units: Always include the appropriate units in your answer to provide context and meaning.
- Interpret the Result: Understand what the calculated rate means in the context of the problem.
By following these steps, you can accurately calculate and interpret rates in various situations.
13. Unit Rate Formula: Simplifying Comparisons
The unit rate formula is a variation of the rate formula used to find the amount of one quantity per single unit of another quantity. It is expressed as:
Unit Rate = Quantity 1 / 1 Unit of Quantity 2
This formula simplifies comparisons by ensuring the denominator is always one. For example, if you want to find the price per ounce of a product, you would divide the total price by the number of ounces. The resulting unit rate allows you to easily compare the value of different products.
14. Miles per Minute: A Unit Rate Example
“Miles per minute” is a unit rate that expresses the distance traveled in miles for every minute. To calculate miles per minute, divide the total distance traveled by the total number of minutes. For example, if a car travels 120 miles in 2 hours (120 minutes), the unit rate is:
120 miles / 120 minutes = 1 mile per minute
This unit rate is useful for understanding speed in a more granular way, especially in situations where time is measured in minutes rather than hours.
15. Applying Revolutions per Minute in Practical Scenarios
Revolutions per minute (RPM) is a unit rate that measures the number of complete rotations a rotating object makes in one minute. It is commonly used to measure the speed of engines, motors, and other rotating machinery. RPM is calculated by counting the number of revolutions and dividing by the number of minutes. For example, if a motor completes 3000 revolutions in one minute, its RPM is 3000. Understanding RPM is crucial for optimizing performance and preventing damage to mechanical systems.
16. Salary per Month: Understanding Income Rate
Salary per month is a rate that expresses the amount of money earned in one month. It is calculated by dividing the annual salary by 12 (the number of months in a year). For example, if a person earns $60,000 per year, their salary per month is:
$60,000 / 12 = $5,000 per month
This rate is essential for budgeting, financial planning, and comparing income levels.
17. Frequency per Minute: Measuring Repetitive Events
Frequency per minute measures the number of times an event occurs in one minute. It is commonly used in various fields, including healthcare (e.g., heart rate), manufacturing (e.g., production rate), and telecommunications (e.g., data transmission rate). To calculate frequency per minute, count the number of occurrences of the event and divide by the number of minutes. For example, if a heart beats 72 times in one minute, the frequency is 72 beats per minute.
18. Simple Interest Rate: Understanding Financial Returns
Simple interest rate is the percentage of the principal amount that is paid by a borrower to a lender on an annual basis. It is calculated using the formula:
Simple Interest = Principal x Rate x Time
Where:
- Principal is the initial amount of money borrowed or invested
- Rate is the annual interest rate (expressed as a decimal)
- Time is the number of years the money is borrowed or invested
For example, if a person borrows $1,000 at a simple interest rate of 5% for 3 years, the simple interest earned is:
$1,000 x 0.05 x 3 = $150
Simple interest rate is a fundamental concept in finance, used to calculate the cost of borrowing money or the return on an investment.
19. Defining Rate in Mathematical Terms
In mathematical terms, a rate is defined as a ratio that compares two quantities with different units. It is typically expressed as a fraction, with the first quantity as the numerator and the second quantity as the denominator. The rate can be simplified to its lowest terms to provide a clear and concise comparison. Rates are used extensively in various mathematical and scientific applications to measure and compare different quantities.
20. Steps to Express Rate Effectively in Lowest Form
To express a rate effectively in its lowest form, follow these steps:
- Write the Rate: Express the rate as a fraction with the appropriate units.
- Find the Greatest Common Factor (GCF): Determine the greatest common factor of the numerator and denominator.
- Divide: Divide both the numerator and the denominator by the GCF.
- Simplify: Ensure the fraction is in its simplest form.
- Include Units: Always include the units in your final answer.
Example:
Express the rate 60 miles / 2 hours in its lowest form.
- Rate: 60 miles / 2 hours
- GCF: The greatest common factor of 60 and 2 is 2.
- Divide: 60 ÷ 2 = 30, 2 ÷ 2 = 1
- Simplify: 30 miles / 1 hour
- Answer: 30 miles per hour
Expressing rates in their lowest form makes them easier to understand and compare.
21. Rate vs. Percentage: Understanding the Key Differences
Rate and percentage are related concepts, but they are used in different contexts and have distinct meanings. Here are the key differences:
| Feature | Rate | Percentage |
|---|---|---|
| Definition | Ratio of two quantities with different units | Ratio or rate out of 100 |
| Units | Different units (e.g., miles per hour) | Percentage (%) |
| Use Cases | Speed, price, flow rate | Discounts, interest rates, proportions |
| Representation | Fraction with different units | Number followed by % symbol |
Rates compare quantities with different units, while percentages express a proportion or ratio as a fraction of 100. Understanding these differences is essential for accurate interpretation and application in various scenarios.
22. Three Common Examples of Rate in Real-World Scenarios
Here are three common examples of rate in real-world scenarios:
- Speed: A car traveling at 60 miles per hour (mph) is an example of rate, measuring the distance covered in a unit of time.
- Price: The cost of gasoline at $3.50 per gallon is a rate, comparing the price to the quantity.
- Heart Rate: A person’s heart beating at 72 beats per minute (bpm) is a rate, measuring the frequency of heartbeats in a unit of time.
These examples illustrate how rates are used to measure and compare different quantities in various everyday situations.
23. Rate vs. Unit Rate: Key Distinctions
Rate and unit rate are related concepts, but they have distinct characteristics. Here are the key differences:
| Feature | Rate | Unit Rate |
|---|---|---|
| Definition | Ratio of two quantities with different units | Ratio of a quantity to one unit of another |
| Denominator | Can be any value | Always 1 |
| Purpose | Compares two quantities | Simplifies comparison to a single unit |
| Examples | 120 miles / 2 hours | 60 miles per hour |
The key distinction is that unit rate always has a denominator of 1, making it easier to compare different rates.
24. Solved Examples: Applying Rate Definitions in Problems
Let’s look at some solved examples to understand how to apply rate definitions in practical problems:
Example 1: A printer prints 120 pages in 2 minutes. Find the unit rate of the number of pages printed per minute.
- Solution: Unit Rate = 120 pages / 2 minutes = 60 pages per minute
Example 2: A baker bakes 48 cookies in 4 hours. Find the rate of baking cookies per hour.
- Solution: Rate = 48 cookies / 4 hours = 12 cookies per hour
Example 3: A family travels 600 miles and uses 30 gallons of gas. Calculate the average number of miles per gallon.
- Solution: Average = 600 miles / 30 gallons = 20 miles per gallon
These examples illustrate how to apply the concept of rate to solve real-world problems.
25. Practice Questions: Testing Your Understanding of Rates
Here are some practice questions to test your understanding of rates:
- A car travels 250 miles in 5 hours. What is the average speed in miles per hour?
- A store sells oranges at $2.40 for 6 oranges. What is the price per orange?
- A factory produces 1500 units in 8 hours. What is the production rate per hour?
Try solving these questions to reinforce your understanding of rates and unit rates. Check your answers against the solutions provided to ensure you’re on the right track.
26. FAQs: Addressing Common Questions About Rate Definition
Here are some frequently asked questions about rate definition:
What is the definition of rate?
- Rate is a ratio that compares two quantities with different units.
What is unit rate definition?
- Unit rate is a ratio that compares the first quantity to one unit of the second quantity.
What is simple interest rate definition?
- Simple interest rate is the percentage of the principal amount paid by a borrower to a lender on an annual basis.
What is the definition of rate in math?
- Rate in math is the ratio of two quantities with different units, usually expressed as a fraction.
What is the difference between a rate and a percentage?
- Rate compares two quantities with different units, while percentage expresses a proportion as a fraction of 100.
What are three examples of rate?
- Speed (miles per hour), price (dollars per pound), and heart rate (beats per minute).
What is the difference between rate and unit rate?
- Rate is the ratio of two different quantities, while unit rate expresses the amount of one quantity for one unit of another quantity.
27. Need More Help? Visit COMPARE.EDU.VN for Comprehensive Comparisons
If you’re looking for more detailed explanations and comparisons, visit COMPARE.EDU.VN. Our website offers comprehensive resources to help you understand and apply various concepts, including rates, ratios, and unit rates. We provide detailed examples, practice questions, and step-by-step solutions to help you master these topics. Whether you’re a student, professional, or someone simply looking to improve your understanding, COMPARE.EDU.VN is your go-to resource.
28. Contact Us for More Information
For additional information or assistance, please contact us:
- Address: 333 Comparison Plaza, Choice City, CA 90210, United States
- WhatsApp: +1 (626) 555-9090
- Website: COMPARE.EDU.VN
Our team is here to help you with your questions and provide the support you need.
29. Conclusion: Mastering Rates for Everyday Applications
Understanding rates is essential for solving everyday problems and making informed decisions. By mastering the concepts and calculations related to rates, you can improve your problem-solving skills and make better choices in various areas of life. Whether it’s calculating the best deals, understanding financial returns, or analyzing statistical measures, rates provide valuable insights. Visit COMPARE.EDU.VN for more resources and comparisons to help you on your journey to mastering rates.
30. Call to Action: Explore More at COMPARE.EDU.VN
Ready to dive deeper into the world of comparisons? Visit COMPARE.EDU.VN today to explore a wide range of articles, guides, and tools that will help you make informed decisions. Whether you’re comparing products, services, or ideas, our website provides the resources you need to succeed. Don’t make decisions in the dark – let COMPARE.EDU.VN light your way.
Understanding ‘a ratio comparing two quantities with different kinds of units’ empowers you to make informed decisions across various aspects of life. COMPARE.EDU.VN is committed to providing you with the tools and knowledge necessary to navigate these comparisons effectively. Explore our comprehensive guides, detailed analyses, and practical examples to enhance your understanding and decision-making skills. Embrace the power of informed choices with COMPARE.EDU.VN, your trusted resource for all things comparison.
This detailed guide aims to clarify the concept of ‘a ratio comparing two quantities with different kinds of units’ and its applications. COMPARE.EDU.VN is dedicated to offering clear, comprehensive, and reliable information to help you make informed decisions. By exploring the resources available on our website, you can gain a deeper understanding of various topics and confidently navigate the complexities of modern life. Visit compare.edu.vn today and unlock the power of informed decision-making.