A Ratio Comparing Two Different Units: Rate Explained

A Ratio Comparing Two Different Units, often referred to as a “rate,” is a fundamental concept in mathematics and everyday life. At COMPARE.EDU.VN, we aim to clarify this concept, providing a comprehensive understanding of its definition, applications, and significance. By exploring real-world examples and practical calculations, we’ll empower you to confidently navigate situations involving rates. Discover clarity and make informed decisions with our comprehensive guide on unit comparisons and relational values.

1. What is a Rate in Mathematics?

In mathematics, a rate is defined as a ratio that compares two quantities of different units. This comparison expresses how much of one quantity exists for each unit of another. Unlike a simple ratio that compares like quantities, a rate involves distinct units, such as miles per hour, dollars per pound, or words per minute. The key characteristic of a rate is the presence of different units, making it a powerful tool for describing and analyzing various real-world phenomena. The rate effectively establishes the relationship between two distinct values, providing a measure of change or quantity per unit.

2. Understanding Unit Rate

A unit rate is a specific type of rate where the second quantity is reduced to one unit. This simplifies the comparison and allows for easy understanding of the relationship between the two quantities. For instance, if a car travels 240 miles in 4 hours, the rate is 240 miles/4 hours. The unit rate, however, would be 60 miles per hour (240 miles / 4 hours = 60 miles/hour). This means the car travels 60 miles for every 1 hour. Finding the unit rate involves dividing both quantities by the second quantity until it equals one. Unit rates are particularly useful for making quick comparisons and understanding the value of one item relative to a single unit of another.

3. Exploring the Definition of Ratio

A ratio is a comparison of two or more quantities that have the same units. It indicates how much of one quantity there is compared to another. Ratios can be expressed in several ways: as a fraction (e.g., 1/2), with a colon (e.g., 1:2), or using the word “to” (e.g., 1 to 2). Unlike rates, ratios compare quantities with identical units, allowing for a straightforward comparison of their relative sizes. For example, the ratio of apples to oranges in a basket could be 3:2, meaning there are three apples for every two oranges. Ratios help in understanding proportions and relative amounts within a set of quantities.

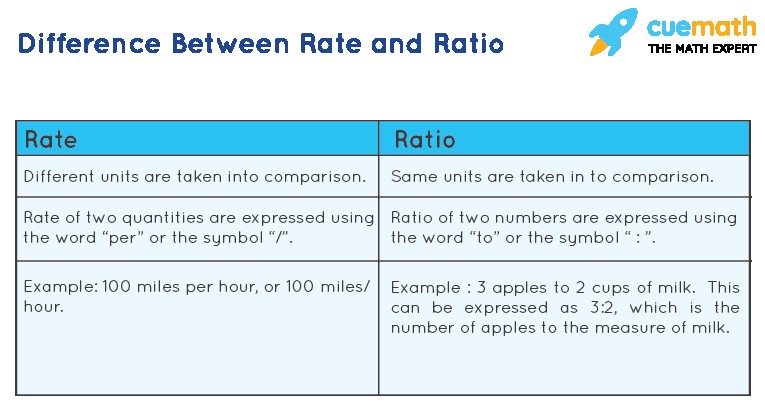
4. Rate vs. Ratio: Key Differences

While both rates and ratios involve comparing quantities, the key difference lies in the units being compared. A rate compares two quantities with different units, whereas a ratio compares two quantities with the same units. Here’s a breakdown of the distinctions:

Units: Rates involve different units (e.g., miles and hours), while ratios involve the same units (e.g., apples and apples).
Purpose: Rates describe how much of one quantity exists for each unit of another, while ratios show the relative size of two or more quantities.
Examples: Examples of rates include speed (miles per hour) and price (dollars per pound). Examples of ratios include the proportion of ingredients in a recipe or the comparison of heights between two people.

Understanding these differences is crucial for correctly interpreting and applying these concepts in various mathematical and real-world scenarios.

5. Real-World Examples of Rates

Rates are ubiquitous in everyday life, playing a crucial role in making informed decisions and understanding various phenomena. Here are some common examples:

Speed: Measured in miles per hour (mph) or kilometers per hour (km/h), speed is a rate that compares distance traveled to the time taken.
Price: Expressed as dollars per pound ($/lb) or euros per kilogram (€/kg), price compares the cost of an item to its weight or quantity.
Fuel Efficiency: Measured in miles per gallon (mpg) or liters per 100 kilometers (L/100km), fuel efficiency compares the distance a vehicle can travel to the amount of fuel it consumes.
Typing Speed: Words per minute (WPM) is a rate that measures how quickly someone can type.
Heart Rate: Beats per minute (BPM) measures the number of times the heart beats in one minute.

These examples demonstrate the versatility of rates in quantifying and comparing different aspects of our daily experiences.

6. Calculating Rates: A Step-by-Step Guide

Calculating a rate involves a straightforward process of dividing one quantity by another. Here’s a step-by-step guide:

Step 1: Identify the Two Quantities

Determine the two quantities you want to compare, ensuring they have different units.

Step 2: Write the Rate as a Fraction

Express the rate as a fraction, with the first quantity as the numerator and the second quantity as the denominator.

Step 3: Simplify the Fraction

Simplify the fraction to its simplest form, if possible, by dividing both the numerator and denominator by their greatest common factor.

Step 4: Include the Units

Write the units of both quantities next to the numerical value to clearly indicate the rate.

Example:

Suppose you drive 150 miles in 3 hours.

Identify the Quantities: Distance = 150 miles, Time = 3 hours
Write the Rate as a Fraction: 150 miles / 3 hours
Simplify the Fraction: 50 miles / 1 hour
Include the Units: 50 miles per hour (mph)

7. How to Calculate Unit Rate

Calculating a unit rate is a specific application of rate calculation, where the goal is to express the rate in terms of one unit of the second quantity. Here’s how to do it:

Step 1: Identify the Rate

Determine the rate you want to convert to a unit rate.

Step 2: Divide by the Denominator

Divide both the numerator and the denominator of the rate by the original denominator. This will make the denominator equal to one.

Step 3: Include the Units

Write the units of both quantities next to the numerical value to clearly indicate the unit rate.

Example:

Suppose you earn $480 for working 40 hours.

Identify the Rate: $480 / 40 hours
Divide by the Denominator: ($480 / 40) / (40 hours / 40) = $12 / 1 hour
Include the Units: $12 per hour

8. Common Formulas Involving Rates

Several formulas utilize rates to solve common problems. Here are a few important ones:

Speed, Distance, Time:
- Speed = Distance / Time
- Distance = Speed × Time
- Time = Distance / Speed
Cost per Unit:
- Cost per Unit = Total Cost / Number of Units
Work Rate:
- Work Rate = Amount of Work / Time

These formulas are essential tools for solving a wide range of practical problems involving rates.

9. Solved Examples: Putting Rate Calculations into Practice

Let’s walk through some examples to illustrate how to calculate and apply rates in different scenarios.

Example 1: Calculating Speed

A train travels 450 miles in 5 hours. What is its average speed?

Speed = Distance / Time
Speed = 450 miles / 5 hours
Speed = 90 miles per hour

Example 2: Finding Cost per Unit

A box of 24 apples costs $12. What is the cost per apple?

Cost per Unit = Total Cost / Number of Units
Cost per Unit = $12 / 24 apples
Cost per Unit = $0.50 per apple

Example 3: Determining Work Rate

John can complete a task in 6 hours. What is his work rate?

Work Rate = Amount of Work / Time
Work Rate = 1 task / 6 hours
Work Rate = 1/6 task per hour

10. Practice Problems to Sharpen Your Skills

To solidify your understanding of rates, try solving these practice problems:

A car travels 325 miles in 5 hours. Calculate its average speed.
A store sells 5 pounds of oranges for $8. What is the cost per pound?
Sarah can type 600 words in 12 minutes. Find her typing speed in words per minute.
A factory produces 1500 units in 8 hours. What is the production rate per hour?
If you earn $675 for working 45 hours, what is your hourly wage?

Check your answers by applying the formulas and steps discussed earlier.

11. Tips for Solving Rate-Related Problems

Solving rate-related problems can be made easier by following these tips:

Read Carefully: Understand the problem statement and identify the quantities and units involved.
Use the Correct Formula: Choose the appropriate formula based on the given information and what you need to find.
Keep Track of Units: Always include units in your calculations to ensure accuracy and consistency.
Simplify When Possible: Simplify fractions and rates to make calculations easier.
Double-Check Your Work: Verify your answers to ensure they make sense in the context of the problem.

12. Advanced Applications of Rates

Beyond basic calculations, rates are used in more complex applications, such as:

Economics: Analyzing economic growth rates, inflation rates, and unemployment rates.
Finance: Calculating interest rates, investment returns, and loan repayments.
Science: Determining reaction rates in chemistry, growth rates in biology, and speed of light in physics.
Engineering: Designing systems based on flow rates, data transfer rates, and production rates.

These advanced applications highlight the importance of understanding rates in various professional fields.

13. How Rates Impact Decision-Making

Rates play a crucial role in making informed decisions in both personal and professional contexts. For example:

Budgeting: Understanding interest rates on loans and credit cards helps in making sound financial decisions.
Shopping: Comparing prices per unit allows for cost-effective purchasing decisions.
Travel Planning: Calculating travel time based on speed and distance helps in efficient trip planning.
Business Management: Analyzing production rates and cost per unit aids in optimizing business operations.

By understanding and utilizing rates, individuals and businesses can make more informed and strategic decisions.

14. The Significance of Rates in Scientific Measurements

In scientific measurements, rates are essential for quantifying and analyzing various phenomena. Examples include:

Reaction Rates: Measuring how quickly chemical reactions occur.
Growth Rates: Assessing the rate at which populations or organisms increase in size.
Decay Rates: Determining the rate at which radioactive substances decay.
Flow Rates: Measuring the volume of fluid that flows through a pipe or channel per unit time.

These rates are critical for conducting experiments, analyzing data, and drawing meaningful conclusions in scientific research.

15. Maximizing Efficiency Using Rate Analysis

Rate analysis can be used to maximize efficiency in various processes. For example:

Manufacturing: Optimizing production rates to increase output and reduce costs.
Transportation: Improving fuel efficiency and reducing travel time to minimize expenses.
Healthcare: Enhancing patient care by improving the rate of diagnosis and treatment.
Education: Boosting learning outcomes by optimizing the rate of knowledge transfer.

By analyzing and improving rates, organizations can achieve significant gains in efficiency and productivity.

16. Common Mistakes to Avoid When Working with Rates

When working with rates, it’s important to avoid common mistakes that can lead to inaccurate results:

Mixing Units: Ensure all quantities are expressed in consistent units before performing calculations.
Incorrect Formulas: Use the correct formula based on the given information and what you need to find.
Misinterpreting Rates: Understand the meaning of the rate and what it represents in the context of the problem.
Ignoring Context: Consider the context of the problem and whether the rate is constant or variable.
Rounding Errors: Avoid rounding intermediate values, as this can lead to significant errors in the final answer.

17. Utilizing Technology for Rate Calculations

Technology can greatly simplify rate calculations. Calculators, spreadsheets, and specialized software can quickly perform complex calculations and analyses. Online tools and apps are also available for calculating rates and converting units. By leveraging these technological resources, you can save time and improve accuracy in rate-related tasks.

18. Tips for Remembering Rate Formulas

Remembering rate formulas can be easier with these tips:

Understand the Concept: Focus on understanding the underlying concept of the rate rather than just memorizing the formula.
Use Mnemonics: Create memorable phrases or acronyms to help you recall the formulas.
Practice Regularly: Solve practice problems to reinforce your understanding and memory of the formulas.
Relate to Real-World Examples: Connect the formulas to real-world examples to make them more meaningful and easier to remember.
Create Flashcards: Use flashcards to quiz yourself on the formulas and their applications.

19. How to Teach Rates to Students

Teaching rates to students can be effective by using these strategies:

Start with Real-World Examples: Introduce rates with relatable examples from everyday life.
Use Visual Aids: Employ diagrams, charts, and graphs to help students visualize rates and their relationships.
Hands-On Activities: Engage students in hands-on activities that involve measuring and calculating rates.
Problem-Solving Exercises: Provide plenty of problem-solving exercises to reinforce their understanding and skills.
Relate to Other Concepts: Connect rates to other mathematical concepts, such as ratios, proportions, and percentages.

20. The Future of Rate Analysis in Technology

The future of rate analysis in technology is promising, with advancements in data analytics, machine learning, and artificial intelligence. These technologies can enable more sophisticated and accurate rate calculations, leading to better insights and decision-making. Real-time rate analysis, predictive modeling, and automated optimization are just a few of the potential applications of these technologies. As technology continues to evolve, rate analysis will become an even more powerful tool for understanding and managing complex systems.

21. Exploring Different Types of Rates

Rates come in various forms, each suited for different applications:

Average Rate: The overall rate over a period of time or a range of values.
Instantaneous Rate: The rate at a specific point in time.
Constant Rate: A rate that remains the same over time.
Variable Rate: A rate that changes over time.
Relative Rate: A rate expressed as a percentage or proportion of another rate.

Understanding these different types of rates allows for more nuanced analysis and interpretation.

22. Addressing Common Misconceptions About Rates

Common misconceptions about rates include:

Confusing Rates and Ratios: Believing that rates and ratios are the same thing.
Ignoring Units: Neglecting to include units in rate calculations.
Using Incorrect Formulas: Applying the wrong formula for the given problem.
Misinterpreting Results: Drawing incorrect conclusions from rate calculations.
Overlooking Context: Failing to consider the context of the problem and the factors that may affect the rate.

Addressing these misconceptions is essential for ensuring accurate and meaningful rate analysis.

23. Incorporating Real-World Data into Rate Problems

Incorporating real-world data into rate problems can make them more engaging and relevant. Use data from news articles, scientific studies, or personal experiences to create realistic scenarios. This approach can help students and professionals see the practical applications of rates and develop their problem-solving skills.

24. How Rates Connect to Other Mathematical Concepts

Rates are closely connected to other mathematical concepts, such as:

Ratios: Rates are a specific type of ratio that compares quantities with different units.
Proportions: Rates can be used to set up and solve proportions.
Percentages: Rates can be expressed as percentages to show relative changes or proportions.
Linear Equations: Rates can be used to model linear relationships and solve linear equations.
Calculus: Rates are fundamental to the concepts of derivatives and integrals in calculus.

Understanding these connections can deepen your overall understanding of mathematics.

25. The Role of Rates in Financial Planning

Rates play a vital role in financial planning. Interest rates, inflation rates, and investment returns are all rates that can significantly impact your financial well-being. Understanding these rates can help you make informed decisions about saving, investing, and managing debt.

26. Understanding Rates in Healthcare

In healthcare, rates are used to measure and analyze various aspects of patient care, such as:

Mortality Rate: The number of deaths per unit of population.
Morbidity Rate: The number of cases of a disease per unit of population.
Recovery Rate: The proportion of patients who recover from a disease or condition.
Readmission Rate: The proportion of patients who are readmitted to the hospital within a certain period.

These rates are essential for monitoring public health, evaluating the effectiveness of treatments, and improving patient outcomes.

27. Rates in Environmental Science

Environmental science relies heavily on rates to study and manage environmental processes, such as:

Deforestation Rate: The rate at which forests are being cleared.
Pollution Rate: The rate at which pollutants are being released into the environment.
Biodiversity Loss Rate: The rate at which species are becoming extinct.
Climate Change Rate: The rate at which the Earth’s climate is changing.

These rates are crucial for understanding the impacts of human activities on the environment and developing strategies for sustainable development.

28. The Use of Rates in Sports Analytics

In sports analytics, rates are used to measure and analyze various aspects of athletic performance, such as:

Speed: The rate at which an athlete moves over a certain distance.
Accuracy: The rate at which an athlete successfully completes a task, such as shooting a basketball or throwing a baseball.
Efficiency: The rate at which an athlete converts opportunities into points or successes.
Injury Rate: The rate at which athletes sustain injuries.

These rates are valuable for evaluating player performance, developing training strategies, and making informed decisions about team composition and tactics.

29. Rates and Their Impact on Supply Chain Management

Rates are critical in supply chain management for optimizing logistics and operations. Key rates include:

Production Rate: The speed at which goods are manufactured.
Inventory Turnover Rate: The frequency at which inventory is sold and replaced.
Delivery Rate: The speed at which goods are delivered to customers.
Defect Rate: The percentage of products that are defective.

Managing these rates effectively ensures efficient supply chains, reduces costs, and improves customer satisfaction.

30. Resources for Further Learning About Rates

To further your understanding of rates, consider these resources:

Online Courses: Platforms like Coursera, Khan Academy, and Udemy offer courses on mathematics and related topics.
Textbooks: Consult textbooks on algebra, calculus, and statistics for in-depth explanations and examples.
Websites: Websites like COMPARE.EDU.VN, MathWorld, and Wolfram Alpha provide definitions, formulas, and calculators.
Tutoring: Seek help from a math tutor or instructor for personalized guidance and support.
Practice Problems: Solve practice problems regularly to reinforce your understanding and skills.

Conclusion: The Power of Understanding Rates

Understanding rates is essential for navigating various aspects of life, from personal finance to scientific research. By mastering the concepts and calculations discussed in this article, you can make more informed decisions, solve complex problems, and achieve your goals. Visit COMPARE.EDU.VN for more in-depth comparisons and tools to assist in your decision-making process. Whether you’re comparing products, services, or ideas, our platform provides the insights you need to make the best choice.

Are you ready to make smarter comparisons? Visit COMPARE.EDU.VN today and discover the power of informed decision-making. Our platform offers comprehensive comparisons across a wide range of products and services, helping you find the best options to suit your needs and budget. Don’t make a decision without checking COMPARE.EDU.VN first! Contact us at 333 Comparison Plaza, Choice City, CA 90210, United States, or reach out via Whatsapp at +1 (626) 555-9090.

Frequently Asked Questions (FAQs) About Rates

1. What is the definition of a rate?

A rate is a ratio that compares two quantities of different units, expressing how much of one quantity exists for each unit of another.

2. How does a unit rate differ from a regular rate?

A unit rate is a rate where the second quantity is reduced to one unit, simplifying the comparison.

3. Can you provide examples of rates used in everyday life?

Examples include speed (miles per hour), price (dollars per pound), and fuel efficiency (miles per gallon).

4. What are some common mistakes to avoid when calculating rates?

Mistakes include mixing units, using incorrect formulas, and misinterpreting the rate’s meaning.

5. How can technology assist in calculating and analyzing rates?

Calculators, spreadsheets, and specialized software can quickly perform complex calculations and analyses.

6. Why is it important to understand rates in financial planning?

Understanding rates such as interest rates and investment returns helps in making informed financial decisions.

7. What role do rates play in environmental science?

Rates are used to study and manage environmental processes, such as deforestation and pollution.

8. How are rates utilized in sports analytics?

Rates measure and analyze athletic performance, such as speed, accuracy, and efficiency.

9. In what ways do rates impact supply chain management?

Rates optimize logistics and operations by managing production, inventory turnover, and delivery.

10. Where can I find additional resources for learning about rates?

Online courses, textbooks, websites like compare.edu.vn, and tutoring services provide valuable resources for further learning.