A Rate Is a Special Ratio That Compares Quantities

Unlock the power of rates! This guide from COMPARE.EDU.VN explores how A Rate Is A Special Ratio That Compares quantities, offering clarity and practical applications for everyday life. Discover how understanding rates can simplify comparisons and inform your decisions.

1. Understanding the Definition of Rate in Mathematics

In mathematics, a rate is a special ratio that compares two distinct quantities that are measured in different units. Essentially, it tells you how much of one thing there is compared to another, but with the added dimension of differing units. For example, when you talk about speed, you’re comparing distance (miles or kilometers) to time (hours or minutes). This comparison, expressed as “miles per hour” or “kilometers per minute,” is a rate.

The key characteristic of a rate is the difference in the units being compared. This distinguishes it from a simple ratio, which compares quantities with the same units (more on ratios later). Rates are fundamental in various real-world applications, from calculating fuel efficiency to understanding population growth. Mastering the concept of rate is crucial for informed decision-making and problem-solving in numerous fields. Rate of change is a critical aspect when observing phenomena over time.

2. Delving into the Concept of Unit Rate

The a rate is a special ratio that compares, the unit rate takes this a step further by standardizing the comparison. A unit rate expresses how much of one quantity exists for a single unit of another quantity. In simpler terms, the denominator of the rate is always 1. This standardization makes comparing different rates much easier.

Consider the example of grocery shopping. You might see two different brands of cereal with different prices and sizes. To determine which is the better deal, you can calculate the unit rate (price per ounce or gram). This allows you to directly compare the cost of one unit of cereal, regardless of the package size. Unit rates are incredibly useful in everyday life, enabling you to make informed decisions when purchasing goods, services, or even evaluating job offers (salary per year or hour). Understanding the unit rate helps you to evaluate value accurately.

3. Exploring the Definition of a Ratio

To fully grasp what a rate is a special ratio that compares, it’s important to understand what a ratio itself is. A ratio is a comparison of two or more quantities. These quantities must be of the same kind, meaning they share the same units. Ratios can be expressed in several ways:

Using a colon: 3:4 (read as “3 to 4”)
As a fraction: 3/4
Using the word “to”: 3 to 4

For example, if a classroom has 15 boys and 20 girls, the ratio of boys to girls is 15:20, which can be simplified to 3:4. This means for every 3 boys, there are 4 girls. Ratios are used extensively in recipes, scale models, and probability calculations. Unlike rates, ratios deal with comparing quantities within the same unit of measurement. Scale ratios are especially important in map reading.

4. Rate vs. Ratio: Understanding the Key Differences

While a rate is a special ratio that compares, it’s not the same as a ratio. The crucial difference lies in the units being compared.

Feature	Rate	Ratio
Units	Different units	Same units
Comparison	Compares quantities with different units	Compares quantities with the same units
Example	Miles per hour (distance/time)	Ratio of apples to oranges (apples/oranges)
Simplification	May not always be simplified to a whole number	Often simplified to the lowest terms
Common Uses	Speed, price per unit, growth rate	Recipes, scale models, probability

Caucasian Kid and Caucasian Teacher Grade 8 10

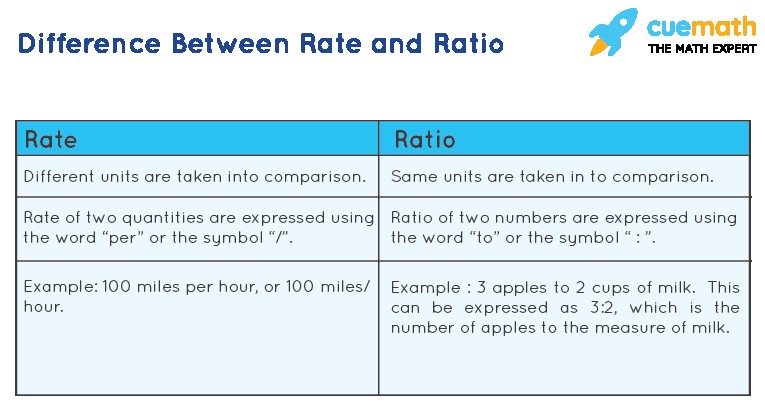
Understanding this distinction is vital for applying the correct mathematical concepts to real-world problems. Confusing rates and ratios can lead to incorrect calculations and flawed decision-making. Remember, the key is to identify whether the quantities being compared have the same units or different units.

5. Examples of Rates in Everyday Life

A rate is a special ratio that compares quantities is evident in numerous daily scenarios. Here are a few examples to illustrate the concept:

Speed: As mentioned earlier, speed is a classic example of a rate, measuring the distance traveled per unit of time (e.g., 60 miles per hour).
Price per Unit: When shopping, you often compare the price per unit (e.g., dollars per pound) to determine the best value.
Heart Rate: Your heart rate, measured in beats per minute (bpm), is a rate that reflects how many times your heart beats in a minute.
Click-Through Rate (CTR): In online advertising, CTR measures the number of clicks an ad receives per number of impressions (e.g., 2 clicks per 100 impressions).
Fuel Efficiency: Measured in miles per gallon (mpg) or liters per 100 kilometers (L/100km), fuel efficiency tells you how far a vehicle can travel on a given amount of fuel.
Exchange Rates: Exchange rates, such as USD to EUR, determine how much of one currency you receive for another.

These examples demonstrate the wide range of applications where rates are used to compare quantities and make informed decisions. Recognizing rates in everyday situations can help you analyze information more effectively.

6. Calculating Rates: A Step-by-Step Guide

Calculating a rate is a special ratio that compares two quantities is a straightforward process. Here’s a step-by-step guide:

Identify the Two Quantities: Determine the two quantities you want to compare and their respective units. For instance, distance (miles) and time (hours).
Set Up the Ratio: Express the relationship between the two quantities as a ratio, with the first quantity as the numerator and the second quantity as the denominator. In our example, this would be miles/hours.
Simplify the Ratio (if possible): Divide both the numerator and denominator by their greatest common factor to simplify the ratio.
Express the Rate with Units: Write the simplified ratio with the appropriate units. For example, if you travel 120 miles in 2 hours, the rate is 60 miles/hour or 60 miles per hour.

Example:

A factory produces 500 widgets in 8 hours. Calculate the production rate.

Quantities: Widgets (500) and Time (8 hours)
Ratio: 500 widgets / 8 hours
Simplify: 62.5 widgets / 1 hour
Rate: 62.5 widgets per hour

This step-by-step approach makes calculating rates accessible and ensures accurate results.

7. Calculating Unit Rates: Finding the Value of One

Calculating a unit rate builds upon the process of calculating a rate. The key difference is that you need to manipulate the ratio so that the denominator is equal to 1. Here’s how:

Identify the Rate: Start with the rate you want to convert to a unit rate (e.g., 24 dollars for 6 gallons).
Divide by the Denominator: Divide both the numerator and the denominator by the original denominator. In our example, divide both 24 dollars and 6 gallons by 6.
Express the Unit Rate: The resulting ratio will have a denominator of 1, representing the unit rate (e.g., 4 dollars per gallon).

Example:

A store sells a 12-pack of soda for 6 dollars. Calculate the unit rate (price per can).

Rate: 6 dollars / 12 cans
Divide: (6 dollars / 12) / (12 cans / 12)
Unit Rate: 0.50 dollars per can (or 50 cents per can)

Unit rate calculations provide a standardized way to compare values and identify the best deals.

8. Real-World Applications: Using Rates and Unit Rates to Make Informed Decisions

A rate is a special ratio that compares quantities is used extensively to empower informed decision-making in various aspects of life.

Shopping: Comparing unit prices to find the best value for groceries, household items, and other products.
Finance: Calculating interest rates on loans or investments to understand the true cost or return.
Travel: Determining the fastest route based on speed limits and distances, or comparing fuel efficiency of different vehicles.
Health: Monitoring heart rate during exercise to ensure you’re in the optimal training zone, or tracking calorie intake per day to manage your weight.
Business: Analyzing sales growth rate to assess business performance, or calculating production rate to optimize manufacturing processes.
Education: Calculating the rate of learning or progress in a specific subject to identify areas that need more attention.

By understanding and applying the concepts of rates and unit rates, you can make more informed and effective decisions in all areas of your life. Rates are key in performance metrics across industries.

9. Common Mistakes to Avoid When Working with Rates

When working with a rate is a special ratio that compares quantities, several common mistakes can lead to inaccurate results:

Mixing Up Units: Ensure that you are using consistent units throughout your calculations. For example, if you’re calculating speed, make sure you’re using either miles per hour or kilometers per hour, not a combination of both.
Incorrectly Setting Up the Ratio: Double-check that you have the quantities in the correct order in the ratio. If you’re calculating price per unit, make sure the price is in the numerator and the quantity is in the denominator.
Forgetting to Simplify: Always simplify the ratio to its lowest terms to make the rate easier to understand and compare.
Misinterpreting Unit Rates: Remember that a unit rate always has a denominator of 1. Make sure you’ve correctly calculated the unit rate before making any decisions based on it.
Not Considering Other Factors: While rates and unit rates are valuable tools, they don’t always tell the whole story. Consider other factors, such as quality, brand reputation, and personal preferences, before making a final decision.

Avoiding these common mistakes will help you ensure accuracy and make sound judgments when working with rates.

10. Advanced Applications: Rates in Science and Engineering

While a rate is a special ratio that compares is commonplace, rates are fundamental concepts in various scientific and engineering disciplines.

Chemistry: Reaction rates measure how quickly a chemical reaction occurs.
Physics: Velocity, acceleration, and flow rate are all examples of rates used to describe motion and fluid dynamics.
Biology: Population growth rates and metabolic rates are used to study the dynamics of living organisms.
Engineering: Rates of change are used to model and control systems, such as temperature control in a chemical reactor or speed control in a vehicle.
Economics: Inflation rate, unemployment rate, and economic growth rate are used to assess the health of an economy.

These advanced applications demonstrate the versatility and importance of rates in understanding and modeling complex phenomena. The principles of rate calculation remain consistent, but the context and interpretation become more sophisticated.

11. Rates and Proportions: Understanding the Relationship

Rates are closely related to proportions. A proportion is an equation that states that two ratios (or rates) are equal. Proportions are often used to solve problems involving scaling and conversions.

For example, if you know that the rate of exchange between USD and EUR is 1.10 (1 USD = 1.10 EUR), you can use a proportion to calculate how many EUR you would receive for a given amount of USD.

Let x be the amount of EUR you would receive for 100 USD.

The proportion would be:

1 USD / 1.10 EUR = 100 USD / x EUR

Solving for x, you get:

x = 110 EUR

Proportions provide a powerful tool for solving problems involving rates and ratios, particularly when dealing with scaling or conversions.

12. Tips for Mastering Rate Calculations

Mastering a rate is a special ratio that compares requires practice and attention to detail. Here are some tips to help you improve your skills:

Practice Regularly: Work through various examples and problems involving rates and unit rates.
Pay Attention to Units: Always include units in your calculations and make sure they are consistent.
Visualize the Problem: Try to visualize the scenario described in the problem to better understand the relationship between the quantities.
Break Down Complex Problems: Break down complex problems into smaller, more manageable steps.
Check Your Work: Always check your work to ensure that your calculations are accurate.
Use Online Resources: Utilize online calculators and tutorials to reinforce your understanding and practice your skills.
Seek Help When Needed: Don’t hesitate to ask for help from teachers, tutors, or classmates if you’re struggling with the concepts.

By following these tips and dedicating time to practice, you can master rate calculations and apply them effectively in various situations.

13. Case Study: Comparing Mobile Phone Plans Using Rates

Let’s consider a practical example of how a rate is a special ratio that compares quantities can be used to make informed decisions. Suppose you’re choosing between two mobile phone plans:

Plan A: 50 dollars per month for 10 GB of data.
Plan B: 60 dollars per month for 15 GB of data.

To determine which plan offers the best value, calculate the unit rate (price per GB):

Plan A: 50 dollars / 10 GB = 5 dollars/GB
Plan B: 60 dollars / 15 GB = 4 dollars/GB

Based on the unit rate, Plan B offers a better value at 4 dollars per GB compared to Plan A’s 5 dollars per GB. However, you should also consider your data usage. If you typically use less than 10 GB per month, Plan A might be more cost-effective, even though the unit rate is higher.

This case study illustrates how rates and unit rates can be used to compare options and make informed decisions based on your individual needs and circumstances.

14. Tools and Resources for Calculating Rates

Numerous tools and resources are available to help you calculate rates and unit rates:

Calculators: Online calculators can quickly perform rate and unit rate calculations.
Spreadsheet Software: Programs like Microsoft Excel and Google Sheets can be used to create custom rate calculators and analyze data.
Mobile Apps: Many mobile apps are designed to help you with rate calculations and unit conversions.
Online Tutorials: Websites like Khan Academy and YouTube offer free tutorials on rates, ratios, and proportions.
Textbooks and Workbooks: Math textbooks and workbooks provide comprehensive explanations and practice problems.

These resources can help you learn the concepts and practice your skills in a variety of ways.

15. The Importance of Understanding Rates in a Data-Driven World

In today’s data-driven world, understanding a rate is a special ratio that compares quantities is more important than ever. Data is everywhere, and being able to interpret and analyze it effectively is a crucial skill.

Rates are used to:

Track trends and patterns
Compare performance
Make predictions
Identify areas for improvement
Evaluate the effectiveness of interventions

Whether you’re analyzing financial data, tracking website traffic, or monitoring health metrics, understanding rates is essential for extracting meaningful insights and making informed decisions.

16. Rates and Financial Literacy: Managing Your Money Wisely

Understanding a rate is a special ratio that compares quantities is fundamental to financial literacy and managing your money wisely. Here are some examples:

Interest Rates: Understanding interest rates on loans and credit cards is crucial for making informed borrowing decisions.
Investment Returns: Calculating the rate of return on investments helps you assess their performance.
Inflation Rate: Monitoring the inflation rate helps you understand how the purchasing power of your money changes over time.
Exchange Rates: Understanding exchange rates is essential for international travel and transactions.
Tax Rates: Understanding tax rates helps you plan your finances and minimize your tax liability.

By developing your financial literacy and understanding rates, you can make informed decisions about saving, investing, and managing your money effectively.

17. How COMPARE.EDU.VN Can Help You Master Rates and Comparisons

COMPARE.EDU.VN is your ultimate resource for understanding and applying the concept of a rate is a special ratio that compares in various contexts. We offer comprehensive comparisons, detailed analyses, and practical tools to help you make informed decisions.

Whether you’re comparing products, services, or ideas, COMPARE.EDU.VN provides you with the information you need to make the right choice. Our platform offers:

Detailed Comparisons: Side-by-side comparisons of products and services, highlighting key features, benefits, and drawbacks.
Expert Reviews: In-depth reviews from industry experts, providing valuable insights and recommendations.
User Ratings and Reviews: Feedback from real users, giving you a balanced perspective on different options.
Rate Calculators: Easy-to-use rate calculators to help you perform calculations and compare values.
Educational Resources: Articles, tutorials, and videos to help you learn about rates, ratios, and other mathematical concepts.

Visit COMPARE.EDU.VN today and discover how we can help you master rates and make smarter decisions.

18. FAQs About Rate Definitions

1. What is the formal definition of rate?

A rate is a ratio that compares two quantities measured in different units.

2. How does a unit rate differ from a regular rate?

A unit rate simplifies the comparison to a single unit of the denominator, making it easy to compare different rates directly.

3. Why are rates important in everyday life?

Rates are used in various situations to compare options, make informed decisions, and analyze data effectively.

4. Can you provide some examples of rate calculations?

Speed (distance/time), price per unit (price/quantity), and heart rate (beats/minute) are common examples of rate calculations.

5. What common mistakes should I avoid when calculating rates?

Mixing up units, incorrectly setting up the ratio, forgetting to simplify, and misinterpreting unit rates are common mistakes to avoid.

6. Where can I find reliable information and tools to help me with rate calculations?

COMPARE.EDU.VN provides detailed comparisons, expert reviews, rate calculators, and educational resources to help you master rates and make smarter decisions.

7. How can understanding rates improve my financial literacy?

Understanding interest rates, investment returns, inflation rates, and exchange rates is crucial for managing your money wisely.

8. What advanced applications of rates are used in science and engineering?

Reaction rates, velocity, acceleration, population growth rates, and economic growth rates are examples of advanced applications of rates.

9. What is the relationship between rates and proportions?

Proportions are equations that state that two ratios (or rates) are equal, used for scaling and conversions.

10. How can COMPARE.EDU.VN help me compare different options using rates?

COMPARE.EDU.VN offers detailed comparisons, expert reviews, and rate calculators to help you make informed decisions based on your individual needs and circumstances.

Make Informed Decisions with COMPARE.EDU.VN

Are you struggling to compare different products, services, or ideas? Do you feel overwhelmed by the amount of information available? COMPARE.EDU.VN is here to help. We provide comprehensive comparisons, detailed analyses, and practical tools to help you make informed decisions with confidence.

Visit COMPARE.EDU.VN today to find the comparisons you need and make the right choice for your needs and budget. Contact us at 333 Comparison Plaza, Choice City, CA 90210, United States. Whatsapp: +1 (626) 555-9090. Website: compare.edu.vn. Let us help you simplify your decision-making process and achieve your goals.