A Ratio That Compares Quantities With Different Units Is A rate. This comprehensive guide, brought to you by COMPARE.EDU.VN, will delve into the concept of rates, unit rates, and their differences from ratios, providing you with a clear understanding and practical examples to enhance your analytical skills. Explore detailed explanations, solved problems, and practice questions to master this essential mathematical concept.
1. Understanding The Definition Of Rate In Mathematics
In mathematics, a ratio that compares quantities with different units is a rate. A rate is essentially a comparison between two quantities with different units. This comparison is expressed as a ratio, illustrating how much of one quantity there is for each unit of another quantity.
For example, consider a scenario where a car travels 120 miles in 2 hours. The rate, in this case, would be 60 miles per hour (120 miles / 2 hours). This means that for every hour, the car covers 60 miles. The word “per” is often a key indicator that you are dealing with a rate, and it can be represented by the symbol “/” in mathematical problems.
Key Points:
- Definition: A rate compares two quantities with different units.
- Example: Miles per hour, words per minute.
- Indicator: The word “per” or the symbol “/” often indicates a rate.
 Car Traveling at a Rate
Car Traveling at a Rate
2. Exploring Unit Rate And Its Significance
A unit rate is a specific type of rate where the second quantity (the denominator) is always one. It simplifies the comparison by expressing how much of the first quantity exists for a single unit of the second quantity.
For instance, if you earn $15 per hour, this is a unit rate. It tells you exactly how much money you earn for one hour of work. Similarly, if a store sells apples at $2 per pound, you know the cost for one pound of apples.
Key Points:
- Definition: A unit rate compares a quantity to one unit of another quantity.
- Denominator: The second quantity in the comparison is always 1.
- Examples: $15 per hour, $2 per pound.
3. Ratio Definition: Comparing Like Quantities
A ratio, unlike a rate, compares two or more quantities with the same units. It shows the relative size of these quantities. Ratios are often written with a colon (:) or as fractions.
For example, if you have 3 apples and 4 oranges, the ratio of apples to oranges is 3:4. This means that for every 3 apples, there are 4 oranges. It’s a comparison of two quantities using the same unit (in this case, the number of fruits).
Key Points:
- Definition: A ratio compares two or more quantities with the same units.
- Notation: Ratios are often written with a colon (:) or as fractions.
- Example: The ratio of apples to oranges is 3:4.
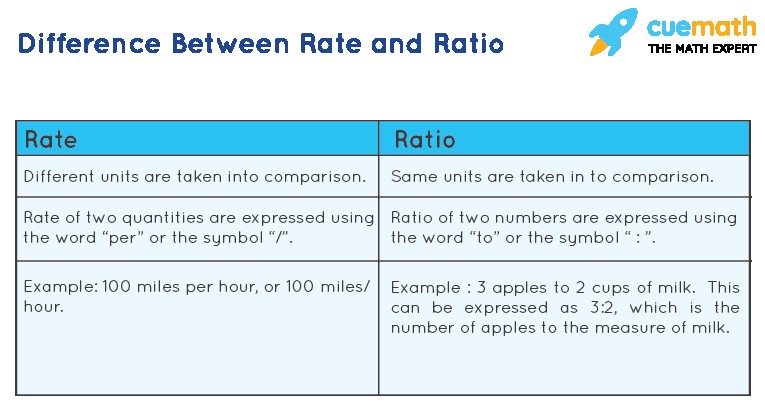
4. Rate And Ratio Difference: Key Distinctions
While both rates and ratios involve comparing quantities, the critical difference lies in the units being compared. A rate compares quantities with different units, while a ratio compares quantities with the same units.
| Feature | Rate | Ratio |
|---|---|---|
| Definition | Compares quantities with different units. | Compares quantities with the same units. |
| Units | Different units (e.g., miles/hour). | Same units (e.g., apples to oranges). |
| Use Case | Measuring speed, cost per item, etc. | Comparing quantities within the same category. |
| Representation | Often expressed with “per” or “/”. | Often expressed with a colon (:) or as a fraction. |
Examples:
- Rate: The speed of a car is 60 miles per hour.
- Ratio: The ratio of students to teachers is 20:1.
5. Calculating Rate: Step-By-Step Guide
Calculating a rate involves dividing one quantity by another to find the ratio between them. Here’s a step-by-step guide:
- Identify the Quantities: Determine the two quantities you want to compare. Ensure they have different units.
- Write the Ratio: Write the first quantity as the numerator and the second quantity as the denominator.
- Simplify: Simplify the ratio to its simplest form.
- Include Units: Always include the units in your answer to provide context.
Example:
Suppose you travel 300 miles in 5 hours. To find the rate (speed), follow these steps:
- Quantities: Distance = 300 miles, Time = 5 hours
- Ratio: Rate = 300 miles / 5 hours
- Simplify: Rate = 60 miles / 1 hour
- Answer: The rate is 60 miles per hour.
6. Real-World Examples Of Rate
Rates are used extensively in everyday life and various fields. Here are some common examples:
- Speed: Miles per hour (mph) or kilometers per hour (km/h)
- Price: Dollars per pound ($/lb) or euros per kilogram (€/kg)
- Consumption: Liters per kilometer (L/km) or gallons per mile (mpg)
- Work: Words per minute (wpm) or tasks per day
These rates help us make informed decisions, whether it’s choosing the most fuel-efficient car or comparing the cost of groceries.
7. Applications Of Unit Rate
Unit rates are particularly useful for comparing different options. By converting rates to unit rates, you can easily see which option offers the best value.
Example:
Suppose you are comparing two brands of coffee:
- Brand A: $8 for 2 pounds
- Brand B: $12 for 3 pounds
To find the unit rate for each:
- Brand A: $8 / 2 pounds = $4 per pound
- Brand B: $12 / 3 pounds = $4 per pound
In this case, both brands have the same unit rate, so the cost per pound is the same.
8. Tips For Solving Rate Problems
Solving rate problems can be straightforward with the right approach. Here are some helpful tips:
- Read Carefully: Understand the problem and identify the quantities and their units.
- Set Up the Ratio Correctly: Ensure the quantities are in the correct order (numerator/denominator).
- Simplify: Simplify the ratio to its simplest form.
- Include Units: Always include units in your final answer.
- Check Your Answer: Make sure your answer makes sense in the context of the problem.
9. Common Mistakes To Avoid When Working With Rates
- Mixing Units: Ensure the units are consistent throughout the problem. If not, convert them to the same unit.
- Incorrect Setup: Placing the quantities in the wrong order in the ratio.
- Forgetting Units: Omitting units in the final answer, which can lead to misinterpretation.
- Not Simplifying: Failing to simplify the ratio to its simplest form, making it harder to interpret.
10. Solved Examples On Rate Definition
Example 1: Calculating Typing Speed
John types 400 words in 10 minutes. What is his typing speed in words per minute?
Solution:
- Quantities: Words = 400, Time = 10 minutes
- Ratio: Rate = 400 words / 10 minutes
- Simplify: Rate = 40 words / 1 minute
- Answer: John’s typing speed is 40 words per minute.
Example 2: Finding the Cost Per Item
A store sells 5 apples for $3. What is the cost per apple?
Solution:
- Quantities: Cost = $3, Apples = 5
- Ratio: Rate = $3 / 5 apples
- Simplify: Rate = $0.60 / 1 apple
- Answer: The cost per apple is $0.60.
Example 3: Determining Fuel Efficiency
A car travels 350 miles on 14 gallons of gas. What is the car’s fuel efficiency in miles per gallon?
Solution:
- Quantities: Distance = 350 miles, Gas = 14 gallons
- Ratio: Rate = 350 miles / 14 gallons
- Simplify: Rate = 25 miles / 1 gallon
- Answer: The car’s fuel efficiency is 25 miles per gallon.
11. Practice Questions On Rate
- A train travels 600 miles in 8 hours. What is its average speed in miles per hour?
- A worker produces 150 parts in 6 hours. What is the production rate in parts per hour?
- A store sells 3 oranges for $2. What is the cost per orange?
- A student reads 120 pages in 4 hours. What is the reading rate in pages per hour?
- A factory uses 5000 liters of water in 2 days. What is the water consumption rate in liters per day?
12. Advanced Rate Problems
Problem 1: Comparing Speeds
Car A travels 300 miles in 5 hours, and Car B travels 250 miles in 4 hours. Which car is faster?
Solution:
- Car A: Rate = 300 miles / 5 hours = 60 mph
- Car B: Rate = 250 miles / 4 hours = 62.5 mph
- Answer: Car B is faster.
Problem 2: Calculating Unit Cost with Discounts
A store sells bananas for $0.50 each. If you buy a dozen, you get a 10% discount. What is the unit cost of a banana when buying a dozen?
Solution:
- Cost of a dozen: 12 bananas * $0.50 = $6
- Discount: $6 * 10% = $0.60
- Discounted cost: $6 – $0.60 = $5.40
- Unit cost: $5.40 / 12 bananas = $0.45 per banana
- Answer: The unit cost is $0.45 per banana when buying a dozen.
Problem 3: Combining Rates
John can paint a room in 6 hours, and Mary can paint the same room in 8 hours. How long will it take them to paint the room together?
Solution:
- John’s rate: 1/6 room per hour
- Mary’s rate: 1/8 room per hour
- Combined rate: 1/6 + 1/8 = 4/24 + 3/24 = 7/24 room per hour
- Time to paint together: 1 / (7/24) = 24/7 hours ≈ 3.43 hours
- Answer: It will take them approximately 3.43 hours to paint the room together.
13. Exploring More Complex Rate Scenarios
Scenario 1: Currency Exchange Rates
Understanding currency exchange rates is crucial for international travelers and businesses. An exchange rate is a rate that specifies how much one currency is worth in terms of another currency.
Example:
If the exchange rate is 1 USD = 0.85 EUR, it means that 1 US dollar is equivalent to 0.85 euros.
Application:
Suppose you want to convert 500 USD to EUR.
- Calculation: 500 USD * 0.85 EUR/USD = 425 EUR
- Answer: 500 USD is equivalent to 425 EUR.
Scenario 2: Interest Rates
Interest rates are a common application of rates in finance. They represent the cost of borrowing money or the return on an investment, usually expressed as a percentage per year.
Example:
If you deposit $1000 in a savings account with an annual interest rate of 5%, you will earn $50 in interest after one year.
Calculation:
- Interest earned: $1000 * 5% = $50
- Answer: You will earn $50 in interest.
Scenario 3: Population Density
Population density is a rate that measures the number of people per unit area, such as people per square mile or square kilometer. It helps in understanding how crowded a region is.
Example:
If a city has a population of 500,000 people and an area of 100 square miles, the population density is:
Calculation:
- Population density: 500,000 people / 100 square miles = 5000 people per square mile
- Answer: The population density is 5000 people per square mile.
14. Understanding Rate Of Change
The rate of change is a concept used to describe how one quantity changes in relation to another quantity. It is a fundamental concept in calculus and is used in various fields such as physics, economics, and engineering.
Average Rate Of Change
The average rate of change is the change in the value of a quantity divided by the elapsed time.
Formula:
Average Rate of Change = (Change in Quantity) / (Change in Time)
Example:
If the temperature of a room increases from 20°C to 25°C in 2 hours, the average rate of change is:
- Change in temperature: 25°C – 20°C = 5°C
- Change in time: 2 hours
- Average Rate of Change: 5°C / 2 hours = 2.5°C per hour
Instantaneous Rate Of Change
The instantaneous rate of change is the rate of change at a specific point in time. It is found by taking the derivative of the function that describes the quantity with respect to time.
Example:
Suppose the position of a car is given by the function s(t) = 3t^2 + 2t, where s is in meters and t is in seconds. The instantaneous velocity (rate of change of position) at t = 3 seconds is:
- Derivative: v(t) = ds/dt = 6t + 2
- Velocity at t = 3: v(3) = 6(3) + 2 = 20 m/s
15. Rate Definition: The Role Of Proportions
Proportions are mathematical statements that show two ratios (or rates) are equal. Understanding proportions can simplify solving rate-related problems.
Direct Proportion
In a direct proportion, as one quantity increases, the other quantity increases proportionally.
Example:
If 2 apples cost $1, then 4 apples will cost $2. This is a direct proportion because the cost increases linearly with the number of apples.
Solving Problems:
If 5 workers can complete a task in 10 days, how long will it take 10 workers to complete the same task, assuming they work at the same rate?
- Set up proportion: (5 workers) / (10 days) = (10 workers) / (x days)
- Solve for x: x = (10 workers * 10 days) / 5 workers = 20 days
- Answer: It will take 10 workers 5 days to complete the same task.
Inverse Proportion
In an inverse proportion, as one quantity increases, the other quantity decreases.
Example:
If 2 workers can complete a task in 6 days, then 4 workers can complete the same task in 3 days. This is an inverse proportion because the time decreases as the number of workers increases.
Solving Problems:
If a car travels at 50 mph and takes 4 hours to reach a destination, how long will it take if the car travels at 100 mph?
- Set up proportion: (50 mph) / (4 hours) = (100 mph) / (x hours)
- Solve for x: x = (50 mph * 4 hours) / 100 mph = 2 hours
- Answer: It will take 2 hours if the car travels at 100 mph.
16. Practical Exercises To Strengthen Your Understanding Of Rate
Exercise 1: Calculating Fuel Consumption
A car travels 450 miles on 15 gallons of gasoline. Calculate the car’s fuel consumption rate in miles per gallon (mpg).
Solution:
- Rate Calculation: mpg = Total Miles / Total Gallons
- Plugging in Values: mpg = 450 miles / 15 gallons
- Simplifying: mpg = 30 miles/gallon
- Answer: The car’s fuel consumption rate is 30 miles per gallon.
Exercise 2: Determining Hourly Wage
An individual earns $560 for working 40 hours in a week. Find the hourly wage.
Solution:
- Rate Calculation: Hourly Wage = Total Earnings / Total Hours Worked
- Plugging in Values: Hourly Wage = $560 / 40 hours
- Simplifying: Hourly Wage = $14/hour
- Answer: The hourly wage is $14 per hour.
Exercise 3: Calculating Production Rate
A factory produces 2400 units of a product in 8 hours. Calculate the production rate per hour.
Solution:
- Rate Calculation: Production Rate = Total Units Produced / Total Hours
- Plugging in Values: Production Rate = 2400 units / 8 hours
- Simplifying: Production Rate = 300 units/hour
- Answer: The production rate is 300 units per hour.
Exercise 4: Comparing Reading Speeds
Person A reads 90 pages in 3 hours, while Person B reads 120 pages in 4 hours. Compare their reading speeds in pages per hour.
Solution:
- Person A’s Reading Speed:
- Reading Speed = Total Pages / Total Hours
- Reading Speed = 90 pages / 3 hours = 30 pages/hour
- Person B’s Reading Speed:
- Reading Speed = Total Pages / Total Hours
- Reading Speed = 120 pages / 4 hours = 30 pages/hour
- Comparison: Both individuals have the same reading speed of 30 pages per hour.
Exercise 5: Calculating Water Flow Rate
A water tank fills up with 3600 liters in 6 hours. Calculate the water flow rate in liters per hour.
Solution:
- Rate Calculation: Water Flow Rate = Total Liters / Total Hours
- Plugging in Values: Water Flow Rate = 3600 liters / 6 hours
- Simplifying: Water Flow Rate = 600 liters/hour
- Answer: The water flow rate is 600 liters per hour.
17. Rate Definition: Linking With Statistical Analysis
In statistical analysis, rates are often used to represent the occurrence of events relative to a population or time period. They are essential for comparing data across different groups or timeframes.
Mortality Rate
Mortality rate is a statistical measure of the number of deaths in a given population, scaled to the size of that population, per unit of time. It is typically expressed in deaths per 1,000 people per year.
Formula:
Mortality Rate = (Number of Deaths / Total Population) * 1000
Example:
If a town has a population of 50,000 and records 600 deaths in a year:
- Mortality Rate Calculation:
- Mortality Rate = (600 deaths / 50,000 population) * 1000
- Mortality Rate = 0.012 * 1000 = 12 deaths per 1,000 people
- Answer: The mortality rate is 12 deaths per 1,000 people per year.
Incidence Rate
Incidence rate is a measure of the frequency with which new cases of a disease or condition occur in a population over a period of time. It is typically expressed as the number of new cases per 1,000 or 100,000 people per year.
Formula:
Incidence Rate = (Number of New Cases / Total Population at Risk) * 1000 (or 100,000)
Example:
If a city has a population of 200,000 and records 400 new cases of a disease in a year:
- Incidence Rate Calculation:
- Incidence Rate = (400 new cases / 200,000 population) * 100,000
- Incidence Rate = 0.002 * 100,000 = 200 new cases per 100,000 people
- Answer: The incidence rate is 200 new cases per 100,000 people per year.
Prevalence Rate
Prevalence rate is a measure of the total number of individuals in a population who have a disease or condition at a specific time. It is expressed as the number of cases per 1,000 or 100,000 people.
Formula:
Prevalence Rate = (Total Number of Cases / Total Population) * 1000 (or 100,000)
Example:
If a country has a population of 5,000,000 and there are 25,000 people with a certain condition:
- Prevalence Rate Calculation:
- Prevalence Rate = (25,000 cases / 5,000,000 population) * 100,000
- Prevalence Rate = 0.005 * 100,000 = 500 cases per 100,000 people
- Answer: The prevalence rate is 500 cases per 100,000 people.
18. Exploring The Ethical Implications Of Using Rates In Analysis
When utilizing rates for analytical purposes, ethical considerations become paramount. These considerations ensure fairness, prevent misinterpretation, and maintain transparency in data-driven decision-making.
Avoiding Misinterpretation
Rates can sometimes be misleading if not presented with proper context. For example, a higher mortality rate in one hospital compared to another does not necessarily indicate poorer quality of care. Factors such as the types of patients treated, the severity of their conditions, and the demographics of the patient population can significantly influence mortality rates.
Ethical Consideration:
- Contextual Presentation: Always provide comprehensive context when presenting rates. Include relevant factors that might influence the results to prevent misinterpretations.
Ensuring Fair Comparison
When comparing rates across different groups or time periods, it’s crucial to ensure that the comparisons are fair and equitable. Differences in demographic characteristics, socioeconomic factors, or environmental conditions can affect rates and skew comparisons.
Ethical Consideration:
- Standardization: Use standardization techniques to adjust rates for confounding variables, such as age, sex, or socioeconomic status. This helps to ensure that comparisons are based on similar populations.
Protecting Privacy
Rates often involve aggregating data about individuals, which can raise privacy concerns. It’s essential to protect the confidentiality of individuals and avoid disclosing sensitive information that could be used to identify them.
Ethical Consideration:
- Anonymization: Anonymize data whenever possible to protect the privacy of individuals. Remove or mask any identifiers that could be used to link data back to specific individuals.
Transparency And Accountability
Transparency and accountability are essential principles in ethical analysis. Be transparent about the methods used to calculate rates, the sources of data, and any limitations of the analysis. Be accountable for the accuracy and reliability of the results.
Ethical Consideration:
- Documentation: Document all aspects of the analysis, including data sources, methods, and assumptions. Make this documentation available to stakeholders to ensure transparency and allow for scrutiny.
Avoiding Bias
Bias can occur at various stages of the analysis, from data collection to interpretation. It’s important to be aware of potential sources of bias and take steps to mitigate their impact.
Ethical Consideration:
- Objective Analysis: Strive for objectivity in the analysis. Avoid selectively presenting data or using methods that are likely to produce results that support a particular viewpoint.
19. Case Studies Illustrating The Use Of Rates In Decision-Making
Case Study 1: Healthcare Management
A hospital administrator uses mortality rates to assess the quality of care provided in different departments. After analyzing the data, they discover that the cardiology department has a higher mortality rate compared to other departments.
Decision-Making Process:
- Data Analysis: The administrator examines the mortality rates and identifies potential factors contributing to the higher rate, such as the severity of patients’ conditions and the availability of specialized resources.
- Investigation: The administrator conducts a thorough investigation to determine the root causes of the higher mortality rate.
- Intervention: Based on the findings, the administrator implements interventions to improve the quality of care, such as providing additional training for staff and investing in new equipment.
- Monitoring: The administrator continues to monitor the mortality rates to assess the effectiveness of the interventions and make further adjustments as needed.
Case Study 2: Marketing Strategy
A marketing manager uses conversion rates to evaluate the effectiveness of different advertising campaigns. After analyzing the data, they discover that one campaign has a significantly higher conversion rate compared to others.
Decision-Making Process:
- Data Analysis: The manager examines the conversion rates and identifies potential factors contributing to the higher rate, such as the target audience, the message, and the channel used.
- Optimization: The manager optimizes the other campaigns based on the successful campaign’s elements, such as refining the target audience and improving the message.
- Testing: The manager conducts A/B testing to compare different versions of the campaigns and identify the most effective strategies.
- Allocation: The manager allocates more resources to the campaigns with the highest conversion rates to maximize the return on investment.
Case Study 3: Public Health Policy
A public health official uses incidence rates to track the spread of infectious diseases in a community. After analyzing the data, they discover a sudden increase in the incidence rate of a particular disease.
Decision-Making Process:
- Data Analysis: The official examines the incidence rates and identifies potential factors contributing to the increase, such as a lack of vaccination and poor sanitation.
- Intervention: The official implements interventions to control the spread of the disease, such as launching a vaccination campaign and improving sanitation practices.
- Monitoring: The official continues to monitor the incidence rates to assess the effectiveness of the interventions and make further adjustments as needed.
- Communication: The official communicates the findings to the public and provides recommendations for preventing the spread of the disease.
20. Frequently Asked Questions (FAQs) On Rate Definition
What Is The Definition Of Rate?
A rate is a ratio that compares two quantities with different units, expressing how much of one quantity there is for each unit of another quantity.
What Is Unit Rate Definition?
A unit rate is a rate where the second quantity is one, simplifying the comparison to a single unit.
What Is Simple Interest Rate Definition?
In simple interest, the rate is the percentage of the principal amount charged as interest per year.
What Is The Definition Of Rate In Math?
In mathematics, a rate is defined as a ratio between two quantities with different units, often expressed as a fraction.
What Is The Difference Between A Rate And A Percentage?
A rate compares two numbers with different quantities, while a percentage is a ratio out of a hundred.
What Are Three Examples Of Rate?
Examples include miles per hour, cost per pound, and heartbeats per minute.
What Is The Difference Between Rate And Unit Rate?
A rate is a ratio of two different quantities, whereas a unit rate expresses the amount of the first quantity for one unit of the second quantity.
How Do You Calculate Rate?
Divide the first quantity by the second quantity, ensuring the units are different.
Why Is Understanding Rate Important?
Understanding rates helps in making informed decisions in various aspects of life, from finance to healthcare.
Where Can I Find More Information On Rates?
For more detailed explanations and comparisons, visit COMPARE.EDU.VN for comprehensive resources and guides.
Navigating the world of comparisons can be challenging, but with COMPARE.EDU.VN, you gain access to detailed, objective analyses that empower you to make informed decisions. Whether you’re evaluating products, services, or ideas, we provide the insights you need.
Ready to make smarter choices? Visit COMPARE.EDU.VN today and start comparing with confidence. Our expert comparisons are designed to help you find the best options tailored to your needs and budget.
Take the guesswork out of decision-making. Explore COMPARE.EDU.VN now and discover a world of clear, comprehensive comparisons at your fingertips.
Contact Information:
Address: 333 Comparison Plaza, Choice City, CA 90210, United States
Whatsapp: +1 (626) 555-9090
Website: compare.edu.vn