A Fraction That Compares Two Equivalent Measurements is called a conversion factor, and it’s a ratio demonstrating the equivalence between two different units of measurement; let’s explore how you can utilize COMPARE.EDU.VN to master these critical concepts and make informed decisions. This concept is fundamental in various applications, from everyday tasks to complex scientific calculations, essential for accurate unit conversions and problem-solving, crucial for accurate calculations, and aids in maintaining dimensional consistency.
1. Understanding Conversion Factors
A conversion factor is a fraction that compares two equivalent measurements expressed in different units. Since the numerator and denominator represent the same quantity, the fraction is equal to one. Multiplying by a conversion factor changes the units of a measurement without changing its value. This equivalence is vital for transforming measurements across different units while maintaining accuracy.
1.1. Definition of a Conversion Factor
A conversion factor is a ratio that equates two different units. This fraction is always equal to 1, because the value on top is equal to the value on the bottom. This principle is applicable in scenarios where you need to shift from measuring in feet to meters, or from pounds to kilograms. The conversion factor acts as a bridge, allowing for seamless transitions between units of measurement.
For example, to convert inches to feet, the conversion factor is 1 foot / 12 inches. Since 1 foot is equal to 12 inches, this fraction is equal to 1. Multiplying any measurement in inches by this factor will convert it to feet without changing the actual length. This principle underscores the essence of conversion factors: maintaining the integrity of the measurement while altering its expression.
1.2. Importance in Measurement
Conversion factors are important because they allow you to express the same measurement in different units. It enables you to switch from grams to ounces or from miles per hour to kilometers per hour. The ability to work fluently with different measurement systems is crucial for anyone involved in science, engineering, or international trade, making conversion factors an indispensable tool.
Using conversion factors correctly ensures that calculations are accurate and consistent, especially when dealing with complex problems involving multiple units. This accuracy is essential in fields where precision is key, such as in pharmaceutical formulations or structural engineering.
1.3. Basic Examples of Conversion Factors
Here are some common conversion factors:
- Length: 1 meter = 100 centimeters
- Mass: 1 kilogram = 1000 grams
- Time: 1 minute = 60 seconds
These basic conversion factors are used daily in a variety of contexts, from cooking to construction, highlighting their universal utility. Understanding and applying these factors can simplify everyday tasks and improve accuracy in various fields.
2. How Conversion Factors Work
The magic of conversion factors lies in their ability to change units while preserving value. This is achieved by setting up the conversion factor so that the unwanted unit cancels out, leaving the desired unit in its place. Let’s delve deeper into the mechanics of this process.
2.1. Setting Up the Conversion Factor
When using conversion factors, ensure the units you want to convert from are in the denominator and the units you want to convert to are in the numerator. This setup ensures that the original units cancel out, leaving you with the desired units. This positioning is not arbitrary; it’s a strategic arrangement that leverages the properties of fractions to achieve the desired unit transformation.
For instance, if you want to convert 5 meters to centimeters, the conversion factor should be set up as (100 centimeters / 1 meter). When you multiply 5 meters by this conversion factor, the “meters” unit cancels out, and you are left with the measurement in centimeters:
5 meters * (100 centimeters / 1 meter) = 500 centimeters
2.2. Canceling Units
The key to using conversion factors is to cancel out the units you no longer need. By placing the original unit in the denominator of the conversion factor, you create a situation where the units divide out, similar to simplifying a fraction. This cancellation is a visual confirmation that the conversion has been set up correctly and that the resulting units will be what you intended.
Consider converting 72 inches to feet. You know that 1 foot = 12 inches. The conversion factor is set up as (1 foot / 12 inches). Multiply 72 inches by this factor:
72 inches * (1 foot / 12 inches) = 6 feet
The “inches” unit cancels out, and you are left with 6 feet, demonstrating the effectiveness of this method.
2.3. Multiplying and Dividing
Once you’ve set up the conversion factor and canceled the units, perform the multiplication or division to get your answer in the desired units. Double-check your work to ensure you have correctly applied the conversion factor and performed the arithmetic. This step ensures that the numerical value of the measurement is accurately transformed along with the units.
Let’s convert 15 kilograms to grams. The conversion factor is 1 kilogram = 1000 grams. Set up the conversion as:
15 kilograms * (1000 grams / 1 kilogram) = 15,000 grams
By multiplying 15 by 1000, you find that 15 kilograms is equal to 15,000 grams, demonstrating the final step in the conversion process.
3. Common Conversion Factors in Everyday Life
Conversion factors are not just theoretical tools; they are practical aids used in various everyday situations. Whether you’re cooking, traveling, or working on a home improvement project, understanding and using conversion factors can make tasks easier and more accurate.
3.1. Cooking and Baking
In the kitchen, conversion factors are frequently used to adjust recipes or convert between different measurement systems. For example, converting ounces to grams or cups to milliliters can be essential when following recipes from different regions. These conversions ensure that the proportions of ingredients are accurate, leading to successful culinary outcomes.
Here are some common cooking conversion factors:
- 1 cup = 8 fluid ounces
- 1 tablespoon = 3 teaspoons
- 1 pound = 16 ounces
Using these conversions allows you to adapt recipes to your available measuring tools and ensure consistent results, regardless of the original recipe’s measurement system.
3.2. Travel and Distance
When traveling, you may need to convert between miles and kilometers or Fahrenheit and Celsius. These conversions are crucial for understanding distances, weather conditions, and other important information. They allow you to navigate unfamiliar environments with confidence and make informed decisions based on local conditions.
Key travel-related conversion factors include:
- 1 mile = 1.609 kilometers
- Celsius to Fahrenheit: °F = (°C * 9/5) + 32
- Fahrenheit to Celsius: °C = (°F – 32) * 5/9
With these conversions, you can easily translate distances on road signs or understand weather forecasts, ensuring a smoother and more informed travel experience.
3.3. Home Improvement and Construction
Home improvement and construction projects often require converting between different units of length, area, and volume. Whether you’re measuring lumber, calculating paint quantities, or estimating flooring needs, conversion factors are essential for accurate planning and execution. They prevent costly errors and ensure that materials are used efficiently.
Useful conversion factors for home improvement include:
- 1 inch = 2.54 centimeters
- 1 square foot = 0.0929 square meters
- 1 gallon = 3.785 liters
By using these conversions, you can accurately estimate material requirements and avoid common pitfalls in home improvement projects, leading to better results and cost savings.
4. Conversion Factors in Science and Engineering
In science and engineering, conversion factors are indispensable for ensuring precision and consistency in calculations. These fields often deal with a wide array of units, and accurate conversions are essential for reliable results. Let’s explore some key areas where conversion factors play a critical role.
4.1. Physics and Chemistry
Physics and chemistry rely heavily on accurate unit conversions. From calculating energy in joules to measuring concentrations in moles per liter, conversion factors ensure that all values are expressed in consistent units. This consistency is vital for performing accurate calculations and validating experimental results.
Common conversion factors in physics and chemistry include:
- 1 joule = 1 kg*m²/s²
- 1 mole = 6.022 x 10²³ particles
- 1 atmosphere = 101,325 pascals
These conversions allow scientists to work with different units while maintaining the integrity of their calculations, leading to more reliable and reproducible results.
4.2. Engineering Disciplines
Different engineering disciplines, such as civil, mechanical, and electrical engineering, use conversion factors to ensure accuracy in design and construction. Whether it’s converting between pounds per square inch (PSI) and pascals for pressure calculations or converting horsepower to watts for power calculations, these conversions are crucial for safety and efficiency.
Examples of conversion factors used in engineering:
- 1 horsepower = 745.7 watts
- 1 PSI = 6894.76 pascals
- 1 cubic meter = 1000 liters
These conversions allow engineers to design and build structures and systems that meet precise specifications and perform reliably under various conditions.
4.3. Unit Analysis
Unit analysis, also known as dimensional analysis, is a technique that uses conversion factors to check the correctness of equations and calculations. By ensuring that the units on both sides of an equation match, you can verify the accuracy of your work. This method is particularly useful in complex problems involving multiple steps and different units.
For example, if you are calculating force (F = ma), the units should be consistent:
- F (force) in newtons (N)
- m (mass) in kilograms (kg)
- a (acceleration) in meters per second squared (m/s²)
Ensuring that the units align helps prevent errors and confirms that the equation is dimensionally consistent, leading to more accurate results.
5. Tips for Using Conversion Factors Effectively
Using conversion factors effectively requires attention to detail and a systematic approach. By following these tips, you can minimize errors and ensure accurate conversions every time.
5.1. Know Your Conversion Factors
The first step in using conversion factors effectively is to know the common conversions you’ll need. Keep a list of frequently used conversion factors handy, and take the time to memorize those you use most often. This knowledge base will speed up your calculations and reduce the likelihood of errors.
Commonly used conversion factors include:
- Length: inches to centimeters, feet to meters, miles to kilometers
- Mass: ounces to grams, pounds to kilograms
- Volume: cups to milliliters, gallons to liters
5.2. Write Out the Units
Always write out the units when performing conversions. This practice helps you keep track of the units and ensures that you cancel them correctly. It also makes it easier to spot errors in your setup and prevents confusion.
For example, when converting 10 miles to kilometers:
10 miles * (1.609 kilometers / 1 mile)
Writing out the units makes it clear that the “miles” unit will cancel, leaving you with kilometers.
5.3. Double-Check Your Work
After completing a conversion, double-check your work to ensure that you have set up the conversion factor correctly and performed the arithmetic accurately. Verify that the units have canceled out as expected and that the answer makes sense in the context of the problem.
For example, if you are converting a small distance from miles to kilometers, the answer in kilometers should be larger than the original value in miles, since a kilometer is shorter than a mile.
6. Advanced Conversion Techniques
Beyond simple, single-step conversions, there are advanced techniques for handling more complex conversions. These techniques are particularly useful in scientific and engineering applications where multiple units need to be converted simultaneously.
6.1. Multi-Step Conversions
Some conversions require multiple steps. When faced with such conversions, break them down into smaller, more manageable steps. Use a series of conversion factors to gradually transform the units until you reach the desired result.
For example, to convert miles per hour (mph) to meters per second (m/s), you’ll need to convert miles to meters and hours to seconds:
- Convert miles to kilometers: 1 mile = 1.609 kilometers
- Convert kilometers to meters: 1 kilometer = 1000 meters
- Convert hours to minutes: 1 hour = 60 minutes
- Convert minutes to seconds: 1 minute = 60 seconds
By combining these conversions, you can convert mph to m/s accurately.
6.2. Using Dimensional Analysis
Dimensional analysis is a powerful technique for complex conversions. It involves tracking the units through each step of a calculation to ensure that the final answer has the correct units. This method is particularly useful when dealing with equations that involve multiple variables and different units.
For instance, if you’re calculating the volume flow rate (Q) of a fluid through a pipe using the equation Q = Av, where A is the cross-sectional area and v is the velocity, you must ensure that the units are consistent:
- A (area) in square meters (m²)
- v (velocity) in meters per second (m/s)
- Q (volume flow rate) in cubic meters per second (m³/s)
Ensuring that the units align at each step helps prevent errors and confirms the accuracy of the calculation.
6.3. Handling Compound Units
Compound units, such as density (mass per volume) or speed (distance per time), require special attention when converting. Convert each component of the compound unit separately, ensuring that all units are correctly transformed.
For example, to convert density from grams per cubic centimeter (g/cm³) to kilograms per cubic meter (kg/m³):
- Convert grams to kilograms: 1 gram = 0.001 kilograms
- Convert cubic centimeters to cubic meters: 1 cm³ = 1 x 10⁻⁶ m³
Combining these conversions gives you the correct density in kg/m³.
7. Practical Examples and Exercises
To solidify your understanding of conversion factors, let’s work through some practical examples and exercises. These examples cover a range of scenarios from everyday life to scientific applications.
7.1. Example 1: Converting Speed
Convert 60 miles per hour (mph) to kilometers per hour (km/h).
Solution:
- Identify the conversion factor: 1 mile = 1.609 kilometers
- Set up the conversion:
60 miles/hour * (1.609 kilometers / 1 mile) - Cancel the units: The “miles” unit cancels out.
- Perform the calculation:
60 * 1.609 = 96.54 kilometers/hour
Therefore, 60 mph is equal to 96.54 km/h.
7.2. Example 2: Converting Volume
Convert 5 gallons to liters.
Solution:
- Identify the conversion factor: 1 gallon = 3.785 liters
- Set up the conversion:
5 gallons * (3.785 liters / 1 gallon) - Cancel the units: The “gallons” unit cancels out.
- Perform the calculation:
5 * 3.785 = 18.925 liters
Therefore, 5 gallons is equal to 18.925 liters.
7.3. Exercise 1: Converting Mass
Convert 250 grams to ounces. (1 ounce = 28.35 grams)
7.4. Exercise 2: Converting Length
Convert 15 feet to meters. (1 foot = 0.3048 meters)
7.5. Solutions to Exercises
Exercise 1 Solution:
- Set up the conversion:
250 grams * (1 ounce / 28.35 grams) - Cancel the units: The “grams” unit cancels out.
- Perform the calculation:
250 / 28.35 = 8.82 ounces (approximately)
Exercise 2 Solution:
- Set up the conversion:
15 feet * (0.3048 meters / 1 foot) - Cancel the units: The “feet” unit cancels out.
- Perform the calculation:
15 * 0.3048 = 4.572 meters
8. Common Mistakes to Avoid
Even with a solid understanding of conversion factors, it’s easy to make mistakes if you’re not careful. Here are some common pitfalls to avoid when performing conversions.
8.1. Using the Wrong Conversion Factor
One of the most common mistakes is using the wrong conversion factor. Always double-check that you are using the correct conversion factor for the units you are converting between.
Example: Using 1 inch = 2.45 cm instead of the correct value 1 inch = 2.54 cm.
8.2. Setting Up the Conversion Factor Incorrectly
Setting up the conversion factor incorrectly can lead to the units not canceling out properly. Make sure that the units you want to convert from are in the denominator and the units you want to convert to are in the numerator.
Example: Converting inches to feet but using (12 inches / 1 foot) instead of (1 foot / 12 inches).
8.3. Forgetting to Cancel Units
Forgetting to cancel the units can lead to an incorrect answer with the wrong units. Always ensure that the units cancel out properly to leave you with the desired units.
Example: Not canceling “miles” when converting miles per hour to kilometers per hour, resulting in an answer in “miles*kilometers/hour.”
8.4. Not Double-Checking the Answer
Failing to double-check the answer can result in errors going unnoticed. Always verify that the answer makes sense in the context of the problem and that the units are correct.
Example: Converting a large number of feet to inches and getting a smaller number because of a calculation error.
9. Conversion Factors and Technology
In today’s digital age, technology offers numerous tools to simplify unit conversions. From online calculators to mobile apps, these resources can make conversions quicker and more accurate.
9.1. Online Conversion Calculators
Numerous online conversion calculators are available that can quickly convert between different units. These calculators are easy to use and can handle a wide range of conversions.
Examples:
- Google Unit Converter
- ConvertUnits.com
- UnitConverters.net
9.2. Mobile Apps for Conversions
Mobile apps offer convenient access to conversion tools on the go. These apps often include additional features, such as the ability to save frequently used conversions and customize units.
Examples:
- Unit Converter (available on iOS and Android)
- ConvertPad (available on iOS and Android)
- CalcKit (available on Android)
9.3. Spreadsheets and Software
Spreadsheets and software programs like Microsoft Excel and Google Sheets can be used to perform unit conversions. These tools allow you to create custom conversion formulas and automate complex calculations.
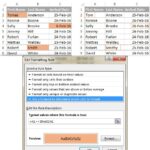
Example: In Excel, you can use the CONVERT function to convert between different units:
=CONVERT(value, "from_unit", "to_unit")For instance, =CONVERT(10,"mi","km") converts 10 miles to kilometers.
10. The Importance of Precision and Accuracy
Precision and accuracy are paramount when using conversion factors, especially in critical applications. A small error in conversion can lead to significant consequences, whether in scientific research, engineering design, or everyday tasks.
10.1. Impact on Scientific Research
In scientific research, accurate unit conversions are essential for reliable results. Incorrect conversions can lead to flawed data, invalid conclusions, and wasted resources. Reproducibility, a cornerstone of the scientific method, relies on the accurate conversion and reporting of measurements.
Example: In pharmaceutical research, a slight error in converting drug dosages from milligrams to grams can have serious implications for patient safety and the validity of clinical trials.
10.2. Consequences in Engineering
In engineering, precision in unit conversions is critical for safety and performance. Incorrect conversions can lead to structural failures, equipment malfunctions, and other hazardous situations.
Example: In civil engineering, a mistake in converting measurements from feet to meters when designing a bridge can compromise its structural integrity, leading to potential collapse.
10.3. Real-World Examples of Errors
Numerous real-world examples illustrate the potential consequences of errors in unit conversions. These incidents highlight the importance of diligence and accuracy when working with conversion factors.
Examples:
- Mars Climate Orbiter (1999): The spacecraft was lost due to a mismatch in units between the software used by NASA and Lockheed Martin. NASA used metric units, while Lockheed Martin used English units, leading to incorrect trajectory calculations.
- Gimli Glider (1983): An Air Canada Boeing 767 ran out of fuel mid-flight due to a miscalculation of fuel quantity. The ground crew used the wrong conversion factor, resulting in the aircraft carrying significantly less fuel than required.
FAQ About Conversion Factors
1. What is a conversion factor?
A conversion factor is a fraction that compares two equivalent measurements expressed in different units. This allows you to convert a measurement from one unit to another without changing its value.
2. Why are conversion factors important?
Conversion factors are important because they allow you to express measurements in different units, which is essential in various fields such as science, engineering, and everyday life. They ensure accurate and consistent calculations.
3. How do I set up a conversion factor?
To set up a conversion factor, place the unit you want to convert from in the denominator and the unit you want to convert to in the numerator. This ensures that the original unit cancels out, leaving you with the desired unit.
4. Can you give an example of a simple conversion factor?
A simple conversion factor is 1 foot = 12 inches. To convert inches to feet, you would use the conversion factor (1 foot / 12 inches).
5. What is unit analysis?
Unit analysis, also known as dimensional analysis, is a technique that uses conversion factors to check the correctness of equations and calculations by ensuring that the units on both sides of an equation match.
6. What are some common mistakes to avoid when using conversion factors?
Common mistakes include using the wrong conversion factor, setting up the conversion factor incorrectly, forgetting to cancel units, and not double-checking the answer.
7. Are there tools available to help with unit conversions?
Yes, numerous tools are available, including online conversion calculators, mobile apps, and spreadsheet software like Microsoft Excel and Google Sheets.
8. How do I handle multi-step conversions?
For multi-step conversions, break them down into smaller, more manageable steps. Use a series of conversion factors to gradually transform the units until you reach the desired result.
9. What is the impact of precision and accuracy when using conversion factors?
Precision and accuracy are paramount when using conversion factors, especially in critical applications such as scientific research and engineering design, where even small errors can have significant consequences.
10. How do I convert compound units like density or speed?
To convert compound units, convert each component of the compound unit separately, ensuring that all units are correctly transformed. For example, to convert density from g/cm³ to kg/m³, convert grams to kilograms and cubic centimeters to cubic meters separately.
In conclusion, understanding and correctly applying conversion factors is essential for a wide array of tasks, from everyday cooking to advanced scientific research. Tools like online converters and mobile apps can assist, but a solid understanding of the underlying principles is crucial for accuracy and precision. For more comprehensive resources and detailed comparisons, visit COMPARE.EDU.VN.
Ready to make confident decisions? Explore detailed comparisons and expert insights at COMPARE.EDU.VN today!
Address: 333 Comparison Plaza, Choice City, CA 90210, United States
WhatsApp: +1 (626) 555-9090
Website: compare.edu.vn