Comparing decimals and whole numbers involves determining the relative size of numerical values. COMPARE.EDU.VN simplifies this process by providing clear, comparative analyses. This guide explores the similarities between these comparisons, providing insights and strategies for confident decision-making, enhanced numerical literacy, and practical applications.
1. Understanding the Basics of Number Comparison
Both decimals and whole numbers are fundamental components of the number system, and understanding how to compare them is crucial for various mathematical and real-world applications. The core principle remains the same: assess the magnitude of two or more numbers to determine which is larger or smaller.
1.1 What are Whole Numbers?
Whole numbers are non-negative integers, including zero. They are used for counting discrete quantities. Examples of whole numbers include 0, 1, 5, 42, and 1000. Whole numbers do not include fractions, decimals, or negative numbers.
1.2 What are Decimals?
Decimals are numbers that include a whole number part and a fractional part, separated by a decimal point. The fractional part represents values less than one, expressed in tenths, hundredths, thousandths, and so on. For example, 3.14, 0.75, and 12.005 are decimal numbers.
1.3 The Foundation of Comparison
The basis for comparing both whole numbers and decimals lies in understanding place value. Each digit in a number has a specific value based on its position. In whole numbers, the place values from right to left are ones, tens, hundreds, thousands, and so on. In decimals, the place values to the right of the decimal point are tenths, hundredths, thousandths, and so on. Recognizing these place values allows us to compare numbers accurately.
2. Similarities in the Comparison Process
Despite the presence of a fractional part in decimals, the comparison process shares fundamental similarities with that of whole numbers.
2.1 Starting from the Left
The primary method for comparing both whole numbers and decimals involves starting from the leftmost digit and moving towards the right. This is because the digits on the left have a higher place value and therefore a greater impact on the overall magnitude of the number.
2.2 Comparing Digit by Digit
When comparing numbers, you examine each digit in corresponding place values. If the digits in a particular place value are different, the number with the larger digit in that place value is the greater number. For example, when comparing 456 and 389, you first compare the hundreds place. Since 4 is greater than 3, 456 is greater than 389, regardless of the other digits.
2.3 Importance of Place Value
Understanding place value is crucial for accurate comparisons. It dictates how much each digit contributes to the overall value of the number. For example, in the number 555, each 5 has a different value: the leftmost 5 represents 500, the middle 5 represents 50, and the rightmost 5 represents 5.
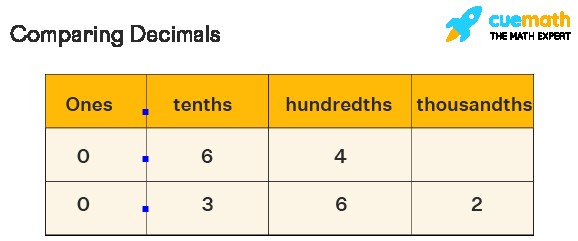
Illustration of place value chart showing comparison of decimals like 0.64 and 0.362
3. Strategies for Comparing Whole Numbers
Comparing whole numbers is straightforward when you understand place value. Here are some strategies to ensure accuracy.
3.1 Counting Digits
The easiest way to start comparing whole numbers is by counting the number of digits. The number with more digits is generally larger. For example, 1,234 is greater than 987 because 1,234 has four digits while 987 has three.
3.2 Aligning Numbers
To accurately compare whole numbers with the same number of digits, align them vertically by their place values. This makes it easier to compare the digits in each place value column.
3.3 Comparing Example
Consider comparing 5,678 and 5,599. Both numbers have four digits, so we start by comparing the thousands place. Both have 5 in the thousands place, so we move to the hundreds place. In this case, 6 is greater than 5, so 5,678 is greater than 5,599.
4. Strategies for Comparing Decimals
Comparing decimals requires a bit more attention to detail, especially when the decimal portions have different lengths.
4.1 Aligning Decimal Points
The first step in comparing decimals is to align the numbers vertically by their decimal points. This ensures that you are comparing digits in the same place value.
4.2 Adding Trailing Zeros
If the decimals have different numbers of digits after the decimal point, you can add trailing zeros to the shorter decimal so that both decimals have the same number of digits. Adding zeros to the end of a decimal does not change its value but makes it easier to compare.
4.3 Comparing Example
Consider comparing 0.75 and 0.755. Align the decimal points and add a trailing zero to 0.75 to make it 0.750. Now you can easily compare 0.750 and 0.755. Both have 7 in the tenths place and 5 in the hundredths place. However, in the thousandths place, 0.750 has 0 while 0.755 has 5. Therefore, 0.755 is greater than 0.75.
5. Key Differences and How to Overcome Them
While the fundamental process of comparing digits from left to right is the same for whole numbers and decimals, some key differences can make comparing decimals more challenging.
5.1 Unequal Number of Digits
One common issue is comparing decimals with different numbers of digits after the decimal point. As mentioned earlier, adding trailing zeros can resolve this issue. For example, when comparing 2.5 and 2.500, adding two trailing zeros to 2.5 makes it clear that 2.5 is equivalent to 2.500.
5.2 Misconceptions about Decimal Length
A common misconception is that a longer decimal is always greater than a shorter one. This is not always the case. For example, 0.9 is greater than 0.899, even though 0.899 has more digits. Always compare the digits in corresponding place values to avoid this mistake.
5.3 Understanding Place Value Differences
It’s essential to understand the place values to the right of the decimal point. The tenths place is more significant than the hundredths place, which is more significant than the thousandths place, and so on. This is why starting the comparison from the tenths place is critical.
6. Visual Aids for Comparison
Visual aids can be extremely helpful in understanding and comparing both whole numbers and decimals.
6.1 Number Lines
Number lines provide a visual representation of numbers and their relative positions. When comparing numbers on a number line, the number to the right is always greater. This works for both whole numbers and decimals.
Example showing decimals 6.5 and 6.7 on a number line
6.2 Place Value Charts
Place value charts are tables that organize numbers by their place values. This can be particularly helpful when comparing decimals, as it ensures that you are comparing digits in the correct place values.
6.3 Bar Models
Bar models are visual representations that use bars to represent numbers. The length of the bar corresponds to the value of the number. This can be useful for comparing fractions and decimals, as it provides a clear visual comparison of their magnitudes.
7. Real-World Applications
Understanding how to compare whole numbers and decimals is essential for many real-world scenarios.
7.1 Financial Transactions
In financial transactions, it’s crucial to compare prices, interest rates, and investment returns. Whether you’re comparing the cost of two items at the store or evaluating different loan options, the ability to compare numbers accurately is essential.
7.2 Measurements
Measurements often involve decimals, whether you’re measuring length, weight, volume, or temperature. Comparing these measurements accurately is crucial in fields like engineering, construction, and healthcare.
7.3 Data Analysis
In data analysis, you often need to compare data points represented as whole numbers and decimals. This could involve comparing sales figures, survey results, or experimental data. Accurate comparisons are essential for drawing meaningful conclusions.
8. Common Mistakes to Avoid
Even with a solid understanding of the principles, it’s easy to make mistakes when comparing numbers. Here are some common pitfalls to avoid.
8.1 Ignoring Place Value
One of the most common mistakes is ignoring place value. Always ensure that you are comparing digits in the same place value.
8.2 Assuming Longer Decimals are Greater
As mentioned earlier, do not assume that a longer decimal is always greater than a shorter one. Always compare digits in corresponding place values.
8.3 Neglecting Trailing Zeros
Forgetting to add trailing zeros when comparing decimals can lead to inaccurate comparisons. Always ensure that both decimals have the same number of digits after the decimal point.
8.4 Overlooking Negative Signs
When comparing negative numbers, remember that the number with the smaller absolute value is actually greater. For example, -2 is greater than -5.
9. Advanced Comparison Techniques
For more complex comparisons, especially when dealing with fractions and percentages, you may need to use advanced techniques.
9.1 Converting Fractions to Decimals
To compare fractions and decimals, convert the fractions to decimals. This makes it easier to compare the numbers directly.
9.2 Using Percentages
Percentages are often used to express proportions. To compare percentages, convert them to decimals or fractions. For example, 25% is equivalent to 0.25 or 1/4.
9.3 Scientific Notation
Scientific notation is used to represent very large or very small numbers. To compare numbers in scientific notation, compare the exponents first. If the exponents are equal, compare the coefficients.
10. Practice Exercises
To solidify your understanding, here are some practice exercises.
10.1 Comparing Whole Numbers
Compare the following pairs of whole numbers:
- 1,234 and 987
- 5,678 and 5,599
- 12,345 and 12,435
- 987,654 and 987,653
- 1,000,000 and 999,999
10.2 Comparing Decimals
Compare the following pairs of decimals:
- 0.75 and 0.755
- 2.5 and 2.500
- 0.9 and 0.899
- 1.234 and 1.243
- 3.14159 and 3.1415
10.3 Comparing Fractions and Decimals
Compare the following fractions and decimals:
- 1/2 and 0.5
- 3/4 and 0.75
- 1/3 and 0.33
- 2/5 and 0.45
- 5/8 and 0.625
11. How COMPARE.EDU.VN Can Help
COMPARE.EDU.VN offers extensive resources to help you master the art of comparing both whole numbers and decimals. Our platform provides detailed comparisons, visual aids, and practice exercises to enhance your understanding.
11.1 Detailed Comparisons
COMPARE.EDU.VN offers in-depth comparisons of various products, services, and ideas. These comparisons include numerical data, such as prices, ratings, and specifications, allowing you to make informed decisions.
11.2 Visual Aids
Our platform incorporates visual aids such as graphs, charts, and tables to help you visualize the differences between numbers. These visual aids can be particularly helpful when comparing decimals, as they provide a clear representation of their magnitudes.
11.3 Practice Exercises
COMPARE.EDU.VN provides a wide range of practice exercises to help you solidify your understanding of number comparison. These exercises cover both whole numbers and decimals and are designed to challenge you at various skill levels.
12. The Role of Technology in Number Comparison
Technology has made comparing numbers easier than ever. Calculators, spreadsheets, and online comparison tools can quickly and accurately compare numbers, saving you time and effort.
12.1 Calculators
Calculators can quickly compare numbers and perform calculations involving decimals. This is particularly useful when dealing with complex financial transactions or scientific measurements.
12.2 Spreadsheets
Spreadsheets such as Microsoft Excel and Google Sheets provide powerful tools for organizing and comparing numerical data. You can use spreadsheets to create tables, charts, and graphs that visualize the differences between numbers.
12.3 Online Comparison Tools
Numerous online comparison tools are available to help you compare products, services, and ideas. These tools often provide detailed comparisons, user reviews, and ratings, allowing you to make informed decisions.
13. Tips for Parents and Educators
Parents and educators can play a crucial role in helping children develop a strong understanding of number comparison. Here are some tips to make learning fun and effective.
13.1 Use Real-World Examples
Use real-world examples to illustrate the importance of number comparison. For example, when shopping with your child, compare the prices of different items to see which is the better deal.
13.2 Play Games
Play games that involve number comparison, such as card games, board games, and online games. These games can make learning fun and engaging.
13.3 Use Visual Aids
Use visual aids such as number lines, place value charts, and bar models to help children visualize the differences between numbers.
13.4 Encourage Practice
Encourage children to practice comparing numbers regularly. The more they practice, the more confident they will become.
14. Conclusion: Mastering the Art of Number Comparison
Comparing decimals and whole numbers is a fundamental skill that is essential for various mathematical and real-world applications. By understanding the basic principles, using effective strategies, and avoiding common mistakes, you can master the art of number comparison.
14.1 The Importance of Accuracy
Accuracy is crucial when comparing numbers. Even a small mistake can lead to significant errors, especially in financial transactions and scientific measurements.
14.2 Continuous Learning
Continuous learning is essential for staying up-to-date with the latest comparison techniques and tools. As technology evolves, new and innovative ways to compare numbers will emerge.
14.3 Leveraging COMPARE.EDU.VN
COMPARE.EDU.VN is your go-to resource for comprehensive comparisons and expert guidance. Whether you’re a student, professional, or everyday consumer, our platform provides the tools and insights you need to make informed decisions.
COMPARE.EDU.VN is dedicated to providing you with the most accurate and comprehensive comparison information. Remember, the ability to compare numbers effectively empowers you to make informed decisions in all aspects of life, leading to better outcomes and greater success. From educational resources to financial decisions, the power of comparison is at your fingertips.
Ready to make smarter choices? Visit COMPARE.EDU.VN today to explore our detailed comparisons and unlock the power of informed decision-making.
- Address: 333 Comparison Plaza, Choice City, CA 90210, United States
- Whatsapp: +1 (626) 555-9090
- Website: compare.edu.vn
15. FAQs About Comparing Decimals and Whole Numbers
15.1 How do you compare a decimal to a whole number?
To compare a decimal to a whole number, treat the whole number as a decimal by adding a decimal point and zeros as needed (e.g., 5 becomes 5.00). Then, compare the numbers as you would compare two decimals, starting from the leftmost digit.
15.2 What is the first step in comparing decimals?
The first step in comparing decimals is to align the numbers vertically by their decimal points. This ensures that you are comparing digits in the same place value.
15.3 How do you add trailing zeros when comparing decimals?
Add trailing zeros to the shorter decimal so that both decimals have the same number of digits after the decimal point. Adding zeros to the end of a decimal does not change its value but makes it easier to compare.
15.4 What is a common mistake to avoid when comparing decimals?
A common mistake to avoid is assuming that a longer decimal is always greater than a shorter one. Always compare the digits in corresponding place values to avoid this mistake.
15.5 How can a number line help in comparing decimals?
When comparing numbers on a number line, the number to the right is always greater. This works for both whole numbers and decimals, providing a visual representation of their relative positions.
15.6 How do you compare negative decimals?
When comparing negative numbers, remember that the number with the smaller absolute value is actually greater. For example, -0.2 is greater than -0.5.
15.7 What is the role of place value in comparing numbers?
Understanding place value is crucial for accurate comparisons. It dictates how much each digit contributes to the overall value of the number, allowing you to compare numbers accurately.
15.8 Can calculators help in comparing numbers?
Yes, calculators can quickly compare numbers and perform calculations involving decimals, saving you time and effort.
15.9 How do you convert fractions to decimals for comparison?
To compare fractions and decimals, convert the fractions to decimals by dividing the numerator by the denominator. This makes it easier to compare the numbers directly.
15.10 What are some real-world applications of comparing decimals and whole numbers?
Real-world applications include financial transactions, measurements, data analysis, and many more scenarios where accurate numerical comparisons are essential.