Comparing numbers in math can seem daunting, but it is a fundamental skill that unlocks many mathematical concepts. At COMPARE.EDU.VN, we provide clear and comprehensive guides on mathematical comparisons and beyond. This article offers an in-depth exploration of comparison techniques in math, covering everything from basic number comparisons to more complex scenarios involving fractions, decimals, and real-world applications. Discover effective strategies for comparing numerical values and enhance your decision-making skills with our insightful analysis.
1. Understanding The Basics Of Mathematical Comparison
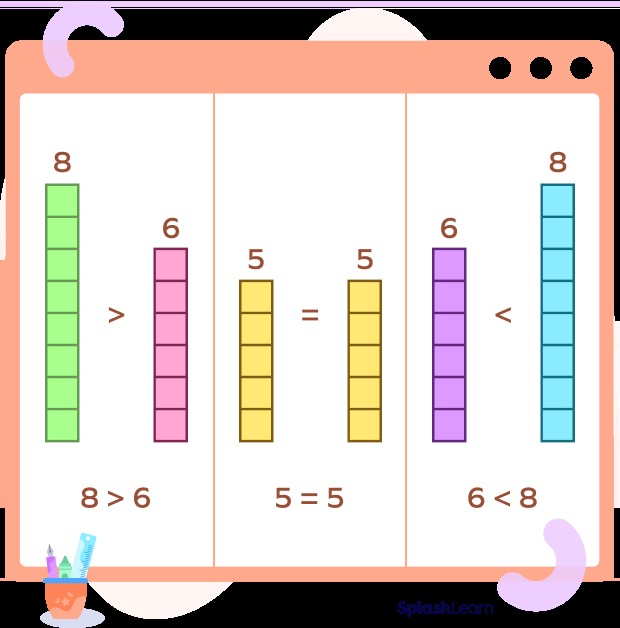
Mathematical comparison is the process of determining the relative size or value of two or more mathematical entities. It involves using specific symbols and methods to establish whether one quantity is greater than, less than, or equal to another. This foundational concept is crucial not only in academic settings but also in everyday decision-making. Mastering the art of comparison allows for informed decisions across various contexts.
1.1 The Symbols of Comparison
The basic tools for expressing mathematical comparisons are the symbols >, <, and =. These symbols help establish relationships between numbers and mathematical expressions. Understanding their usage is essential for performing accurate comparisons.
- Greater Than (>): Indicates that the value on the left is larger than the value on the right. For example, 5 > 3.
- Less Than (<): Indicates that the value on the left is smaller than the value on the right. For example, 2 < 7.
- Equal To (=): Indicates that the values on both sides are the same. For example, 4 = 4.
Using these symbols allows for a concise and clear expression of mathematical relationships.
1.2 The Number Line as a Comparison Tool
The number line is a visual aid that simplifies number comparison. Numbers increase in value as you move from left to right on the number line. This makes it easy to compare integers, fractions, and decimals.
- Positive Numbers: Located to the right of zero, they increase in value as you move further from zero.
- Negative Numbers: Located to the left of zero, they decrease in value as you move further from zero.
Using a number line, one can easily see that 5 > -3 because 5 is located to the right of -3 on the number line. This method is particularly useful for visualizing the relationship between negative and positive numbers.
1.3 Basic Properties of Inequalities
Understanding the fundamental properties of inequalities is crucial for solving more complex comparison problems. These properties ensure that mathematical relationships remain valid under certain operations.
- Addition Property: Adding the same number to both sides of an inequality preserves the inequality. If a > b, then a + c > b + c.
- Subtraction Property: Subtracting the same number from both sides of an inequality preserves the inequality. If a > b, then a – c > b – c.
- Multiplication Property: Multiplying both sides of an inequality by a positive number preserves the inequality. If a > b and c > 0, then ac > bc.
- Division Property: Dividing both sides of an inequality by a positive number preserves the inequality. If a > b and c > 0, then a/c > b/c.
- Reversal Property: If a > b, then b < a. This property is useful for rewriting inequalities to suit specific problem-solving needs.
These properties form the bedrock of inequality manipulation and are essential for solving a variety of mathematical problems.
2. Comparing Whole Numbers: Strategies and Techniques
Comparing whole numbers is a foundational skill in mathematics. It involves determining which of two or more whole numbers is larger, smaller, or if they are equal. This process is essential for a variety of mathematical operations and real-world applications.
2.1 Digit Counting Method
The digit counting method is a straightforward way to compare whole numbers. The number with more digits is generally larger. This method is particularly useful when the numbers have significantly different magnitudes.
- Example: Compare 123 and 1234.
- 123 has three digits.
- 1234 has four digits.
- Therefore, 1234 > 123.
2.2 Place Value Comparison
When whole numbers have the same number of digits, comparing place values is the most effective method. Start from the leftmost digit (highest place value) and move to the right until a difference is found.
- Example: Compare 5678 and 5692.
- Both numbers have four digits.
- The thousands place is the same (5).
- The hundreds place is the same (6).
- The tens place differs: 7 in 5678 and 9 in 5692.
- Since 9 > 7, 5692 > 5678.
2.3 Using the Number Line for Whole Numbers
The number line provides a visual tool for comparing whole numbers. Numbers to the right are larger, while numbers to the left are smaller. This method is particularly helpful for understanding the order and magnitude of numbers.
- Example: Compare 3 and 7.
- On a number line, 7 is to the right of 3.
- Therefore, 7 > 3.
2.4 Real-life Examples of Whole Number Comparisons
Whole number comparisons are common in everyday scenarios. Consider these examples:
- Budgeting: Comparing income and expenses to determine if there is a surplus or deficit.
- Shopping: Comparing the prices of different items to find the best deal.
- Time Management: Comparing the duration of tasks to schedule activities effectively.
- Sports: Comparing scores or statistics to determine the winner or evaluate performance.
Understanding how to compare whole numbers efficiently enhances decision-making skills in these practical situations.
3. Comparing Integers: Dealing with Negative Numbers
Comparing integers involves considering both positive and negative whole numbers. Understanding the properties of negative numbers is essential for accurate comparisons.
3.1 Positive vs. Negative Integers
Any positive integer is always greater than any negative integer. Zero is greater than any negative integer but less than any positive integer.
- Example: Compare 5 and -3.
- 5 is a positive integer.
- -3 is a negative integer.
- Therefore, 5 > -3.
3.2 Comparing Two Positive Integers
Comparing two positive integers is the same as comparing whole numbers. Use the digit counting or place value methods discussed earlier.
- Example: Compare 15 and 20.
- Both are positive integers.
- 20 > 15.
3.3 Comparing Two Negative Integers
When comparing two negative integers, the integer with the smaller absolute value is larger. This is because it is closer to zero on the number line.
- Example: Compare -8 and -5.
- |-8| = 8 and |-5| = 5.
- Since 5 < 8, -5 > -8.
3.4 The Number Line and Integers
Using the number line, negative integers to the right are larger than those to the left. This visual aid helps in understanding the relative magnitudes of negative numbers.
- Example: Compare -2 and -6.
- On a number line, -2 is to the right of -6.
- Therefore, -2 > -6.
3.5 Real-life Examples of Integer Comparisons
Integer comparisons are frequently used in various real-life contexts:
- Temperature: Comparing temperatures above and below zero.
- Finance: Comparing gains and losses in investments.
- Elevation: Comparing altitudes above and below sea level.
- Sports: Comparing scores where negative values may represent penalties or points deducted.
4. Comparing Fractions: Making Sense of Parts of a Whole
Comparing fractions involves determining the relative size of two or more fractional values. Different methods are used depending on whether the fractions have the same or different denominators.
4.1 Like Fractions: Same Denominator
When fractions have the same denominator (like fractions), comparing them is straightforward: compare the numerators. The fraction with the larger numerator is the larger fraction.
- Example: Compare 3/7 and 5/7.
- Both fractions have the same denominator (7).
- Compare the numerators: 3 and 5.
- Since 5 > 3, 5/7 > 3/7.
4.2 Unlike Fractions: Different Denominators
Comparing fractions with different denominators (unlike fractions) requires additional steps to make the comparison accurate.
Method 1: Finding a Common Denominator
Convert the fractions to equivalent fractions with a common denominator. The least common denominator (LCD) is often used for simplicity.
- Example: Compare 1/3 and 2/5.
- Find the LCD of 3 and 5, which is 15.
- Convert 1/3 to 5/15 (multiply numerator and denominator by 5).
- Convert 2/5 to 6/15 (multiply numerator and denominator by 3).
- Now compare 5/15 and 6/15.
- Since 6 > 5, 6/15 > 5/15, so 2/5 > 1/3.
Method 2: Cross Multiplication
Multiply the numerator of the first fraction by the denominator of the second fraction, and vice versa. Compare the results.
- Example: Compare 3/4 and 5/7.
- Multiply 3 (numerator of the first fraction) by 7 (denominator of the second fraction): 3 * 7 = 21.
- Multiply 5 (numerator of the second fraction) by 4 (denominator of the first fraction): 5 * 4 = 20.
- Compare the results: 21 and 20.
- Since 21 > 20, 3/4 > 5/7.
Method 3: Converting to Decimals
Convert each fraction to a decimal and then compare the decimal values.
- Example: Compare 1/4 and 2/5.
- Convert 1/4 to a decimal: 1 ÷ 4 = 0.25.
- Convert 2/5 to a decimal: 2 ÷ 5 = 0.4.
- Compare the decimals: 0.25 and 0.4.
- Since 0.4 > 0.25, 2/5 > 1/4.
4.3 Using Benchmarks for Fraction Comparison
Benchmarks like 0, 1/2, and 1 can simplify fraction comparisons. Determine if a fraction is closer to 0, 1/2, or 1, and use this information to compare.
- Example: Compare 3/8 and 5/9.
- 3/8 is slightly less than 1/2 (1/2 = 4/8).
- 5/9 is slightly more than 1/2 (1/2 = 4.5/9).
- Therefore, 5/9 > 3/8.
4.4 Real-life Examples of Fraction Comparisons
Fraction comparisons are useful in many real-world scenarios:
- Cooking: Determining which recipe uses a larger portion of an ingredient.
- Construction: Comparing lengths or areas represented as fractions.
- Finance: Comparing fractions of investments or debts.
- Education: Comparing test scores represented as fractions.
5. Comparing Decimals: Precision in Measurement
Comparing decimals is crucial for precision in various fields, from science to finance. Understanding how to compare decimal values accurately is essential for many applications.
5.1 Aligning Decimal Points
The first step in comparing decimals is to align the decimal points vertically. This ensures that you are comparing digits in the same place value.
- Example: Compare 3.14 and 3.1415.
3. 14
3. 14155.2 Adding Trailing Zeros
Add trailing zeros to the decimal with fewer digits after the decimal point. This makes the comparison easier without changing the value of the decimal.
- Example: Compare 3.14 and 3.1415.
3. 1400
3. 14155.3 Comparing Place Values
Start comparing the digits from left to right, beginning with the ones place. Continue until you find a difference in place values.
- Example: Compare 3.1400 and 3.1415.
- The ones place is the same (3).
- The tenths place is the same (1).
- The hundredths place is the same (4).
- The thousandths place differs: 0 in 3.1400 and 1 in 3.1415.
- Since 1 > 0, 3.1415 > 3.1400.
5.4 Using the Number Line for Decimals
The number line can also be used for decimal comparisons. Decimals to the right are larger, while those to the left are smaller.
- Example: Compare 0.5 and 0.75.
- On a number line, 0.75 is to the right of 0.5.
- Therefore, 0.75 > 0.5.
5.5 Real-life Examples of Decimal Comparisons
Decimal comparisons are common in many real-world applications:
- Measurement: Comparing lengths, weights, or volumes with decimal precision.
- Finance: Comparing interest rates or monetary values.
- Science: Comparing experimental results or physical constants.
- Engineering: Comparing dimensions or tolerances in designs.
6. Comparing Rational Numbers: Combining Integers and Fractions
Rational numbers encompass both integers and fractions, including positive and negative values. Comparing rational numbers requires combining the techniques used for integers and fractions.
6.1 Converting to a Common Form
Convert all rational numbers to a common form, either fractions or decimals, to facilitate comparison. This ensures that all numbers are in a comparable format.
- Example: Compare -1/2 and -0.75.
- Convert -1/2 to a decimal: -0.5.
- Now compare -0.5 and -0.75.
6.2 Applying Integer Comparison Rules
Use the rules for comparing integers when dealing with rational numbers that include negative values. Remember that negative numbers closer to zero are larger.
- Example: Compare -0.5 and -0.75.
- Since -0.5 is to the right of -0.75 on the number line, -0.5 > -0.75.
- Therefore, -1/2 > -0.75.
6.3 Applying Fraction Comparison Rules
Use the methods for comparing fractions (common denominators, cross multiplication, benchmarks) when dealing with rational numbers in fractional form.
- Example: Compare 2/3 and 3/4.
- Using common denominators, convert 2/3 to 8/12 and 3/4 to 9/12.
- Since 9/12 > 8/12, 3/4 > 2/3.
6.4 Real-life Examples of Rational Number Comparisons
Rational number comparisons are essential in various real-world contexts:
- Finance: Comparing stock prices, interest rates, or investment returns.
- Science: Comparing measurements in experiments, such as temperature changes or concentrations.
- Engineering: Comparing tolerances in manufacturing or design specifications.
- Economics: Comparing economic indicators like GDP growth rates or inflation rates.
7. Advanced Comparison Techniques: Beyond the Basics
While the basic comparison methods are foundational, some situations require more advanced techniques. These techniques often involve manipulating expressions or using specific properties to simplify the comparison process.
7.1 Using Properties of Inequalities
Apply the properties of inequalities (addition, subtraction, multiplication, division) to simplify complex comparisons. This can involve isolating variables or transforming expressions to make the comparison clearer.
- Example: Compare a + 5 and b + 5, given that a > b.
- Since a > b, adding 5 to both sides preserves the inequality.
- Therefore, a + 5 > b + 5.
7.2 Comparing Absolute Values
When comparing expressions involving absolute values, consider different cases based on the sign of the expressions inside the absolute value.
- Example: Compare |x| and |y|, given that x = -3 and y = 2.
- |x| = |-3| = 3.
- |y| = |2| = 2.
- Therefore, |x| > |y|.
7.3 Comparing Algebraic Expressions
To compare algebraic expressions, simplify them as much as possible and then compare the coefficients and constants.
- Example: Compare 3x + 2 and 2x + 5, given that x > 1.
- Since x > 1, 3x > 3 and 2x > 2.
- 3x + 2 > 3 + 2 = 5.
- 2x + 5 > 2 + 5 = 7.
- However, without a specific value for x, it’s difficult to definitively say which expression is larger for all x > 1. Further analysis or specific values might be needed.
7.4 Using Functions for Comparison
Functions can be used to model relationships and make comparisons. Analyzing the behavior of functions, such as whether they are increasing or decreasing, can help in comparing their values.
- Example: Compare f(x) = x^2 and g(x) = x, given that x > 1.
- For x > 1, x^2 increases faster than x.
- Therefore, f(x) > g(x) for x > 1.
8. Real-World Applications: Making Informed Decisions
Mathematical comparison is not just an academic exercise; it is a critical skill for making informed decisions in various aspects of life.
8.1 Personal Finance
In personal finance, comparison is used to evaluate different investment options, loan terms, and budgeting choices.
- Example: Comparing interest rates on loans.
- Loan A: 5.25% interest rate.
- Loan B: 5.5% interest rate.
- Since 5.25% < 5.5%, Loan A is the better option.
8.2 Shopping and Consumer Decisions
Comparison helps consumers make informed choices by evaluating prices, features, and quality of different products.
- Example: Comparing prices of two similar products.
- Product X: $25.99.
- Product Y: $24.75.
- Since $24.75 < $25.99, Product Y is the more economical choice.
8.3 Health and Fitness
Comparison is used to track progress, evaluate different workout routines, and make informed dietary choices.
- Example: Comparing calorie counts of different foods.
- Food A: 250 calories per serving.
- Food B: 300 calories per serving.
- If aiming to reduce calorie intake, Food A is the better option.
8.4 Travel and Logistics
Comparison is essential for planning trips, evaluating transportation options, and optimizing routes.
- Example: Comparing travel times for different routes.
- Route 1: 2 hours 30 minutes.
- Route 2: 2 hours 15 minutes.
- Since 2 hours 15 minutes < 2 hours 30 minutes, Route 2 is the faster option.
8.5 Professional and Academic Settings
In professional and academic settings, comparison is used to analyze data, evaluate performance, and make strategic decisions.
- Example: Comparing sales figures for two different quarters.
- Quarter 1: $150,000 in sales.
- Quarter 2: $165,000 in sales.
- Since $165,000 > $150,000, sales increased in Quarter 2.
9. Practice Problems: Sharpening Your Comparison Skills
To reinforce your understanding of mathematical comparison, try solving these practice problems:
- Compare 456 and 465.
- Compare -12 and -15.
- Compare 2/5 and 3/8.
- Compare 0.65 and 0.625.
- Compare -3/4 and -0.8.
- Compare a + 3 and b + 3, given that a < b.
- Compare |x| and |y|, given that x = -5 and y = 3.
- Compare the areas of two rectangles: Rectangle A (length = 8, width = 5) and Rectangle B (length = 7, width = 6).
- Compare the volumes of two cubes: Cube C (side = 4) and Cube D (side = 3).
- Compare the perimeters of two squares: Square E (side = 6) and Square F (side = 7).
10. Frequently Asked Questions (FAQ)
Q1: Why is it important to learn how to compare numbers in math?
Comparing numbers is fundamental for making informed decisions in everyday life, from personal finance to shopping. It also lays the groundwork for more complex mathematical concepts.
Q2: What is the easiest way to compare two fractions?
One of the easiest ways is to cross multiply. Multiply the numerator of the first fraction by the denominator of the second fraction, and vice versa, then compare the results.
Q3: How do you compare two negative numbers?
When comparing two negative numbers, the number with the smaller absolute value is larger because it is closer to zero on the number line.
Q4: Can you use a number line to compare decimals?
Yes, the number line is a useful visual aid for comparing decimals. Decimals to the right are larger, while those to the left are smaller.
Q5: What should I do if I need to compare a fraction and a decimal?
Convert both numbers to a common format, either fractions or decimals, and then compare them using the appropriate methods.
Q6: How can properties of inequalities help in comparing expressions?
Properties of inequalities (addition, subtraction, multiplication, division) can simplify complex comparisons by isolating variables or transforming expressions.
Q7: What is the role of place value in comparing whole numbers?
Place value is crucial when comparing whole numbers with the same number of digits. Start from the leftmost digit and move to the right until a difference is found.
Q8: How do you compare algebraic expressions?
Simplify the expressions as much as possible and then compare the coefficients and constants. In some cases, you might need to know the value or range of values for the variable.
Q9: In real life, where do we use comparisons the most?
Comparisons are used extensively in personal finance (evaluating loans and investments), shopping (finding the best deals), health and fitness (tracking progress), and travel (optimizing routes).
Q10: What is the significance of benchmarks like 0, 1/2, and 1 when comparing fractions?
Benchmarks simplify fraction comparisons by helping you quickly estimate whether a fraction is closer to 0, 1/2, or 1, making it easier to compare their relative sizes.
Are you still struggling with comparisons? Visit COMPARE.EDU.VN for more detailed guides, practical examples, and interactive tools that make mathematical comparisons easy and accessible. Our resources provide you with the knowledge and confidence to make informed decisions in all areas of life.
Ready to make smarter comparisons? Visit compare.edu.vn today to explore our comprehensive resources and unlock your potential for informed decision-making. Contact us at 333 Comparison Plaza, Choice City, CA 90210, United States. Whatsapp: +1 (626) 555-9090. We’re here to help you compare and choose wisely